Question and Answers Forum

All Questions      Topic List

Integration Questions

Previous in All Question      Next in All Question      

Previous in Integration      Next in Integration      

Question Number 182367 by universe last updated on 08/Dec/22

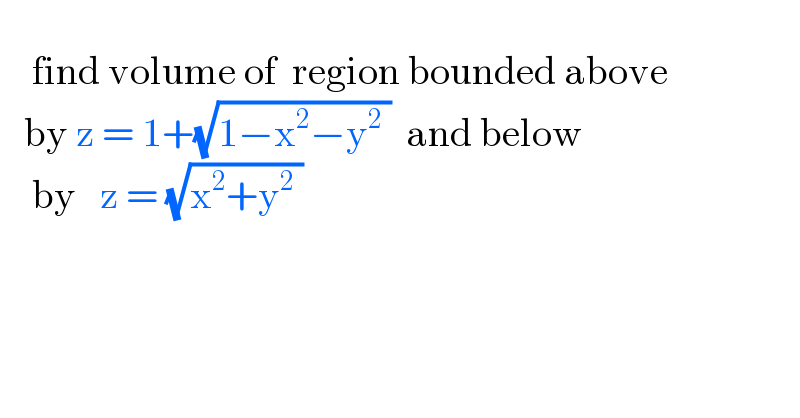
find volume of region bounded above by z = 1+(√(1−x^2 −y^2 )) and below by z = (√(x^2 +y^2 ))

$$ \\ $$$$\:\:\:\:\mathrm{find}\:\mathrm{volume}\:\mathrm{of}\:\:\mathrm{region}\:\mathrm{bounded}\:\mathrm{above}\: \\ $$$$\:\:\:\mathrm{by}\:\mathrm{z}\:=\:\mathrm{1}+\sqrt{\mathrm{1}−\mathrm{x}^{\mathrm{2}} −\mathrm{y}^{\mathrm{2}} \:}\:\:\mathrm{and}\:\mathrm{below} \\ $$$$\:\:\:\:\mathrm{by}\:\:\:\mathrm{z}\:=\:\sqrt{\mathrm{x}^{\mathrm{2}} +\mathrm{y}^{\mathrm{2}} \:}\: \\ $$

Answered by Tokugami last updated on 08/Dec/22

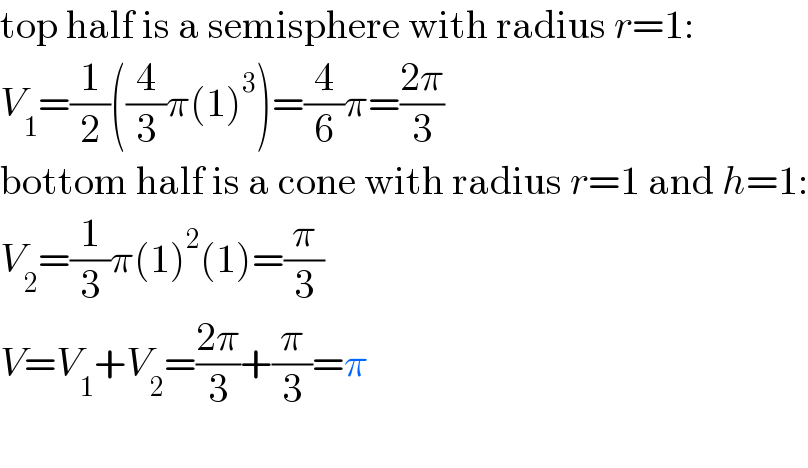
top half is a semisphere with radius r=1: V_1 =(1/2)((4/3)π(1)^3 )=(4/6)π=((2π)/3) bottom half is a cone with radius r=1 and h=1: V_2 =(1/3)π(1)^2 (1)=(π/3) V=V_1 +V_2 =((2π)/3)+(π/3)=π

$$\mathrm{top}\:\mathrm{half}\:\mathrm{is}\:\mathrm{a}\:\mathrm{semisphere}\:\mathrm{with}\:\mathrm{radius}\:{r}=\mathrm{1}: \\ $$$${V}_{\mathrm{1}} =\frac{\mathrm{1}}{\mathrm{2}}\left(\frac{\mathrm{4}}{\mathrm{3}}\pi\left(\mathrm{1}\right)^{\mathrm{3}} \right)=\frac{\mathrm{4}}{\mathrm{6}}\pi=\frac{\mathrm{2}\pi}{\mathrm{3}} \\ $$$$\mathrm{bottom}\:\mathrm{half}\:\mathrm{is}\:\mathrm{a}\:\mathrm{cone}\:\mathrm{with}\:\mathrm{radius}\:{r}=\mathrm{1}\:\mathrm{and}\:{h}=\mathrm{1}: \\ $$$${V}_{\mathrm{2}} =\frac{\mathrm{1}}{\mathrm{3}}\pi\left(\mathrm{1}\right)^{\mathrm{2}} \left(\mathrm{1}\right)=\frac{\pi}{\mathrm{3}} \\ $$$${V}={V}_{\mathrm{1}} +{V}_{\mathrm{2}} =\frac{\mathrm{2}\pi}{\mathrm{3}}+\frac{\pi}{\mathrm{3}}=\pi \\ $$$$ \\ $$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com