Question and Answers Forum

All Questions      Topic List

Others Questions

Previous in All Question      Next in All Question      

Previous in Others      Next in Others      

Question Number 87751 by Rio Michael last updated on 06/Apr/20

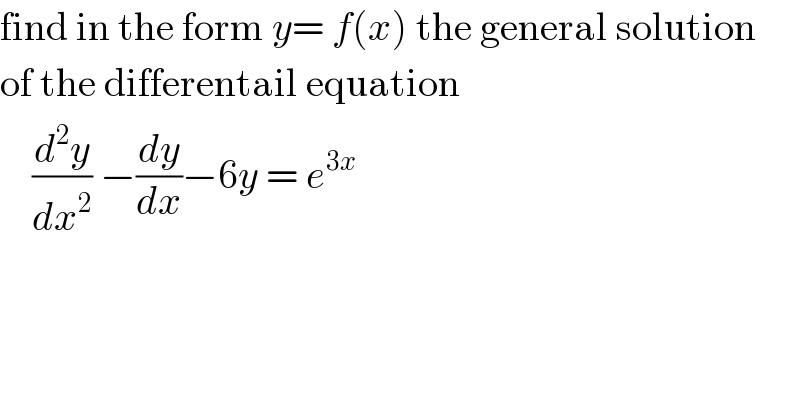
find in the form y= f(x) the general solution of the differentail equation (d^2 y/dx^2 ) −(dy/dx)−6y = e^(3x)

$$\mathrm{find}\:\mathrm{in}\:\mathrm{the}\:\mathrm{form}\:{y}=\:{f}\left({x}\right)\:\mathrm{the}\:\mathrm{general}\:\mathrm{solution}\: \\ $$$$\mathrm{of}\:\mathrm{the}\:\mathrm{differentail}\:\mathrm{equation} \\ $$$$\:\:\:\:\frac{{d}^{\mathrm{2}} {y}}{{dx}^{\mathrm{2}} }\:−\frac{{dy}}{{dx}}−\mathrm{6}{y}\:=\:{e}^{\mathrm{3}{x}} \\ $$$$ \\ $$

Commented by niroj last updated on 06/Apr/20

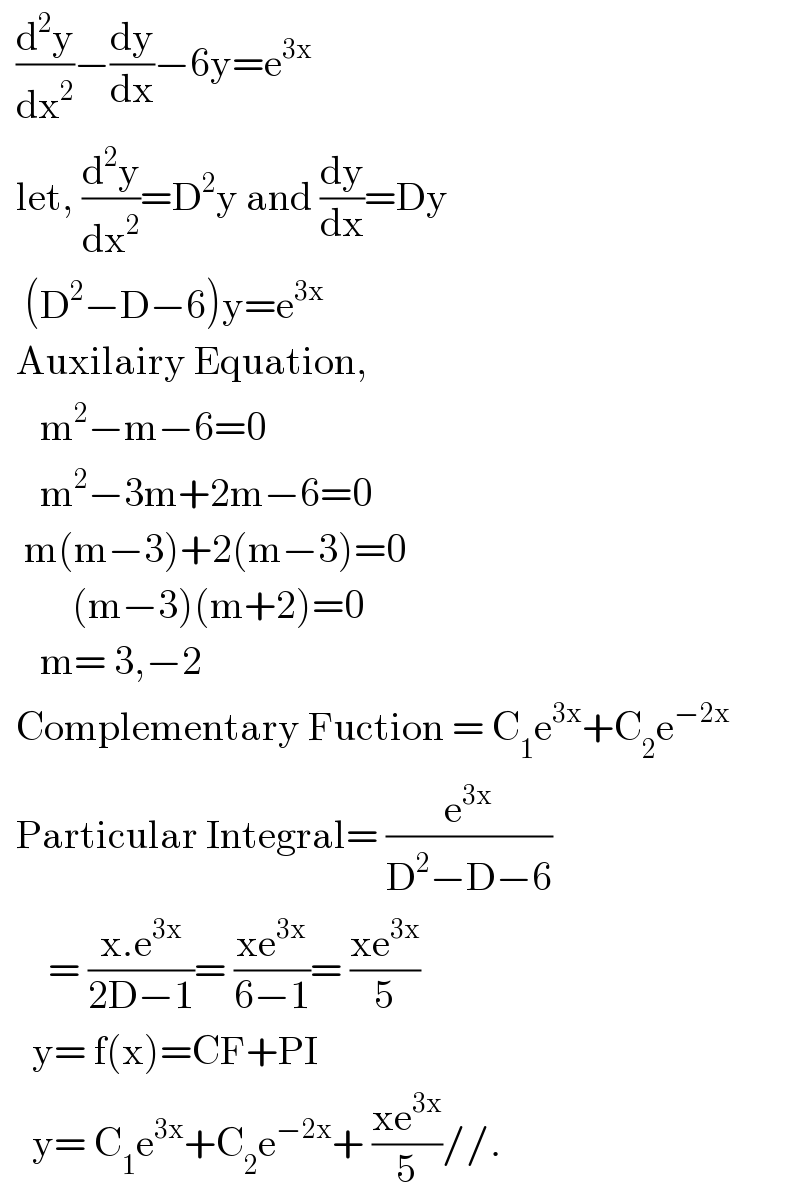
(d^2 y/dx^2 )−(dy/dx)−6y=e^(3x) let, (d^2 y/dx^2 )=D^2 y and (dy/dx)=Dy (D^2 −D−6)y=e^(3x) Auxilairy Equation, m^2 −m−6=0 m^2 −3m+2m−6=0 m(m−3)+2(m−3)=0 (m−3)(m+2)=0 m= 3,−2 Complementary Fuction = C_1 e^(3x) +C_2 e^(−2x) Particular Integral= (e^(3x) /(D^2 −D−6)) = ((x.e^(3x) )/(2D−1))= ((xe^(3x) )/(6−1))= ((xe^(3x) )/5) y= f(x)=CF+PI y= C_1 e^(3x) +C_2 e^(−2x) + ((xe^(3x) )/5)//.

$$\:\:\frac{\mathrm{d}^{\mathrm{2}} \mathrm{y}}{\mathrm{dx}^{\mathrm{2}} }−\frac{\mathrm{dy}}{\mathrm{dx}}−\mathrm{6y}=\mathrm{e}^{\mathrm{3x}} \\ $$$$\:\:\mathrm{let},\:\frac{\mathrm{d}^{\mathrm{2}} \mathrm{y}}{\mathrm{dx}^{\mathrm{2}} }=\mathrm{D}^{\mathrm{2}} \mathrm{y}\:\mathrm{and}\:\frac{\mathrm{dy}}{\mathrm{dx}}=\mathrm{Dy} \\ $$$$\:\:\:\left(\mathrm{D}^{\mathrm{2}} −\mathrm{D}−\mathrm{6}\right)\mathrm{y}=\mathrm{e}^{\mathrm{3x}} \\ $$$$\:\:\mathrm{Auxilairy}\:\mathrm{Equation}, \\ $$$$\:\:\:\:\:\mathrm{m}^{\mathrm{2}} −\mathrm{m}−\mathrm{6}=\mathrm{0} \\ $$$$\:\:\:\:\:\mathrm{m}^{\mathrm{2}} −\mathrm{3m}+\mathrm{2m}−\mathrm{6}=\mathrm{0} \\ $$$$\:\:\:\mathrm{m}\left(\mathrm{m}−\mathrm{3}\right)+\mathrm{2}\left(\mathrm{m}−\mathrm{3}\right)=\mathrm{0} \\ $$$$\:\:\:\:\:\:\:\:\:\left(\mathrm{m}−\mathrm{3}\right)\left(\mathrm{m}+\mathrm{2}\right)=\mathrm{0} \\ $$$$\:\:\:\:\:\mathrm{m}=\:\mathrm{3},−\mathrm{2} \\ $$$$\:\:\mathrm{Complementary}\:\mathrm{Fuction}\:=\:\mathrm{C}_{\mathrm{1}} \mathrm{e}^{\mathrm{3x}} +\mathrm{C}_{\mathrm{2}} \mathrm{e}^{−\mathrm{2x}} \\ $$$$\:\:\mathrm{Particular}\:\mathrm{Integral}=\:\frac{\mathrm{e}^{\mathrm{3x}} }{\mathrm{D}^{\mathrm{2}} −\mathrm{D}−\mathrm{6}} \\ $$$$\:\:\:\:\:\:=\:\frac{\mathrm{x}.\mathrm{e}^{\mathrm{3x}} }{\mathrm{2D}−\mathrm{1}}=\:\frac{\mathrm{xe}^{\mathrm{3x}} }{\mathrm{6}−\mathrm{1}}=\:\frac{\mathrm{xe}^{\mathrm{3x}} }{\mathrm{5}} \\ $$$$\:\:\:\:\mathrm{y}=\:\mathrm{f}\left(\mathrm{x}\right)=\mathrm{CF}+\mathrm{PI} \\ $$$$\:\:\:\:\mathrm{y}=\:\mathrm{C}_{\mathrm{1}} \mathrm{e}^{\mathrm{3x}} +\mathrm{C}_{\mathrm{2}} \mathrm{e}^{−\mathrm{2x}} +\:\frac{\mathrm{xe}^{\mathrm{3x}} }{\mathrm{5}}//. \\ $$

Commented by Rio Michael last updated on 06/Apr/20

thanks sir

$$\mathrm{thanks}\:\mathrm{sir} \\ $$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com