Question and Answers Forum

All Questions      Topic List

Differentiation Questions

Previous in All Question      Next in All Question      

Previous in Differentiation      Next in Differentiation      

Question Number 63301 by Rio Michael last updated on 02/Jul/19

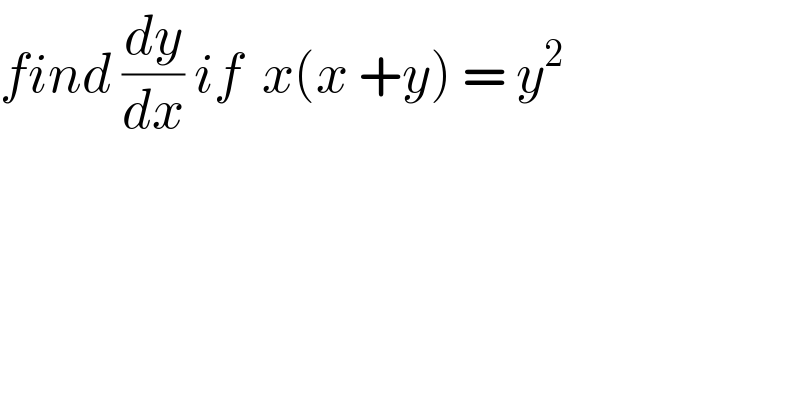
find (dy/dx) if x(x +y) = y^2

$${find}\:\frac{{dy}}{{dx}}\:{if}\:\:{x}\left({x}\:+{y}\right)\:=\:{y}^{\mathrm{2}} \\ $$

Commented by kaivan.ahmadi last updated on 02/Jul/19

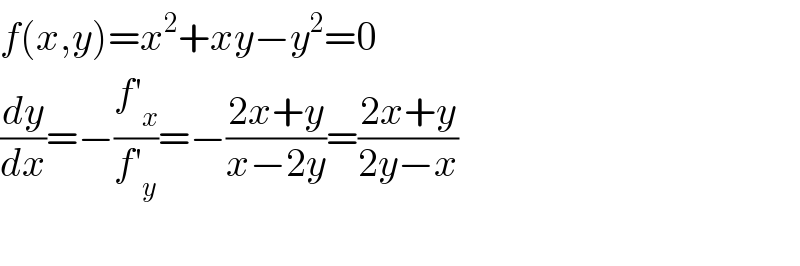
f(x,y)=x^2 +xy−y^2 =0 (dy/dx)=−((f′_x )/(f′_y ))=−((2x+y)/(x−2y))=((2x+y)/(2y−x))

$${f}\left({x},{y}\right)={x}^{\mathrm{2}} +{xy}−{y}^{\mathrm{2}} =\mathrm{0} \\ $$$$\frac{{dy}}{{dx}}=−\frac{{f}'_{{x}} }{{f}'_{{y}} }=−\frac{\mathrm{2}{x}+{y}}{{x}−\mathrm{2}{y}}=\frac{\mathrm{2}{x}+{y}}{\mathrm{2}{y}−{x}} \\ $$$$ \\ $$

Commented by Rio Michael last updated on 02/Jul/19

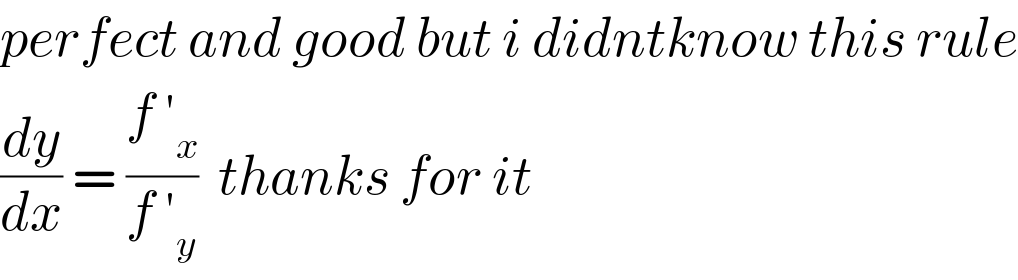
perfect and good but i didntknow this rule (dy/dx) = ((f ′_x )/(f ′_y )) thanks for it

$${perfect}\:{and}\:{good}\:{but}\:{i}\:{didntknow}\:{this}\:{rule} \\ $$$$\frac{{dy}}{{dx}}\:=\:\frac{{f}\:'_{{x}} }{{f}\:'_{{y}} }\:\:{thanks}\:{for}\:{it} \\ $$

Commented by Prithwish sen last updated on 02/Jul/19

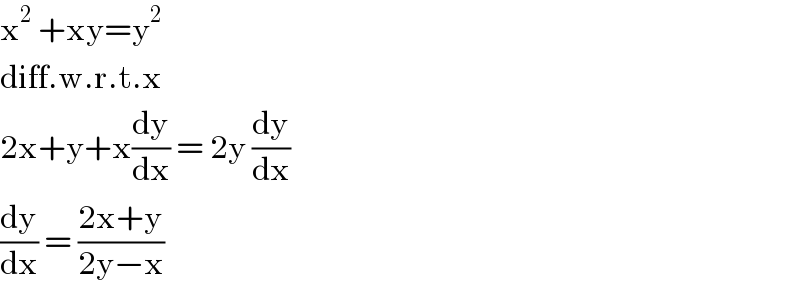
x^2 +xy=y^2 diff.w.r.t.x 2x+y+x(dy/dx) = 2y (dy/dx) (dy/dx) = ((2x+y)/(2y−x))

$$\mathrm{x}^{\mathrm{2}} \:+\mathrm{xy}=\mathrm{y}^{\mathrm{2}} \\ $$$$\mathrm{diff}.\mathrm{w}.\mathrm{r}.\mathrm{t}.\mathrm{x} \\ $$$$\mathrm{2x}+\mathrm{y}+\mathrm{x}\frac{\mathrm{dy}}{\mathrm{dx}}\:=\:\mathrm{2y}\:\frac{\mathrm{dy}}{\mathrm{dx}} \\ $$$$\frac{\mathrm{dy}}{\mathrm{dx}}\:=\:\frac{\mathrm{2x}+\mathrm{y}}{\mathrm{2y}−\mathrm{x}} \\ $$

Commented by Rio Michael last updated on 02/Jul/19

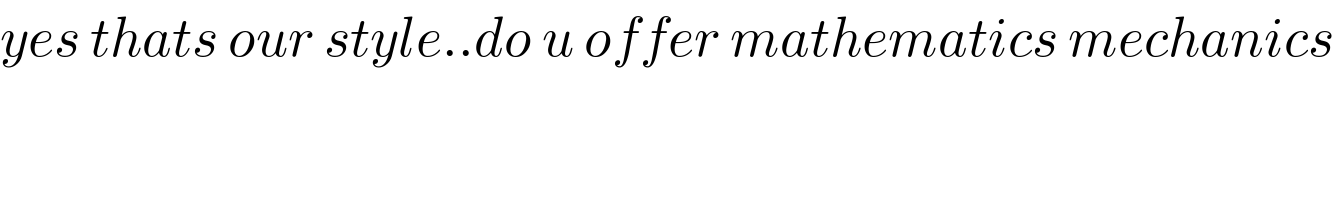
yes thats our style..do u offer mathematics mechanics

$${yes}\:{thats}\:{our}\:{style}..{do}\:{u}\:{offer}\:{mathematics}\:{mechanics} \\ $$

Commented by MJS last updated on 02/Jul/19

the “long version” x^2 +xy−y^2 =0 now differentiate each term and multiply with dx or dy: x^2 → 2xdx xy → ydx+xdy y^2 → 2ydy 2xdx+ydx+xdy−2ydy=0 now “split” dx from dy (2x+y)dx=(2y−x)dy ⇒ (dy/dx)=((2x+y)/(2y−x))

$$\mathrm{the}\:``\mathrm{long}\:\mathrm{version}'' \\ $$$${x}^{\mathrm{2}} +{xy}−{y}^{\mathrm{2}} =\mathrm{0} \\ $$$$\mathrm{now}\:\mathrm{differentiate}\:\mathrm{each}\:\mathrm{term}\:\mathrm{and}\:\mathrm{multiply}\:\mathrm{with} \\ $$$${dx}\:\mathrm{or}\:{dy}: \\ $$$${x}^{\mathrm{2}} \:\rightarrow\:\mathrm{2}{xdx} \\ $$$${xy}\:\rightarrow\:{ydx}+{xdy} \\ $$$${y}^{\mathrm{2}} \:\rightarrow\:\mathrm{2}{ydy} \\ $$$$\mathrm{2}{xdx}+{ydx}+{xdy}−\mathrm{2}{ydy}=\mathrm{0} \\ $$$$\mathrm{now}\:``\mathrm{split}''\:{dx}\:\mathrm{from}\:{dy} \\ $$$$\left(\mathrm{2}{x}+{y}\right){dx}=\left(\mathrm{2}{y}−{x}\right){dy} \\ $$$$\Rightarrow\:\frac{{dy}}{{dx}}=\frac{\mathrm{2}{x}+{y}}{\mathrm{2}{y}−{x}} \\ $$

Commented by Rio Michael last updated on 02/Jul/19

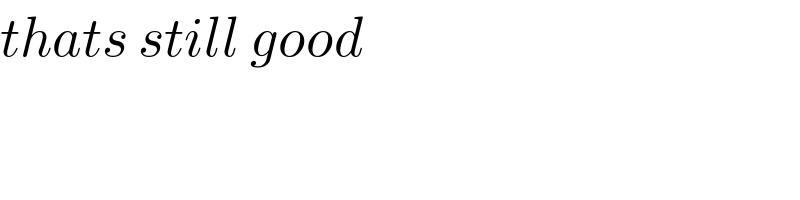
thats still good

$${thats}\:{still}\:{good} \\ $$

Commented by mathmax by abdo last updated on 02/Jul/19

(e) ⇔y^2 −xy −x^2 =0 ⇒p(y)=0 Δ =x^2 +4x^2 =5x^2 ⇒y =(1/2)(x+(√5)xξ(x)) or y =(1/2)(x−(√5)ξ(x)x ) with ξ(x)=1 if x>0 and ξ(x)=−1 if x<0 ⇒y =(x/2)(1+(√5)ξ(x)) or y =(x/2)(1−(√5)ξ(x)) ⇒ (dy/dx) =((1+(√5)ξ(x))/2) or (dy/dx) =((1−(√5)ξ(x))/2)

$$\left({e}\right)\:\Leftrightarrow{y}^{\mathrm{2}} −{xy}\:−{x}^{\mathrm{2}} \:=\mathrm{0}\:\Rightarrow{p}\left({y}\right)=\mathrm{0}\: \\ $$$$\Delta\:={x}^{\mathrm{2}} \:+\mathrm{4}{x}^{\mathrm{2}} \:=\mathrm{5}{x}^{\mathrm{2}} \:\Rightarrow{y}\:=\frac{\mathrm{1}}{\mathrm{2}}\left({x}+\sqrt{\mathrm{5}}{x}\xi\left({x}\right)\right)\:\:{or}\:{y}\:=\frac{\mathrm{1}}{\mathrm{2}}\left({x}−\sqrt{\mathrm{5}}\xi\left({x}\right){x}\:\right)\:\:{with}\:\xi\left({x}\right)=\mathrm{1}\:{if}\:{x}>\mathrm{0} \\ $$$${and}\:\xi\left({x}\right)=−\mathrm{1}\:{if}\:{x}<\mathrm{0}\:\Rightarrow{y}\:=\frac{{x}}{\mathrm{2}}\left(\mathrm{1}+\sqrt{\mathrm{5}}\xi\left({x}\right)\right)\:{or}\:{y}\:=\frac{{x}}{\mathrm{2}}\left(\mathrm{1}−\sqrt{\mathrm{5}}\xi\left({x}\right)\right)\:\Rightarrow \\ $$$$\frac{{dy}}{{dx}}\:=\frac{\mathrm{1}+\sqrt{\mathrm{5}}\xi\left({x}\right)}{\mathrm{2}}\:{or}\:\frac{{dy}}{{dx}}\:=\frac{\mathrm{1}−\sqrt{\mathrm{5}}\xi\left({x}\right)}{\mathrm{2}} \\ $$

Commented by ajfour last updated on 02/Jul/19

whats ξ , please lecture elaboratey, Sir.

$${whats}\:\xi\:,\:{please}\:{lecture}\:{elaboratey}, \\ $$$${Sir}. \\ $$

Commented by mathmax by abdo last updated on 02/Jul/19

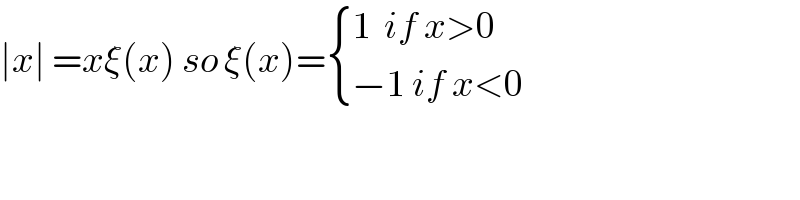
∣x∣ =xξ(x) so ξ(x)= { ((1 if x>0)),((−1 if x<0)) :}

$$\mid{x}\mid\:={x}\xi\left({x}\right)\:{so}\:\xi\left({x}\right)=\begin{cases}{\mathrm{1}\:\:{if}\:{x}>\mathrm{0}}\\{−\mathrm{1}\:{if}\:{x}<\mathrm{0}}\end{cases} \\ $$

Answered by Smail last updated on 02/Jul/19

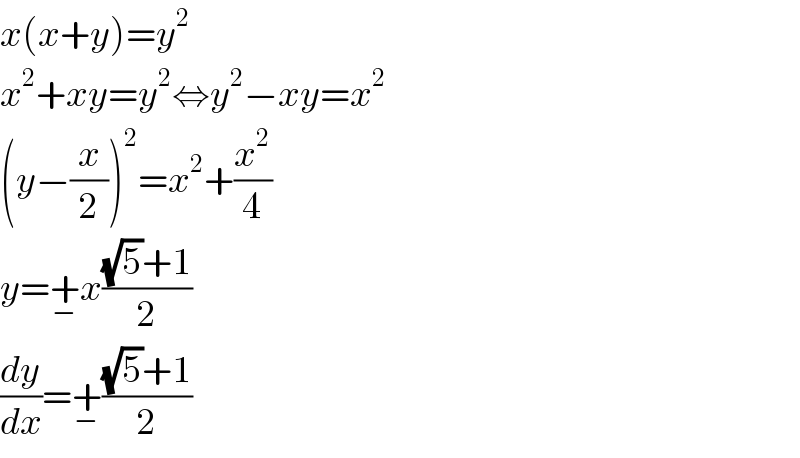
x(x+y)=y^2 x^2 +xy=y^2 ⇔y^2 −xy=x^2 (y−(x/2))^2 =x^2 +(x^2 /4) y=+_− x(((√5)+1)/2) (dy/dx)=+_− (((√5)+1)/2)

$${x}\left({x}+{y}\right)={y}^{\mathrm{2}} \\ $$$${x}^{\mathrm{2}} +{xy}={y}^{\mathrm{2}} \Leftrightarrow{y}^{\mathrm{2}} −{xy}={x}^{\mathrm{2}} \\ $$$$\left({y}−\frac{{x}}{\mathrm{2}}\right)^{\mathrm{2}} ={x}^{\mathrm{2}} +\frac{{x}^{\mathrm{2}} }{\mathrm{4}} \\ $$$${y}=\underset{−} {+}{x}\frac{\sqrt{\mathrm{5}}+\mathrm{1}}{\mathrm{2}} \\ $$$$\frac{{dy}}{{dx}}=\underset{−} {+}\frac{\sqrt{\mathrm{5}}+\mathrm{1}}{\mathrm{2}} \\ $$

Commented by Prithwish sen last updated on 02/Jul/19

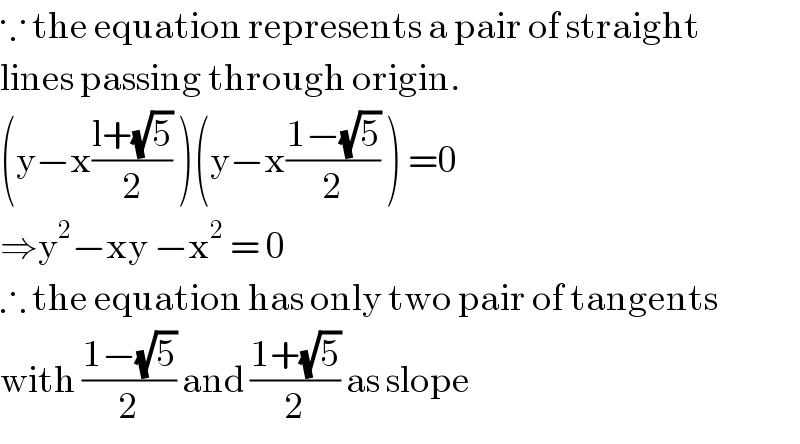
∵ the equation represents a pair of straight lines passing through origin. (y−x((l+(√5))/2) )(y−x((1−(√5))/2) ) =0 ⇒y^2 −xy −x^2 = 0 ∴ the equation has only two pair of tangents with ((1−(√5))/2) and ((1+(√5))/2) as slope

$$\because\:\mathrm{the}\:\mathrm{equation}\:\mathrm{represents}\:\mathrm{a}\:\mathrm{pair}\:\mathrm{of}\:\mathrm{straight} \\ $$$$\mathrm{lines}\:\mathrm{passing}\:\mathrm{through}\:\mathrm{origin}. \\ $$$$\left(\mathrm{y}−\mathrm{x}\frac{\mathrm{l}+\sqrt{\mathrm{5}}}{\mathrm{2}}\:\right)\left(\mathrm{y}−\mathrm{x}\frac{\mathrm{1}−\sqrt{\mathrm{5}}}{\mathrm{2}}\:\right)\:=\mathrm{0} \\ $$$$\Rightarrow\mathrm{y}^{\mathrm{2}} −\mathrm{xy}\:−\mathrm{x}^{\mathrm{2}} \:=\:\mathrm{0} \\ $$$$\therefore\:\mathrm{the}\:\mathrm{equation}\:\mathrm{has}\:\mathrm{only}\:\mathrm{two}\:\mathrm{pair}\:\mathrm{of}\:\mathrm{tangents} \\ $$$$\mathrm{with}\:\frac{\mathrm{1}−\sqrt{\mathrm{5}}}{\mathrm{2}}\:\mathrm{and}\:\frac{\mathrm{1}+\sqrt{\mathrm{5}}}{\mathrm{2}}\:\mathrm{as}\:\mathrm{slope} \\ $$

Commented by Rio Michael last updated on 02/Jul/19

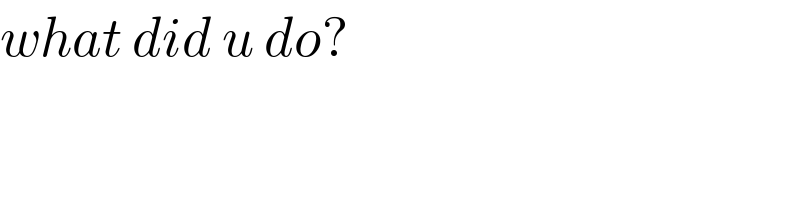
what did u do?

$${what}\:{did}\:{u}\:{do}? \\ $$

Commented by Smail last updated on 02/Jul/19

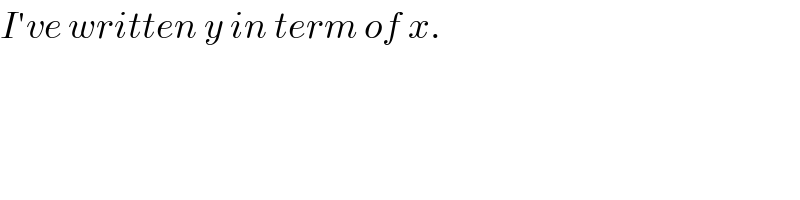
I′ve written y in term of x.

$${I}'{ve}\:{written}\:{y}\:{in}\:{term}\:{of}\:{x}. \\ $$

Commented by Rio Michael last updated on 02/Jul/19

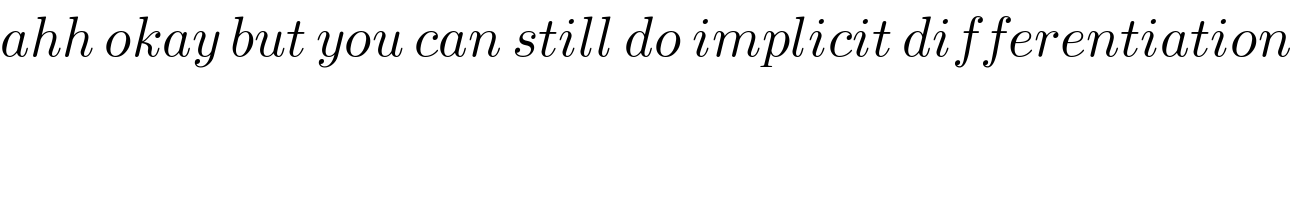
ahh okay but you can still do implicit differentiation

$${ahh}\:{okay}\:{but}\:{you}\:{can}\:{still}\:{do}\:{implicit}\:{differentiation} \\ $$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com