
Question Number 44306 by abdo.msup.com last updated on 26/Sep/18
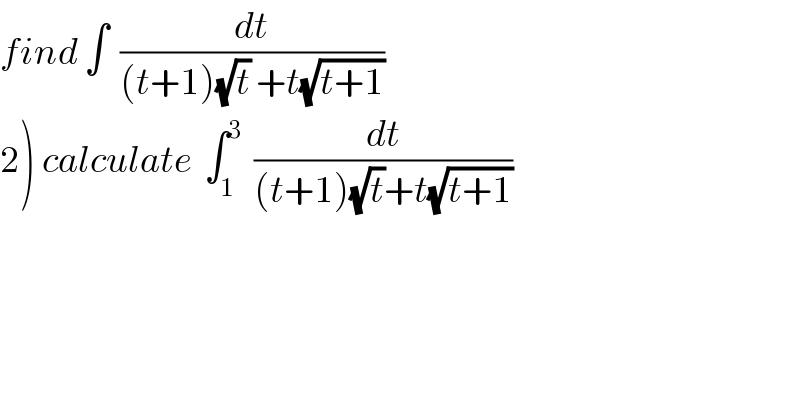
$${find}\:\int\:\:\frac{{dt}}{\left({t}+\mathrm{1}\right)\sqrt{{t}}\:+{t}\sqrt{{t}+\mathrm{1}}} \\ $$$$\left.\mathrm{2}\right)\:{calculate}\:\:\int_{\mathrm{1}} ^{\mathrm{3}} \:\:\frac{{dt}}{\left({t}+\mathrm{1}\right)\sqrt{{t}}+{t}\sqrt{{t}+\mathrm{1}}} \\ $$
Commented by maxmathsup by imad last updated on 29/Sep/18
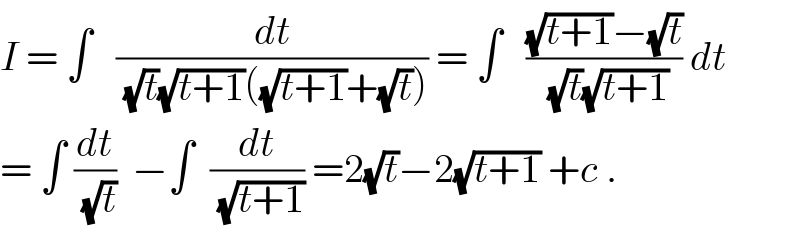
$${I}\:=\:\int\:\:\:\frac{{dt}}{\sqrt{{t}}\sqrt{{t}+\mathrm{1}}\left(\sqrt{{t}+\mathrm{1}}+\sqrt{{t}}\right)}\:=\:\int\:\:\:\frac{\sqrt{{t}+\mathrm{1}}−\sqrt{{t}}}{\sqrt{{t}}\sqrt{{t}+\mathrm{1}}}\:{dt} \\ $$$$=\:\int\:\frac{{dt}}{\sqrt{{t}}}\:\:−\int\:\:\frac{{dt}}{\sqrt{{t}+\mathrm{1}}}\:=\mathrm{2}\sqrt{{t}}−\mathrm{2}\sqrt{{t}+\mathrm{1}}\:+{c}\:. \\ $$
Commented by maxmathsup by imad last updated on 29/Sep/18
![∫_1 ^3 (dt/((t+1)(√t)+t(√(t+1)))) =[2(√t)−2(√(t+1))]_1 ^3 =2(√3)−4−2+2(√2)=2(√2)+2(√3)−6.](Q44464.png)
$$\int_{\mathrm{1}} ^{\mathrm{3}} \:\:\:\:\frac{{dt}}{\left({t}+\mathrm{1}\right)\sqrt{{t}}+{t}\sqrt{{t}+\mathrm{1}}}\:=\left[\mathrm{2}\sqrt{{t}}−\mathrm{2}\sqrt{{t}+\mathrm{1}}\right]_{\mathrm{1}} ^{\mathrm{3}} \:=\mathrm{2}\sqrt{\mathrm{3}}−\mathrm{4}−\mathrm{2}+\mathrm{2}\sqrt{\mathrm{2}}=\mathrm{2}\sqrt{\mathrm{2}}+\mathrm{2}\sqrt{\mathrm{3}}−\mathrm{6}. \\ $$
