Question and Answers Forum

All Questions      Topic List

Integration Questions

Previous in All Question      Next in All Question      

Previous in Integration      Next in Integration      

Question Number 147680 by mathmax by abdo last updated on 22/Jul/21

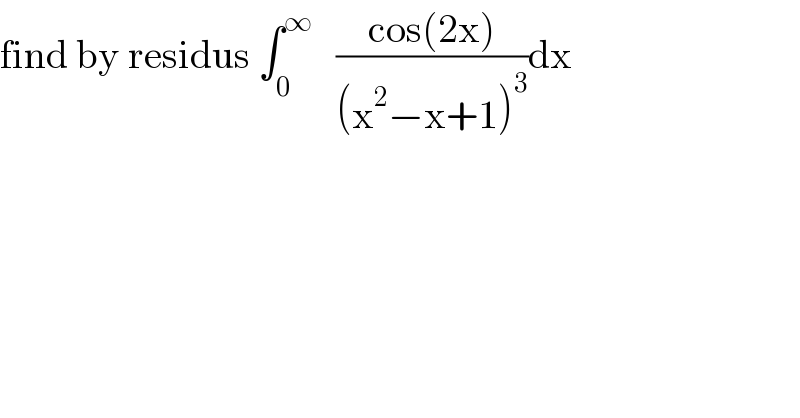
find by residus ∫_0 ^∞ ((cos(2x))/((x^2 −x+1)^3 ))dx

$$\mathrm{find}\:\mathrm{by}\:\mathrm{residus}\:\int_{\mathrm{0}} ^{\infty} \:\:\:\frac{\mathrm{cos}\left(\mathrm{2x}\right)}{\left(\mathrm{x}^{\mathrm{2}} −\mathrm{x}+\mathrm{1}\right)^{\mathrm{3}} }\mathrm{dx} \\ $$

Commented by mathmax by abdo last updated on 23/Jul/21

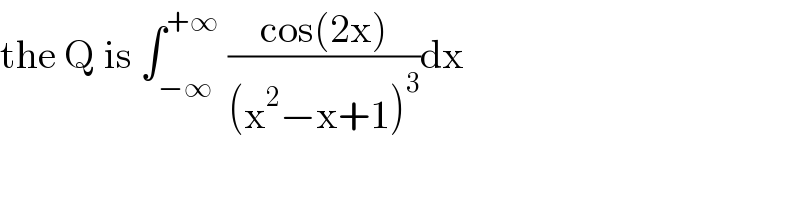
the Q is ∫_(−∞) ^(+∞) ((cos(2x))/((x^2 −x+1)^3 ))dx

$$\mathrm{the}\:\mathrm{Q}\:\mathrm{is}\:\int_{−\infty} ^{+\infty} \:\frac{\mathrm{cos}\left(\mathrm{2x}\right)}{\left(\mathrm{x}^{\mathrm{2}} −\mathrm{x}+\mathrm{1}\right)^{\mathrm{3}} }\mathrm{dx} \\ $$

Commented by mathmax by abdo last updated on 23/Jul/21

let f(a)=∫_(−∞) ^(+∞) ((cos(2x))/(x^2 −x+a))dx with a>(1/4) f^′ (a)=−∫_(−∞) ^(+∞) ((cos(2x))/((x^2 −x+a)^2 )) ⇒f^((2)) (a)=∫_(−∞) ^(+∞) ((2(x^2 −x+a)cos(2a))/((x^2 −x+a)^4 ))dx =2 ∫_(−∞) ^(+∞) ((cos(2x))/((x^2 −x+a)^3 ))dx ⇒∫_(−∞) ^(+∞) ((cos(2x))/((x^2 −x+1)^3 ))dx=(1/2)f^(′′) (1) we have f(a)=Re(∫_(−∞) ^(+∞) (e^(2ix) /(x^2 −x+a))dx)let Λ(z)=(e^(2iz) /(z^2 −z+a)) poles? Δ=1−4a<0 ⇒z_1 =((1+i(√(4a−1)))/2) and z_2 =((1−i(√(4a−1)))/2) Λ(z)=(e^(2iz) /((z−z_1 )(z−z_2 ))) ∫_R Λ(z)dz=2iπ Res(Λ,z_1 ) =(e^(2iz_1 ) /(z_1 −z_2 )) =(e^(2i(((1+i(√(4a−1)))/2))) /(i(√(4a−1)))) =(e^(i−(√(4a−1))) /(i(√(4a−1))))=((e^(−(√(4a−1))) (cos(1)+isin(1)))/(i(√(4a−1)))) ⇒ ∫_R Λ(z)dz=2iπ×((e^(−(√(4a−1))) (cos(1)+isin(1))/(i(√(4a−1)))) =((2π)/( (√(4a−1))))e^(−(√(4a−1))) (cos(1)+isin(1)) ⇒ f(a)=((2πcos(1))/( (√(4a−1))))e^(−(√(4a−1))) ⇒f(a)=2πcos(1)(4a−1)^(−(1/2)) e^(−(√(4a−1))) ⇒f^′ (a)=2πcos(1)(−2a(4a−1)^(−(3/2)) e^(−(√(4a−1))) +(4a−1)^(−(1/2)) (−(4/(2(√(4a−1))))e^(−(√(4a−1))) ) =2πcos(1){−2a(4a−1)^(−(3/2)) −2(4a−1)^(−(3/2)) }e^(−(√(4a−1))) =−4πcos(1){(a+1)(4a−1)^(−(3/2)) }e^(−(√(4a−1))) ⇒ f^((2)) (a)=−4πcos(1){(4a−1)^(−(3/2)) −6(a+1)(4a−1)^(−(5/2)) )e^(−(√(4a−1))) −(2/( (√(4a−1))))e^(−(√(4a−1))) (a+1)(4a−1)^(−(3/2)) } ⇒ f^(′′) (1)=−4πcos(1){(3^(−(3/2)) −12.3^(−(5/2)) )e^(−(√3)) −(2/( (√3)))e^(−(√3)) (2).3^(−(3/2)) } and Ψ=(1/2)f^((2)) (1)

$$\mathrm{let}\:\mathrm{f}\left(\mathrm{a}\right)=\int_{−\infty} ^{+\infty} \:\frac{\mathrm{cos}\left(\mathrm{2x}\right)}{\mathrm{x}^{\mathrm{2}} −\mathrm{x}+\mathrm{a}}\mathrm{dx}\:\:\:\mathrm{with}\:\mathrm{a}>\frac{\mathrm{1}}{\mathrm{4}} \\ $$$$\mathrm{f}^{'} \left(\mathrm{a}\right)=−\int_{−\infty} ^{+\infty} \:\:\frac{\mathrm{cos}\left(\mathrm{2x}\right)}{\left(\mathrm{x}^{\mathrm{2}} −\mathrm{x}+\mathrm{a}\right)^{\mathrm{2}} }\:\Rightarrow\mathrm{f}^{\left(\mathrm{2}\right)} \left(\mathrm{a}\right)=\int_{−\infty} ^{+\infty} \:\frac{\mathrm{2}\left(\mathrm{x}^{\mathrm{2}} −\mathrm{x}+\mathrm{a}\right)\mathrm{cos}\left(\mathrm{2a}\right)}{\left(\mathrm{x}^{\mathrm{2}} −\mathrm{x}+\mathrm{a}\right)^{\mathrm{4}} }\mathrm{dx} \\ $$$$=\mathrm{2}\:\int_{−\infty} ^{+\infty} \:\frac{\mathrm{cos}\left(\mathrm{2x}\right)}{\left(\mathrm{x}^{\mathrm{2}} −\mathrm{x}+\mathrm{a}\right)^{\mathrm{3}} }\mathrm{dx}\:\Rightarrow\int_{−\infty} ^{+\infty} \:\frac{\mathrm{cos}\left(\mathrm{2x}\right)}{\left(\mathrm{x}^{\mathrm{2}} −\mathrm{x}+\mathrm{1}\right)^{\mathrm{3}} }\mathrm{dx}=\frac{\mathrm{1}}{\mathrm{2}}\mathrm{f}^{''} \left(\mathrm{1}\right) \\ $$$$\mathrm{we}\:\mathrm{have}\:\mathrm{f}\left(\mathrm{a}\right)=\mathrm{Re}\left(\int_{−\infty} ^{+\infty} \:\frac{\mathrm{e}^{\mathrm{2ix}} }{\mathrm{x}^{\mathrm{2}} −\mathrm{x}+\mathrm{a}}\mathrm{dx}\right)\mathrm{let}\:\Lambda\left(\mathrm{z}\right)=\frac{\mathrm{e}^{\mathrm{2iz}} }{\mathrm{z}^{\mathrm{2}} −\mathrm{z}+\mathrm{a}}\:\mathrm{poles}? \\ $$$$\Delta=\mathrm{1}−\mathrm{4a}<\mathrm{0}\:\Rightarrow\mathrm{z}_{\mathrm{1}} =\frac{\mathrm{1}+\mathrm{i}\sqrt{\mathrm{4a}−\mathrm{1}}}{\mathrm{2}}\:\:\mathrm{and}\:\mathrm{z}_{\mathrm{2}} =\frac{\mathrm{1}−\mathrm{i}\sqrt{\mathrm{4a}−\mathrm{1}}}{\mathrm{2}} \\ $$$$\Lambda\left(\mathrm{z}\right)=\frac{\mathrm{e}^{\mathrm{2iz}} }{\left(\mathrm{z}−\mathrm{z}_{\mathrm{1}} \right)\left(\mathrm{z}−\mathrm{z}_{\mathrm{2}} \right)} \\ $$$$\int_{\mathrm{R}} \Lambda\left(\mathrm{z}\right)\mathrm{dz}=\mathrm{2i}\pi\:\mathrm{Res}\left(\Lambda,\mathrm{z}_{\mathrm{1}} \right)\:=\frac{\mathrm{e}^{\mathrm{2iz}_{\mathrm{1}} } }{\mathrm{z}_{\mathrm{1}} −\mathrm{z}_{\mathrm{2}} } \\ $$$$=\frac{\mathrm{e}^{\mathrm{2i}\left(\frac{\mathrm{1}+\mathrm{i}\sqrt{\mathrm{4a}−\mathrm{1}}}{\mathrm{2}}\right)} }{\mathrm{i}\sqrt{\mathrm{4a}−\mathrm{1}}}\:=\frac{\mathrm{e}^{\mathrm{i}−\sqrt{\mathrm{4a}−\mathrm{1}}} }{\mathrm{i}\sqrt{\mathrm{4a}−\mathrm{1}}}=\frac{\mathrm{e}^{−\sqrt{\mathrm{4a}−\mathrm{1}}} \left(\mathrm{cos}\left(\mathrm{1}\right)+\mathrm{isin}\left(\mathrm{1}\right)\right)}{\mathrm{i}\sqrt{\mathrm{4a}−\mathrm{1}}}\:\Rightarrow \\ $$$$\int_{\mathrm{R}} \Lambda\left(\mathrm{z}\right)\mathrm{dz}=\mathrm{2i}\pi×\frac{\mathrm{e}^{−\sqrt{\mathrm{4a}−\mathrm{1}}} \left(\mathrm{cos}\left(\mathrm{1}\right)+\mathrm{isin}\left(\mathrm{1}\right)\right.}{\mathrm{i}\sqrt{\mathrm{4a}−\mathrm{1}}} \\ $$$$=\frac{\mathrm{2}\pi}{\:\sqrt{\mathrm{4a}−\mathrm{1}}}\mathrm{e}^{−\sqrt{\mathrm{4a}−\mathrm{1}}} \left(\mathrm{cos}\left(\mathrm{1}\right)+\mathrm{isin}\left(\mathrm{1}\right)\right)\:\Rightarrow \\ $$$$\mathrm{f}\left(\mathrm{a}\right)=\frac{\mathrm{2}\pi\mathrm{cos}\left(\mathrm{1}\right)}{\:\sqrt{\mathrm{4a}−\mathrm{1}}}\mathrm{e}^{−\sqrt{\mathrm{4a}−\mathrm{1}}} \:\:\Rightarrow\mathrm{f}\left(\mathrm{a}\right)=\mathrm{2}\pi\mathrm{cos}\left(\mathrm{1}\right)\left(\mathrm{4a}−\mathrm{1}\right)^{−\frac{\mathrm{1}}{\mathrm{2}}} \:\mathrm{e}^{−\sqrt{\mathrm{4a}−\mathrm{1}}} \\ $$$$\Rightarrow\mathrm{f}^{'} \left(\mathrm{a}\right)=\mathrm{2}\pi\mathrm{cos}\left(\mathrm{1}\right)\left(−\mathrm{2a}\left(\mathrm{4a}−\mathrm{1}\right)^{−\frac{\mathrm{3}}{\mathrm{2}}} \mathrm{e}^{−\sqrt{\mathrm{4a}−\mathrm{1}}} \right. \\ $$$$+\left(\mathrm{4a}−\mathrm{1}\right)^{−\frac{\mathrm{1}}{\mathrm{2}}} \left(−\frac{\mathrm{4}}{\mathrm{2}\sqrt{\mathrm{4a}−\mathrm{1}}}\mathrm{e}^{−\sqrt{\mathrm{4a}−\mathrm{1}}} \right) \\ $$$$=\mathrm{2}\pi\mathrm{cos}\left(\mathrm{1}\right)\left\{−\mathrm{2a}\left(\mathrm{4a}−\mathrm{1}\right)^{−\frac{\mathrm{3}}{\mathrm{2}}} −\mathrm{2}\left(\mathrm{4a}−\mathrm{1}\right)^{−\frac{\mathrm{3}}{\mathrm{2}}} \right\}\mathrm{e}^{−\sqrt{\mathrm{4a}−\mathrm{1}}} \\ $$$$=−\mathrm{4}\pi\mathrm{cos}\left(\mathrm{1}\right)\left\{\left(\mathrm{a}+\mathrm{1}\right)\left(\mathrm{4a}−\mathrm{1}\right)^{−\frac{\mathrm{3}}{\mathrm{2}}} \right\}\mathrm{e}^{−\sqrt{\mathrm{4a}−\mathrm{1}}} \:\Rightarrow \\ $$$$\mathrm{f}^{\left(\mathrm{2}\right)} \left(\mathrm{a}\right)=−\mathrm{4}\pi\mathrm{cos}\left(\mathrm{1}\right)\left\{\left(\mathrm{4a}−\mathrm{1}\right)^{−\frac{\mathrm{3}}{\mathrm{2}}} −\mathrm{6}\left(\mathrm{a}+\mathrm{1}\right)\left(\mathrm{4a}−\mathrm{1}\right)^{−\frac{\mathrm{5}}{\mathrm{2}}} \right)\mathrm{e}^{−\sqrt{\mathrm{4a}−\mathrm{1}}} \\ $$$$\left.−\frac{\mathrm{2}}{\:\sqrt{\mathrm{4a}−\mathrm{1}}}\mathrm{e}^{−\sqrt{\mathrm{4a}−\mathrm{1}}} \left(\mathrm{a}+\mathrm{1}\right)\left(\mathrm{4a}−\mathrm{1}\right)^{−\frac{\mathrm{3}}{\mathrm{2}}} \right\}\:\Rightarrow \\ $$$$\mathrm{f}^{''} \left(\mathrm{1}\right)=−\mathrm{4}\pi\mathrm{cos}\left(\mathrm{1}\right)\left\{\left(\mathrm{3}^{−\frac{\mathrm{3}}{\mathrm{2}}} −\mathrm{12}.\mathrm{3}^{−\frac{\mathrm{5}}{\mathrm{2}}} \right)\mathrm{e}^{−\sqrt{\mathrm{3}}} −\frac{\mathrm{2}}{\:\sqrt{\mathrm{3}}}\mathrm{e}^{−\sqrt{\mathrm{3}}} \left(\mathrm{2}\right).\mathrm{3}^{−\frac{\mathrm{3}}{\mathrm{2}}} \right\} \\ $$$$\mathrm{and}\:\Psi=\frac{\mathrm{1}}{\mathrm{2}}\mathrm{f}^{\left(\mathrm{2}\right)} \left(\mathrm{1}\right) \\ $$$$ \\ $$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com