
Question Number 200388 by mr W last updated on 18/Nov/23

$${find}\:{all}\:{values}\:{for}\:{k}\:{such}\:{that}\:{the}\:{eq}. \\ $$$${x}^{\mathrm{3}} −\mathrm{13}{x}+{k}=\mathrm{0}\:{has}\:{three}\:{integer}\:{roots}. \\ $$
Answered by Frix last updated on 18/Nov/23
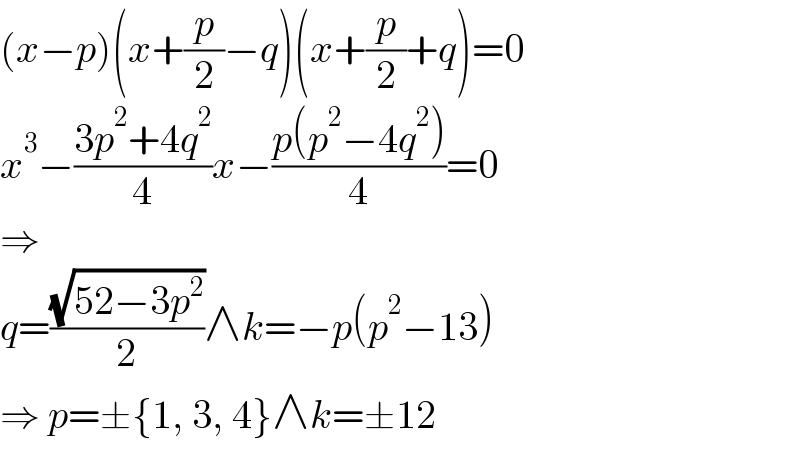
$$\left({x}−{p}\right)\left({x}+\frac{{p}}{\mathrm{2}}−{q}\right)\left({x}+\frac{{p}}{\mathrm{2}}+{q}\right)=\mathrm{0} \\ $$$${x}^{\mathrm{3}} −\frac{\mathrm{3}{p}^{\mathrm{2}} +\mathrm{4}{q}^{\mathrm{2}} }{\mathrm{4}}{x}−\frac{{p}\left({p}^{\mathrm{2}} −\mathrm{4}{q}^{\mathrm{2}} \right)}{\mathrm{4}}=\mathrm{0} \\ $$$$\Rightarrow \\ $$$${q}=\frac{\sqrt{\mathrm{52}−\mathrm{3}{p}^{\mathrm{2}} }}{\mathrm{2}}\wedge{k}=−{p}\left({p}^{\mathrm{2}} −\mathrm{13}\right) \\ $$$$\Rightarrow\:{p}=\pm\left\{\mathrm{1},\:\mathrm{3},\:\mathrm{4}\right\}\wedge{k}=\pm\mathrm{12} \\ $$
Commented by mr W last updated on 18/Nov/23

$${thanks}\:{sir}! \\ $$
