Question and Answers Forum

All Questions      Topic List

Algebra Questions

Previous in All Question      Next in All Question      

Previous in Algebra      Next in Algebra      

Question Number 179436 by Shrinava last updated on 29/Oct/22

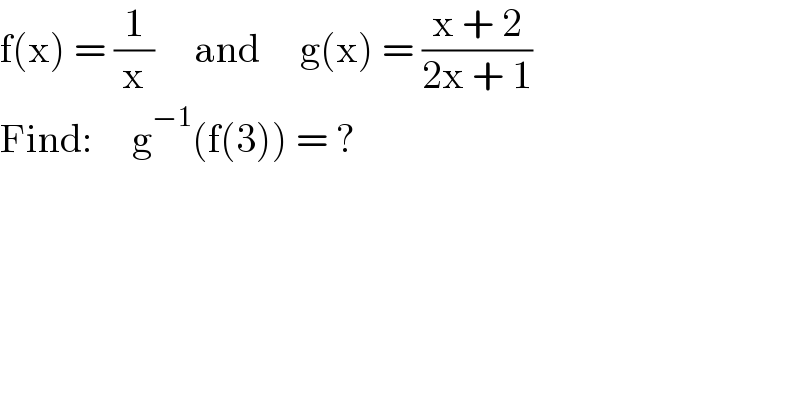
f(x) = (1/x) and g(x) = ((x + 2)/(2x + 1)) Find: g^(−1) (f(3)) = ?

$$\mathrm{f}\left(\mathrm{x}\right)\:=\:\frac{\mathrm{1}}{\mathrm{x}}\:\:\:\:\:\mathrm{and}\:\:\:\:\:\mathrm{g}\left(\mathrm{x}\right)\:=\:\frac{\mathrm{x}\:+\:\mathrm{2}}{\mathrm{2x}\:+\:\mathrm{1}} \\ $$$$\mathrm{Find}:\:\:\:\:\:\mathrm{g}^{−\mathrm{1}} \left(\mathrm{f}\left(\mathrm{3}\right)\right)\:=\:? \\ $$

Commented by cherokeesay last updated on 29/Oct/22

y=((x+2)/(2x+1))⇔2xy+y=x+2⇒ x(2y−1)=2−y⇔x=((2−y)/(2y−1)) ⇔g^(−1) (x)=((2−x)/(2x−1)) g^(−1) [f(3)]=((2−(1/3))/(2×(1/3)−1))=−5

$${y}=\frac{{x}+\mathrm{2}}{\mathrm{2}{x}+\mathrm{1}}\Leftrightarrow\mathrm{2}{xy}+{y}={x}+\mathrm{2}\Rightarrow \\ $$$${x}\left(\mathrm{2}{y}−\mathrm{1}\right)=\mathrm{2}−{y}\Leftrightarrow{x}=\frac{\mathrm{2}−{y}}{\mathrm{2}{y}−\mathrm{1}} \\ $$$$\Leftrightarrow{g}^{−\mathrm{1}} \left({x}\right)=\frac{\mathrm{2}−{x}}{\mathrm{2}{x}−\mathrm{1}} \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:{g}^{−\mathrm{1}} \left[{f}\left(\mathrm{3}\right)\right]=\frac{\mathrm{2}−\frac{\mathrm{1}}{\mathrm{3}}}{\mathrm{2}×\frac{\mathrm{1}}{\mathrm{3}}−\mathrm{1}}=−\mathrm{5} \\ $$

Answered by ARUNG_Brandon_MBU last updated on 29/Oct/22

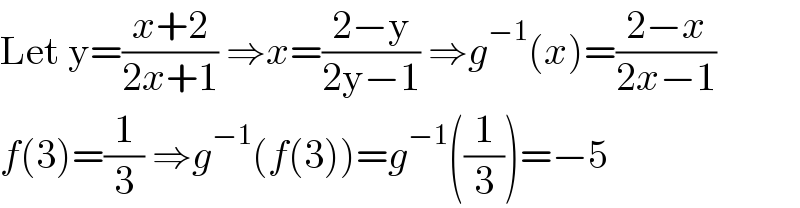
Let y=((x+2)/(2x+1)) ⇒x=((2−y)/(2y−1)) ⇒g^(−1) (x)=((2−x)/(2x−1)) f(3)=(1/3) ⇒g^(−1) (f(3))=g^(−1) ((1/3))=−5

$$\mathrm{Let}\:\mathrm{y}=\frac{{x}+\mathrm{2}}{\mathrm{2}{x}+\mathrm{1}}\:\Rightarrow{x}=\frac{\mathrm{2}−\mathrm{y}}{\mathrm{2y}−\mathrm{1}}\:\Rightarrow{g}^{−\mathrm{1}} \left({x}\right)=\frac{\mathrm{2}−{x}}{\mathrm{2}{x}−\mathrm{1}} \\ $$$${f}\left(\mathrm{3}\right)=\frac{\mathrm{1}}{\mathrm{3}}\:\Rightarrow{g}^{−\mathrm{1}} \left({f}\left(\mathrm{3}\right)\right)={g}^{−\mathrm{1}} \left(\frac{\mathrm{1}}{\mathrm{3}}\right)=−\mathrm{5} \\ $$

Answered by mr W last updated on 29/Oct/22

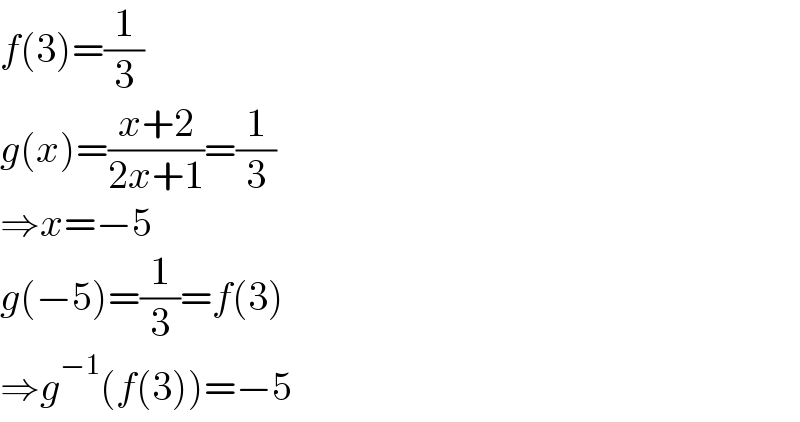
f(3)=(1/3) g(x)=((x+2)/(2x+1))=(1/3) ⇒x=−5 g(−5)=(1/3)=f(3) ⇒g^(−1) (f(3))=−5

$${f}\left(\mathrm{3}\right)=\frac{\mathrm{1}}{\mathrm{3}} \\ $$$${g}\left({x}\right)=\frac{{x}+\mathrm{2}}{\mathrm{2}{x}+\mathrm{1}}=\frac{\mathrm{1}}{\mathrm{3}} \\ $$$$\Rightarrow{x}=−\mathrm{5} \\ $$$${g}\left(−\mathrm{5}\right)=\frac{\mathrm{1}}{\mathrm{3}}={f}\left(\mathrm{3}\right) \\ $$$$\Rightarrow{g}^{−\mathrm{1}} \left({f}\left(\mathrm{3}\right)\right)=−\mathrm{5} \\ $$

Commented by Shrinava last updated on 29/Oct/22

thank you dear professors

$$\mathrm{thank}\:\mathrm{you}\:\mathrm{dear}\:\mathrm{professors} \\ $$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com