Question and Answers Forum

All Questions      Topic List

Others Questions

Previous in All Question      Next in All Question      

Previous in Others      Next in Others      

Question Number 67148 by Cmr 237 last updated on 29/Aug/19

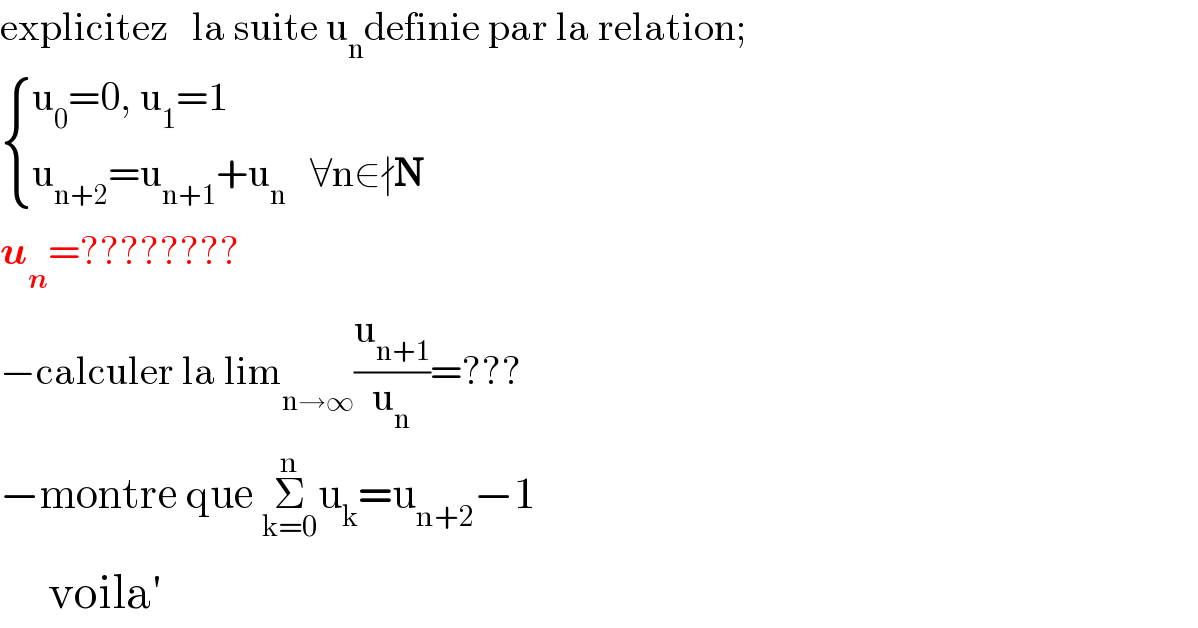
explicitez la suite u_n definie par la relation; { ((u_0 =0, u_1 =1)),((u_(n+2) =u_(n+1) +u_n ∀n∈∤N)) :} u_n =???????? −calculer la lim _(n→∞) (u_(n+1) /u_n )=??? −montre que Σ_(k=0) ^n u_k =u_(n+2) −1 voila^′

$$\mathrm{explicitez}\:\:\:\mathrm{la}\:\mathrm{suite}\:\mathrm{u}_{\mathrm{n}} \mathrm{definie}\:\mathrm{par}\:\mathrm{la}\:\mathrm{relation}; \\ $$$$\begin{cases}{\mathrm{u}_{\mathrm{0}} =\mathrm{0},\:\mathrm{u}_{\mathrm{1}} =\mathrm{1}}\\{\mathrm{u}_{\mathrm{n}+\mathrm{2}} =\mathrm{u}_{\mathrm{n}+\mathrm{1}} +\mathrm{u}_{\mathrm{n}} \:\:\:\forall\mathrm{n}\in\nmid\boldsymbol{\mathrm{N}}}\end{cases} \\ $$$$\boldsymbol{{u}}_{\boldsymbol{{n}}} =???????? \\ $$$$−\mathrm{calculer}\:\mathrm{la}\:\mathrm{lim}\underset{\mathrm{n}\rightarrow\infty} {\:}\frac{\mathrm{u}_{\mathrm{n}+\mathrm{1}} }{\mathrm{u}_{\mathrm{n}} }=??? \\ $$$$−\mathrm{montre}\:\mathrm{que}\:\underset{\mathrm{k}=\mathrm{0}} {\overset{\mathrm{n}} {\sum}}\mathrm{u}_{\mathrm{k}} =\mathrm{u}_{\mathrm{n}+\mathrm{2}} −\mathrm{1} \\ $$$$\:\:\:\:\:\:\mathrm{voila}^{'} \\ $$

Commented by Tony Lin last updated on 23/Aug/19

Fibonacci Sequence u_n =(1/(√5))[(((1+(√5))/2))^n −(((1−(√5))/2))^n ]

$${Fibonacci}\:{Sequence} \\ $$$${u}_{{n}} =\frac{\mathrm{1}}{\sqrt{\mathrm{5}}}\left[\left(\frac{\mathrm{1}+\sqrt{\mathrm{5}}}{\mathrm{2}}\right)^{{n}} −\left(\frac{\mathrm{1}−\sqrt{\mathrm{5}}}{\mathrm{2}}\right)^{{n}} \right] \\ $$

Commented by mathmax by abdo last updated on 23/Aug/19

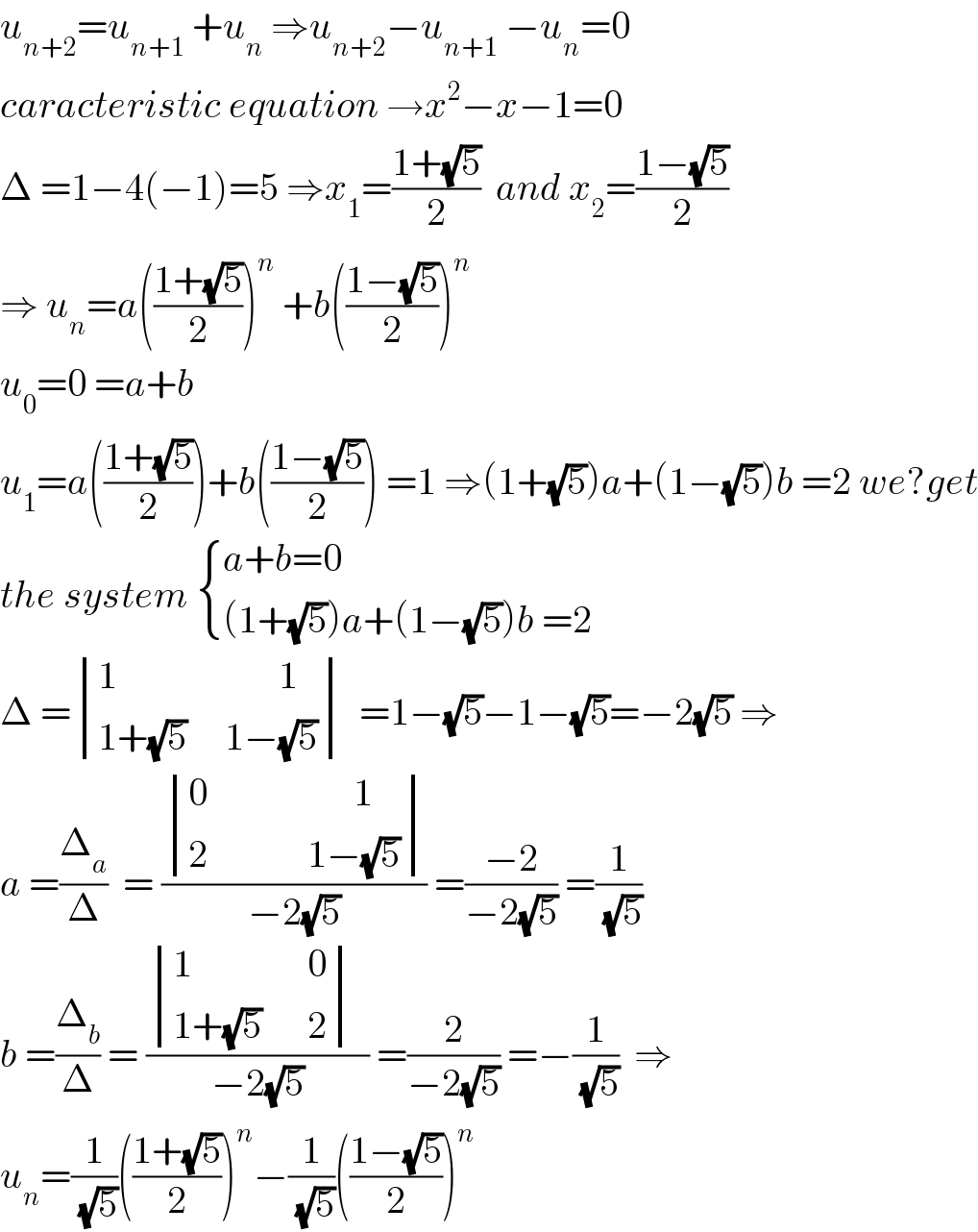
u_(n+2) =u_(n+1) +u_n ⇒u_(n+2) −u_(n+1) −u_n =0 caracteristic equation →x^2 −x−1=0 Δ =1−4(−1)=5 ⇒x_1 =((1+(√5))/2) and x_2 =((1−(√5))/2) ⇒ u_n =a(((1+(√5))/2))^n +b(((1−(√5))/2))^n u_0 =0 =a+b u_1 =a(((1+(√5))/2))+b(((1−(√5))/2)) =1 ⇒(1+(√5))a+(1−(√5))b =2 we?get the system { ((a+b=0)),(((1+(√5))a+(1−(√5))b =2)) :} Δ = determinant (((1 1)),((1+(√5) 1−(√5)))) =1−(√5)−1−(√5)=−2(√5) ⇒ a =(Δ_a /Δ) = ( determinant (((0 1)),((2 1−(√5))))/(−2(√5))) =((−2)/(−2(√5))) =(1/(√5)) b =(Δ_b /Δ) = (( determinant (((1 0)),((1+(√5) 2))) )/(−2(√5))) =(2/(−2(√5))) =−(1/(√5)) ⇒ u_n =(1/(√5))(((1+(√5))/2))^n −(1/(√5))(((1−(√5))/2))^n

$${u}_{{n}+\mathrm{2}} ={u}_{{n}+\mathrm{1}} \:+{u}_{{n}} \:\Rightarrow{u}_{{n}+\mathrm{2}} −{u}_{{n}+\mathrm{1}} \:−{u}_{{n}} =\mathrm{0}\: \\ $$$${caracteristic}\:{equation}\:\rightarrow{x}^{\mathrm{2}} −{x}−\mathrm{1}=\mathrm{0} \\ $$$$\Delta\:=\mathrm{1}−\mathrm{4}\left(−\mathrm{1}\right)=\mathrm{5}\:\Rightarrow{x}_{\mathrm{1}} =\frac{\mathrm{1}+\sqrt{\mathrm{5}}}{\mathrm{2}}\:\:{and}\:{x}_{\mathrm{2}} =\frac{\mathrm{1}−\sqrt{\mathrm{5}}}{\mathrm{2}} \\ $$$$\Rightarrow\:{u}_{{n}} ={a}\left(\frac{\mathrm{1}+\sqrt{\mathrm{5}}}{\mathrm{2}}\right)^{{n}} \:+{b}\left(\frac{\mathrm{1}−\sqrt{\mathrm{5}}}{\mathrm{2}}\right)^{{n}} \\ $$$${u}_{\mathrm{0}} =\mathrm{0}\:={a}+{b} \\ $$$${u}_{\mathrm{1}} ={a}\left(\frac{\mathrm{1}+\sqrt{\mathrm{5}}}{\mathrm{2}}\right)+{b}\left(\frac{\mathrm{1}−\sqrt{\mathrm{5}}}{\mathrm{2}}\right)\:=\mathrm{1}\:\Rightarrow\left(\mathrm{1}+\sqrt{\mathrm{5}}\right){a}+\left(\mathrm{1}−\sqrt{\mathrm{5}}\right){b}\:=\mathrm{2}\:{we}?{get} \\ $$$${the}\:{system}\:\begin{cases}{{a}+{b}=\mathrm{0}}\\{\left(\mathrm{1}+\sqrt{\mathrm{5}}\right){a}+\left(\mathrm{1}−\sqrt{\mathrm{5}}\right){b}\:=\mathrm{2}}\end{cases} \\ $$$$\Delta\:=\begin{vmatrix}{\mathrm{1}\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\mathrm{1}}\\{\mathrm{1}+\sqrt{\mathrm{5}}\:\:\:\:\:\mathrm{1}−\sqrt{\mathrm{5}}}\end{vmatrix}\:\:=\mathrm{1}−\sqrt{\mathrm{5}}−\mathrm{1}−\sqrt{\mathrm{5}}=−\mathrm{2}\sqrt{\mathrm{5}}\:\Rightarrow \\ $$$${a}\:=\frac{\Delta_{{a}} }{\Delta}\:\:=\:\frac{\begin{vmatrix}{\mathrm{0}\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\mathrm{1}}\\{\mathrm{2}\:\:\:\:\:\:\:\:\:\:\:\:\:\mathrm{1}−\sqrt{\mathrm{5}}}\end{vmatrix}}{−\mathrm{2}\sqrt{\mathrm{5}}}\:=\frac{−\mathrm{2}}{−\mathrm{2}\sqrt{\mathrm{5}}}\:=\frac{\mathrm{1}}{\sqrt{\mathrm{5}}} \\ $$$${b}\:=\frac{\Delta_{{b}} }{\Delta}\:=\:\frac{\begin{vmatrix}{\mathrm{1}\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\mathrm{0}}\\{\mathrm{1}+\sqrt{\mathrm{5}}\:\:\:\:\:\:\mathrm{2}}\end{vmatrix}\:\:}{−\mathrm{2}\sqrt{\mathrm{5}}}\:=\frac{\mathrm{2}}{−\mathrm{2}\sqrt{\mathrm{5}}}\:=−\frac{\mathrm{1}}{\sqrt{\mathrm{5}}}\:\:\Rightarrow \\ $$$${u}_{{n}} =\frac{\mathrm{1}}{\sqrt{\mathrm{5}}}\left(\frac{\mathrm{1}+\sqrt{\mathrm{5}}}{\mathrm{2}}\right)^{{n}} −\frac{\mathrm{1}}{\sqrt{\mathrm{5}}}\left(\frac{\mathrm{1}−\sqrt{\mathrm{5}}}{\mathrm{2}}\right)^{{n}} \: \\ $$

Commented by mathmax by abdo last updated on 23/Aug/19

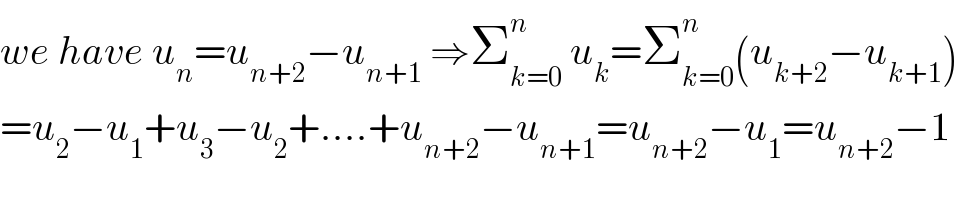
we have u_n =u_(n+2) −u_(n+1) ⇒Σ_(k=0) ^n u_k =Σ_(k=0) ^n (u_(k+2) −u_(k+1) ) =u_2 −u_1 +u_3 −u_2 +....+u_(n+2) −u_(n+1) =u_(n+2) −u_1 =u_(n+2) −1

$${we}\:{have}\:{u}_{{n}} ={u}_{{n}+\mathrm{2}} −{u}_{{n}+\mathrm{1}} \:\Rightarrow\sum_{{k}=\mathrm{0}} ^{{n}} \:{u}_{{k}} =\sum_{{k}=\mathrm{0}} ^{{n}} \left({u}_{{k}+\mathrm{2}} −{u}_{{k}+\mathrm{1}} \right) \\ $$$$={u}_{\mathrm{2}} −{u}_{\mathrm{1}} +{u}_{\mathrm{3}} −{u}_{\mathrm{2}} +....+{u}_{{n}+\mathrm{2}} −{u}_{{n}+\mathrm{1}} ={u}_{{n}+\mathrm{2}} −{u}_{\mathrm{1}} ={u}_{{n}+\mathrm{2}} −\mathrm{1} \\ $$

Commented by Kunal12588 last updated on 23/Aug/19

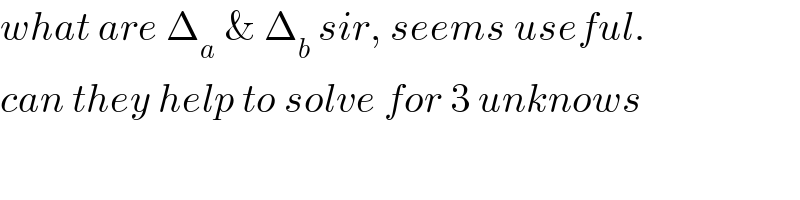
what are Δ_a & Δ_b sir, seems useful. can they help to solve for 3 unknows

$${what}\:{are}\:\Delta_{{a}} \:\&\:\Delta_{{b}} \:{sir},\:{seems}\:{useful}. \\ $$$${can}\:{they}\:{help}\:{to}\:{solve}\:{for}\:\mathrm{3}\:{unknows} \\ $$

Commented by mathmax by abdo last updated on 23/Aug/19

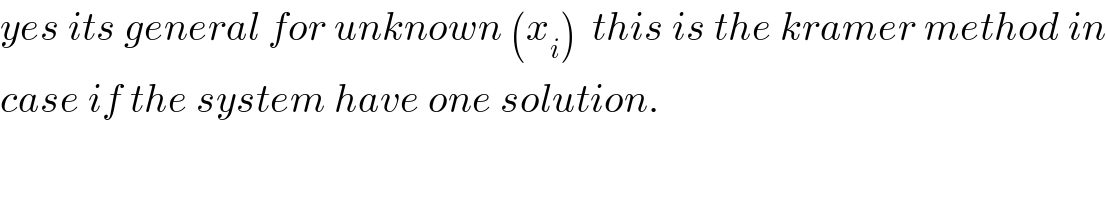
yes its general for unknown (x_i ) this is the kramer method in case if the system have one solution.

$${yes}\:{its}\:{general}\:{for}\:{unknown}\:\left({x}_{{i}} \right)\:\:{this}\:{is}\:{the}\:{kramer}\:{method}\:{in} \\ $$$${case}\:{if}\:{the}\:{system}\:{have}\:{one}\:{solution}. \\ $$

Commented by mathmax by abdo last updated on 23/Aug/19

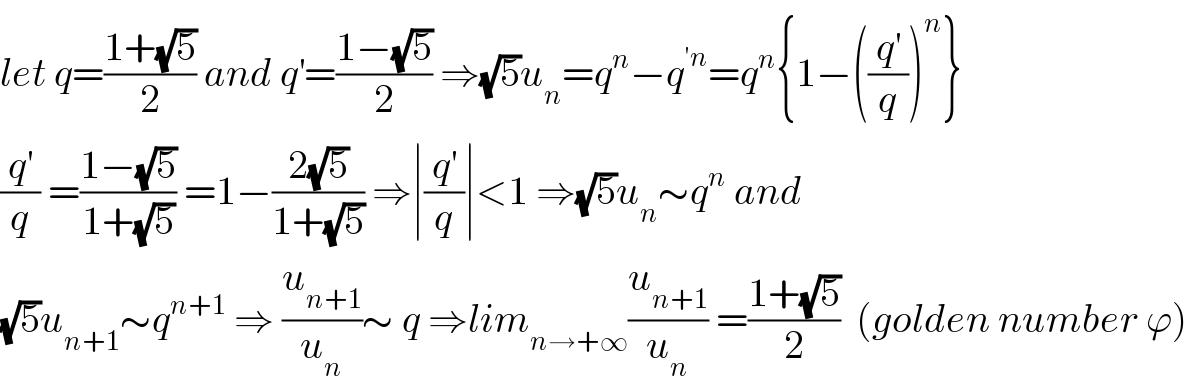
let q=((1+(√5))/2) and q^′ =((1−(√5))/2) ⇒(√5)u_n =q^n −q^(′n) =q^n {1−((q^′ /q))^n } (q^′ /q) =((1−(√5))/(1+(√5))) =1−((2(√5))/(1+(√5))) ⇒∣(q^′ /q)∣<1 ⇒(√5)u_n ∼q^n and (√5)u_(n+1) ∼q^(n+1) ⇒ (u_(n+1) /u_n )∼ q ⇒lim_(n→+∞) (u_(n+1) /u_n ) =((1+(√5))/2) (golden number ϕ)

$${let}\:{q}=\frac{\mathrm{1}+\sqrt{\mathrm{5}}}{\mathrm{2}}\:{and}\:{q}^{'} =\frac{\mathrm{1}−\sqrt{\mathrm{5}}}{\mathrm{2}}\:\Rightarrow\sqrt{\mathrm{5}}{u}_{{n}} ={q}^{{n}} −{q}^{'{n}} ={q}^{{n}} \left\{\mathrm{1}−\left(\frac{{q}^{'} }{{q}}\right)^{{n}} \right\} \\ $$$$\frac{{q}^{'} }{{q}}\:=\frac{\mathrm{1}−\sqrt{\mathrm{5}}}{\mathrm{1}+\sqrt{\mathrm{5}}}\:=\mathrm{1}−\frac{\mathrm{2}\sqrt{\mathrm{5}}}{\mathrm{1}+\sqrt{\mathrm{5}}}\:\Rightarrow\mid\frac{{q}^{'} }{{q}}\mid<\mathrm{1}\:\Rightarrow\sqrt{\mathrm{5}}{u}_{{n}} \sim{q}^{{n}} \:{and} \\ $$$$\sqrt{\mathrm{5}}{u}_{{n}+\mathrm{1}} \sim{q}^{{n}+\mathrm{1}} \:\Rightarrow\:\frac{{u}_{{n}+\mathrm{1}} }{{u}_{{n}} }\sim\:{q}\:\Rightarrow{lim}_{{n}\rightarrow+\infty} \frac{{u}_{{n}+\mathrm{1}} }{{u}_{{n}} }\:=\frac{\mathrm{1}+\sqrt{\mathrm{5}}}{\mathrm{2}}\:\:\left({golden}\:{number}\:\varphi\right) \\ $$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com