
Question Number 218866 by Nicholas666 last updated on 16/Apr/25
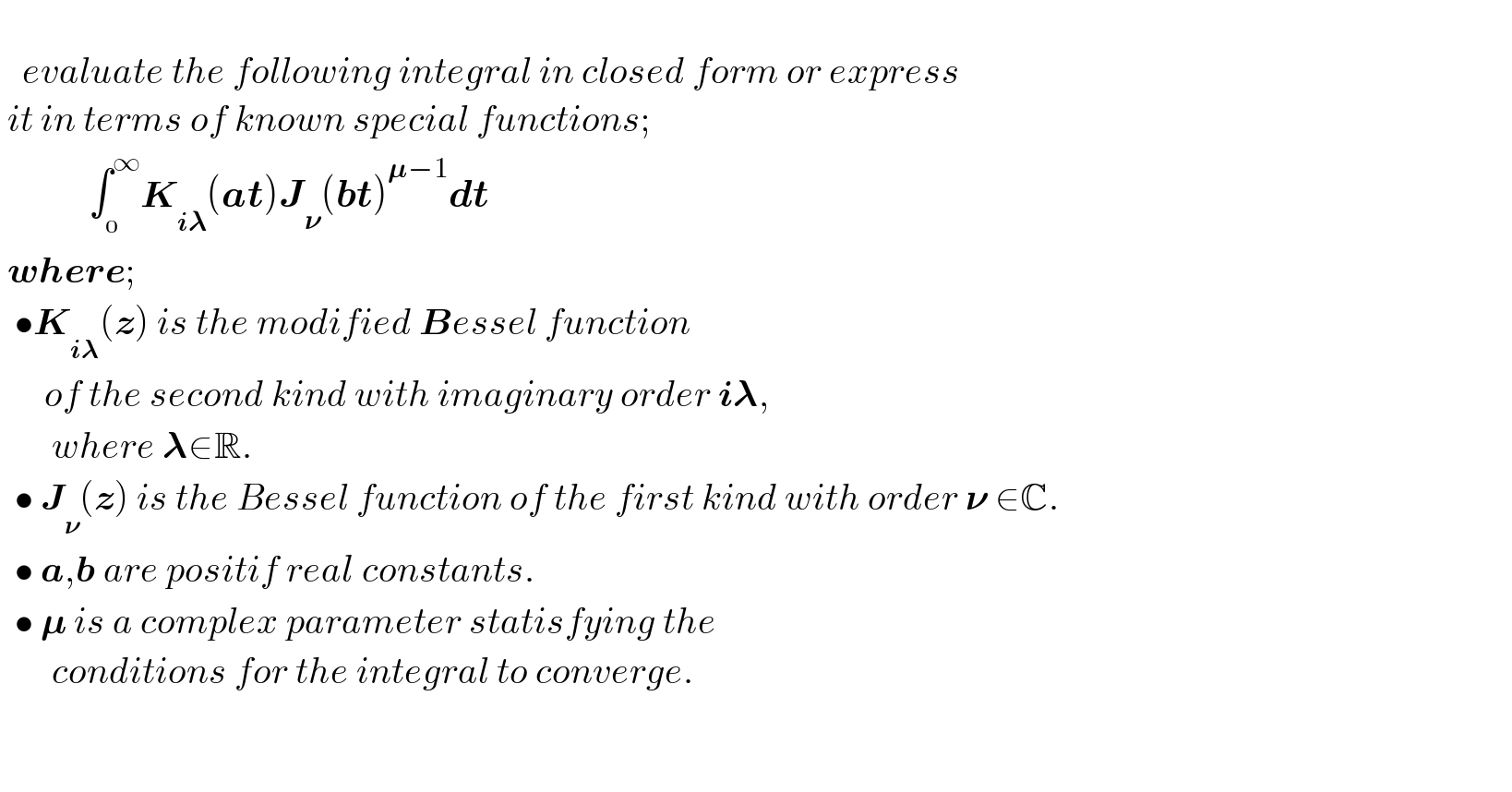
$$ \\ $$$$\:\:\:{evaluate}\:{the}\:{following}\:{integral}\:{in}\:{closed}\:{form}\:{or}\:{express} \\ $$$$\:{it}\:{in}\:{terms}\:{of}\:{known}\:{special}\:{functions};\:\:\: \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\int_{ } ^{\infty} \boldsymbol{{K}}_{\boldsymbol{{i}\lambda}} \left(\boldsymbol{{at}}\right)\boldsymbol{{J}}_{\boldsymbol{\nu}} \left(\boldsymbol{{bt}}\right)^{\boldsymbol{\mu}−\mathrm{1}} \boldsymbol{{dt}} \\ $$$$\:\boldsymbol{{where}}; \\ $$$$\:\:\bullet\boldsymbol{{K}}_{\boldsymbol{{i}\lambda}} \left(\boldsymbol{{z}}\right)\:{is}\:{the}\:{modified}\:\boldsymbol{{B}}{essel}\:{function}\: \\ $$$$\:\:\:\:\:\:{of}\:{the}\:{second}\:{kind}\:{with}\:{imaginary}\:{order}\:\boldsymbol{{i}\lambda}, \\ $$$$\:\:\:\:\:\:\:{where}\:\boldsymbol{\lambda}\in\mathbb{R}. \\ $$$$\:\:\bullet\:\boldsymbol{{J}}_{\boldsymbol{\nu}} \left(\boldsymbol{{z}}\right)\:{is}\:{the}\:{Bessel}\:{function}\:{of}\:{the}\:{first}\:{kind}\:{with}\:{order}\:\boldsymbol{\nu}\:\in\mathbb{C}.\:\:\:\:\: \\ $$$$\:\:\bullet\:\boldsymbol{{a}},\boldsymbol{{b}}\:{are}\:{positif}\:{real}\:{constants}. \\ $$$$\:\:\bullet\:\boldsymbol{\mu}\:{is}\:{a}\:{complex}\:{parameter}\:{statisfying}\:{the}\:\:\: \\ $$$$\:\:\:\:\:\:\:{conditions}\:{for}\:{the}\:{integral}\:{to}\:{converge}.\:\:\:\: \\ $$$$ \\ $$$$\:\:\: \\ $$
