Question and Answers Forum

All Questions      Topic List

Trigonometry Questions

Previous in All Question      Next in All Question      

Previous in Trigonometry      Next in Trigonometry      

Question Number 40640 by upadhyayrakhi20@gmail.com last updated on 25/Jul/18

evaluate sin 72^.

$${evaluate} \\ $$$$\mathrm{sin}\:\mathrm{72}\:^{.} \\ $$

Answered by tanmay.chaudhury50@gmail.com last updated on 25/Jul/18

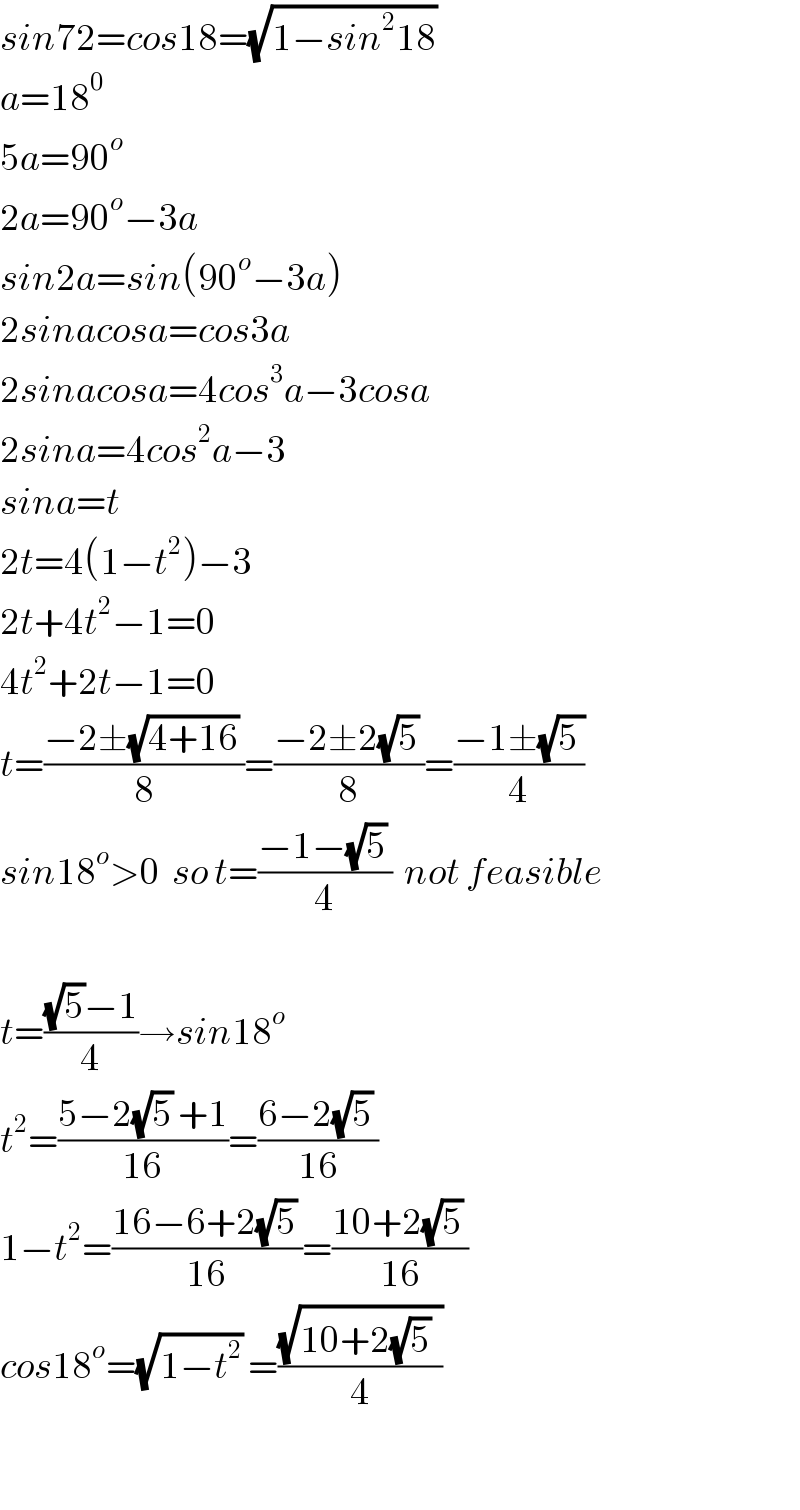
sin72=cos18=(√(1−sin^2 18)) a=18^0 5a=90^o 2a=90^o −3a sin2a=sin(90^o −3a) 2sinacosa=cos3a 2sinacosa=4cos^3 a−3cosa 2sina=4cos^2 a−3 sina=t 2t=4(1−t^2 )−3 2t+4t^2 −1=0 4t^2 +2t−1=0 t=((−2±(√(4+16)) )/8)=((−2±2(√5) )/8)=((−1±(√(5 )))/4) sin18^o >0 so t=((−1−(√5) )/4) not feasible t=(((√5)−1)/4)→sin18^o t^2 =((5−2(√5) +1)/(16))=((6−2(√5) )/(16)) 1−t^2 =((16−6+2(√5) )/(16))=((10+2(√5) )/(16)) cos18^o =(√(1−t^2 )) =((√(10+2(√5) ))/4)

$${sin}\mathrm{72}={cos}\mathrm{18}=\sqrt{\mathrm{1}−{sin}^{\mathrm{2}} \mathrm{18}}\: \\ $$$${a}=\mathrm{18}^{\mathrm{0}} \\ $$$$\mathrm{5}{a}=\mathrm{90}^{{o}} \\ $$$$\mathrm{2}{a}=\mathrm{90}^{{o}} −\mathrm{3}{a} \\ $$$${sin}\mathrm{2}{a}={sin}\left(\mathrm{90}^{{o}} −\mathrm{3}{a}\right) \\ $$$$\mathrm{2}{sinacosa}={cos}\mathrm{3}{a} \\ $$$$\mathrm{2}{sinacosa}=\mathrm{4}{cos}^{\mathrm{3}} {a}−\mathrm{3}{cosa} \\ $$$$\mathrm{2}{sina}=\mathrm{4}{cos}^{\mathrm{2}} {a}−\mathrm{3} \\ $$$${sina}={t} \\ $$$$\mathrm{2}{t}=\mathrm{4}\left(\mathrm{1}−{t}^{\mathrm{2}} \right)−\mathrm{3} \\ $$$$\mathrm{2}{t}+\mathrm{4}{t}^{\mathrm{2}} −\mathrm{1}=\mathrm{0} \\ $$$$\mathrm{4}{t}^{\mathrm{2}} +\mathrm{2}{t}−\mathrm{1}=\mathrm{0} \\ $$$${t}=\frac{−\mathrm{2}\pm\sqrt{\mathrm{4}+\mathrm{16}}\:}{\mathrm{8}}=\frac{−\mathrm{2}\pm\mathrm{2}\sqrt{\mathrm{5}}\:}{\mathrm{8}}=\frac{−\mathrm{1}\pm\sqrt{\mathrm{5}\:}}{\mathrm{4}} \\ $$$${sin}\mathrm{18}^{{o}} >\mathrm{0}\:\:{so}\:{t}=\frac{−\mathrm{1}−\sqrt{\mathrm{5}}\:}{\mathrm{4}}\:\:{not}\:{feasible} \\ $$$$ \\ $$$${t}=\frac{\sqrt{\mathrm{5}}−\mathrm{1}}{\mathrm{4}}\rightarrow{sin}\mathrm{18}^{{o}} \\ $$$${t}^{\mathrm{2}} =\frac{\mathrm{5}−\mathrm{2}\sqrt{\mathrm{5}}\:+\mathrm{1}}{\mathrm{16}}=\frac{\mathrm{6}−\mathrm{2}\sqrt{\mathrm{5}}\:}{\mathrm{16}} \\ $$$$\mathrm{1}−{t}^{\mathrm{2}} =\frac{\mathrm{16}−\mathrm{6}+\mathrm{2}\sqrt{\mathrm{5}}\:}{\mathrm{16}}=\frac{\mathrm{10}+\mathrm{2}\sqrt{\mathrm{5}}\:}{\mathrm{16}} \\ $$$${cos}\mathrm{18}^{{o}} =\sqrt{\mathrm{1}−{t}^{\mathrm{2}} }\:=\frac{\sqrt{\mathrm{10}+\mathrm{2}\sqrt{\mathrm{5}}\:\:}}{\mathrm{4}} \\ $$$$ \\ $$

Commented by Tawa1 last updated on 25/Jul/18

great ..

$$\mathrm{great}\:.. \\ $$

Commented by tanmay.chaudhury50@gmail.com last updated on 25/Jul/18

thank you sir...

$${thank}\:{you}\:{sir}... \\ $$

Commented by upadhyayrakhi20@gmail.com last updated on 25/Jul/18

thnx

$$\mathrm{thnx} \\ $$

Commented by tanmay.chaudhury50@gmail.com last updated on 25/Jul/18

its ok...

$${its}\:{ok}... \\ $$

Answered by MJS last updated on 25/Jul/18

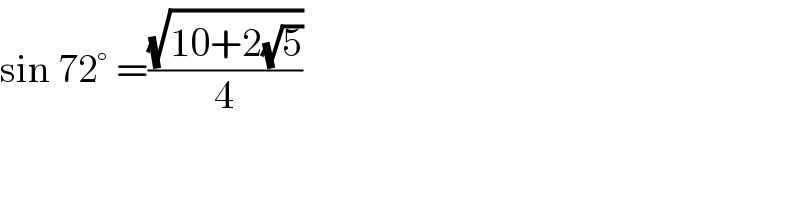
sin 72° =((√(10+2(√5)))/4)

$$\mathrm{sin}\:\mathrm{72}°\:=\frac{\sqrt{\mathrm{10}+\mathrm{2}\sqrt{\mathrm{5}}}}{\mathrm{4}} \\ $$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com