
Question Number 26909 by sorour87 last updated on 31/Dec/17
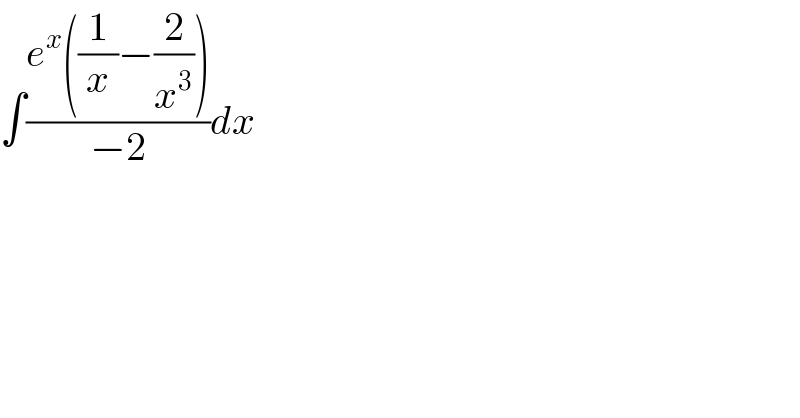
$$\int\frac{{e}^{{x}} \left(\frac{\mathrm{1}}{{x}}−\frac{\mathrm{2}}{{x}^{\mathrm{3}} }\right)}{−\mathrm{2}}{dx} \\ $$
Answered by prakash jain last updated on 31/Dec/17
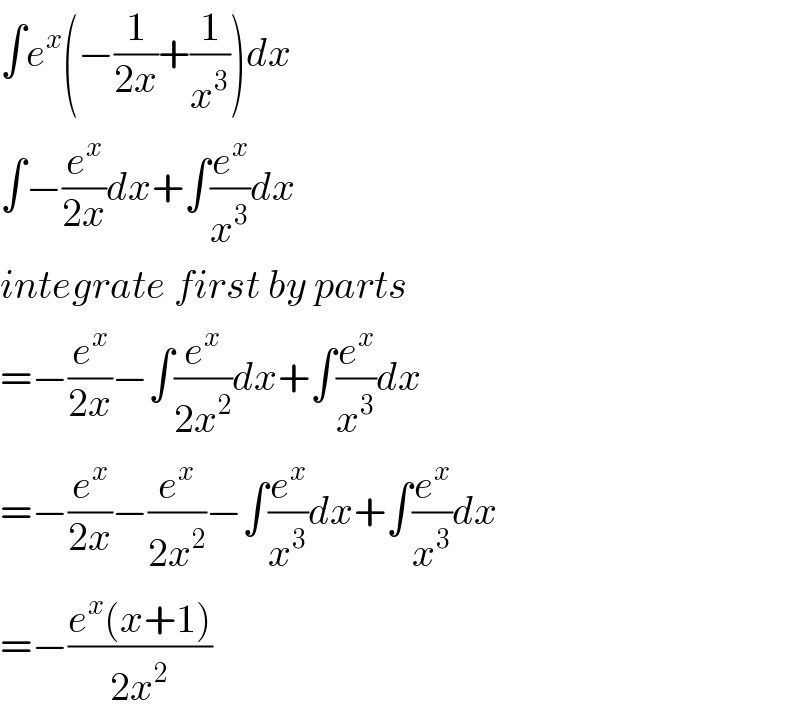
$$\int{e}^{{x}} \left(−\frac{\mathrm{1}}{\mathrm{2}{x}}+\frac{\mathrm{1}}{{x}^{\mathrm{3}} }\right){dx} \\ $$$$\int−\frac{{e}^{{x}} }{\mathrm{2}{x}}{dx}+\int\frac{{e}^{{x}} }{{x}^{\mathrm{3}} }{dx} \\ $$$${integrate}\:{first}\:{by}\:{parts} \\ $$$$=−\frac{{e}^{{x}} }{\mathrm{2}{x}}−\int\frac{{e}^{{x}} }{\mathrm{2}{x}^{\mathrm{2}} }{dx}+\int\frac{{e}^{{x}} }{{x}^{\mathrm{3}} }{dx} \\ $$$$=−\frac{{e}^{{x}} }{\mathrm{2}{x}}−\frac{{e}^{{x}} }{\mathrm{2}{x}^{\mathrm{2}} }−\int\frac{{e}^{{x}} }{{x}^{\mathrm{3}} }{dx}+\int\frac{{e}^{{x}} }{{x}^{\mathrm{3}} }{dx} \\ $$$$=−\frac{{e}^{{x}} \left({x}+\mathrm{1}\right)}{\mathrm{2}{x}^{\mathrm{2}} } \\ $$
