Question and Answers Forum

All Questions      Topic List

Integration Questions

Previous in All Question      Next in All Question      

Previous in Integration      Next in Integration      

Question Number 24828 by moxhix last updated on 27/Nov/17

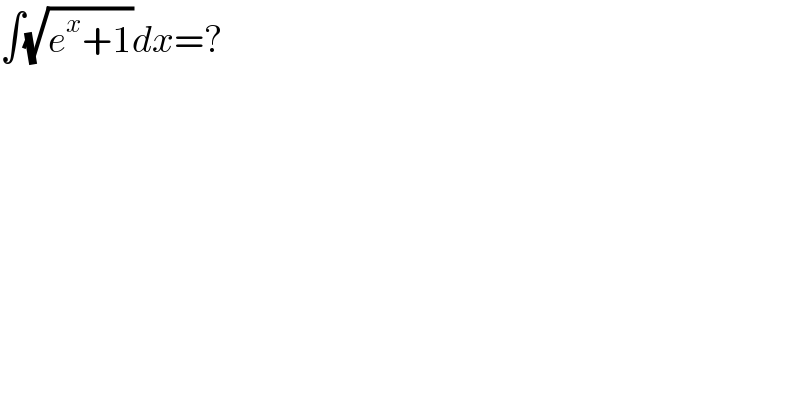
∫(√(e^x +1))dx=?

$$\int\sqrt{{e}^{{x}} +\mathrm{1}}{dx}=? \\ $$

Commented by mrW1 last updated on 27/Nov/17

see Q24670

$${see}\:{Q}\mathrm{24670} \\ $$

Commented by moxhix last updated on 27/Nov/17

Thank you

$${Thank}\:{you} \\ $$

Commented by maxmathsup by imad last updated on 24/May/19

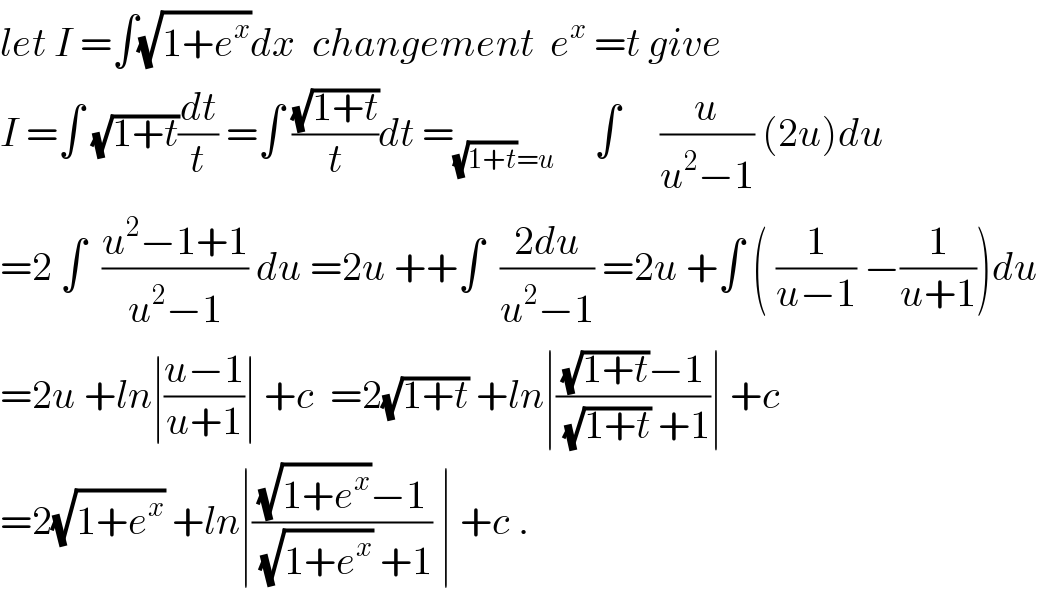
let I =∫(√(1+e^x ))dx changement e^x =t give I =∫ (√(1+t))(dt/t) =∫ ((√(1+t))/t)dt =_((√(1+t))=u) ∫ (u/(u^2 −1)) (2u)du =2 ∫ ((u^2 −1+1)/(u^2 −1)) du =2u ++∫ ((2du)/(u^2 −1)) =2u +∫ ( (1/(u−1)) −(1/(u+1)))du =2u +ln∣((u−1)/(u+1))∣ +c =2(√(1+t)) +ln∣(((√(1+t))−1)/((√(1+t)) +1))∣ +c =2(√(1+e^x )) +ln∣(((√(1+e^x ))−1)/((√(1+e^x )) +1)) ∣ +c .

$${let}\:{I}\:=\int\sqrt{\mathrm{1}+{e}^{{x}} }{dx}\:\:{changement}\:\:{e}^{{x}} \:={t}\:{give} \\ $$$${I}\:=\int\:\sqrt{\mathrm{1}+{t}}\frac{{dt}}{{t}}\:=\int\:\frac{\sqrt{\mathrm{1}+{t}}}{{t}}{dt}\:=_{\sqrt{\mathrm{1}+{t}}={u}} \:\:\:\:\:\int\:\:\:\:\:\frac{{u}}{{u}^{\mathrm{2}} −\mathrm{1}}\:\left(\mathrm{2}{u}\right){du} \\ $$$$=\mathrm{2}\:\int\:\:\frac{{u}^{\mathrm{2}} −\mathrm{1}+\mathrm{1}}{{u}^{\mathrm{2}} −\mathrm{1}}\:{du}\:=\mathrm{2}{u}\:++\int\:\:\frac{\mathrm{2}{du}}{{u}^{\mathrm{2}} −\mathrm{1}}\:=\mathrm{2}{u}\:+\int\:\left(\:\frac{\mathrm{1}}{{u}−\mathrm{1}}\:−\frac{\mathrm{1}}{{u}+\mathrm{1}}\right){du} \\ $$$$=\mathrm{2}{u}\:+{ln}\mid\frac{{u}−\mathrm{1}}{{u}+\mathrm{1}}\mid\:+{c}\:\:=\mathrm{2}\sqrt{\mathrm{1}+{t}}\:+{ln}\mid\frac{\sqrt{\mathrm{1}+{t}}−\mathrm{1}}{\sqrt{\mathrm{1}+{t}}\:+\mathrm{1}}\mid\:+{c} \\ $$$$=\mathrm{2}\sqrt{\mathrm{1}+{e}^{{x}} }\:+{ln}\mid\frac{\sqrt{\mathrm{1}+{e}^{{x}} }−\mathrm{1}}{\sqrt{\mathrm{1}+{e}^{{x}} }\:+\mathrm{1}}\:\mid\:+{c}\:. \\ $$

Answered by Eng.Firas last updated on 27/Nov/17

let e^x =tan^2 θ e^x dx=2tanθ sec^2 θ dθ dx=2((sec^2 θ)/(tanθ)) dθ ∫(√(tan^2 θ+1 ))((sec^2 θ)/(tanθ))dθ ∫secθ ((1+tan^2 θ)/(tanθ))dθ ∫secθ cotθ dθ +∫secθ tanθ dθ ∫ cscθ dθ +∫secθ tanθ dθ =−ln∣cscθ+cotθ∣+secθ+C =−ln∣((√(1+e^x ))/(√e^x ))+(1/(√e^x ))∣+(√(e^x +1))+C

$${let}\:{e}^{{x}} ={tan}^{\mathrm{2}} \theta \\ $$$$\:{e}^{{x}} {dx}=\mathrm{2}{tan}\theta\:{sec}^{\mathrm{2}} \theta\:{d}\theta \\ $$$${dx}=\mathrm{2}\frac{{sec}^{\mathrm{2}} \theta}{{tan}\theta}\:{d}\theta \\ $$$$\int\sqrt{{tan}^{\mathrm{2}} \theta+\mathrm{1}\:}\frac{{sec}^{\mathrm{2}} \theta}{{tan}\theta}{d}\theta \\ $$$$\int{sec}\theta\:\frac{\mathrm{1}+{tan}^{\mathrm{2}} \theta}{{tan}\theta}{d}\theta \\ $$$$\int{sec}\theta\:{cot}\theta\:{d}\theta\:+\int{sec}\theta\:{tan}\theta\:{d}\theta \\ $$$$\int\:{csc}\theta\:{d}\theta\:+\int{sec}\theta\:{tan}\theta\:{d}\theta \\ $$$$=−{ln}\mid{csc}\theta+{cot}\theta\mid+{sec}\theta+{C} \\ $$$$=−{ln}\mid\frac{\sqrt{\mathrm{1}+{e}^{{x}} }}{\sqrt{{e}^{{x}} }}+\frac{\mathrm{1}}{\sqrt{{e}^{{x}} }}\mid+\sqrt{{e}^{{x}} +\mathrm{1}}+{C} \\ $$$$ \\ $$$$ \\ $$

Commented by moxhix last updated on 27/Nov/17

Thank you

$${Thank}\:{you} \\ $$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com