Question and Answers Forum

All Questions      Topic List

Integration Questions

Previous in All Question      Next in All Question      

Previous in Integration      Next in Integration      

Question Number 167586 by cortano1 last updated on 20/Mar/22

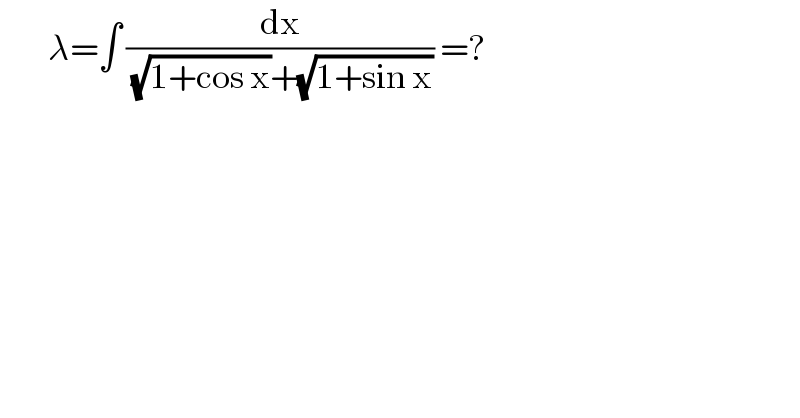
λ=∫ (dx/( (√(1+cos x))+(√(1+sin x)))) =?

$$\:\:\:\:\:\:\:\:\lambda=\int\:\frac{\mathrm{dx}}{\:\sqrt{\mathrm{1}+\mathrm{cos}\:\mathrm{x}}+\sqrt{\mathrm{1}+\mathrm{sin}\:\mathrm{x}}}\:=? \\ $$

Commented by MJS_new last updated on 20/Mar/22

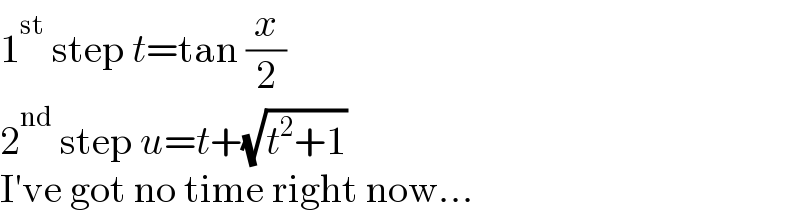
1^(st) step t=tan (x/2) 2^(nd) step u=t+(√(t^2 +1)) I′ve got no time right now...

$$\mathrm{1}^{\mathrm{st}} \:\mathrm{step}\:{t}=\mathrm{tan}\:\frac{{x}}{\mathrm{2}} \\ $$$$\mathrm{2}^{\mathrm{nd}} \:\mathrm{step}\:{u}={t}+\sqrt{{t}^{\mathrm{2}} +\mathrm{1}} \\ $$$$\mathrm{I}'\mathrm{ve}\:\mathrm{got}\:\mathrm{no}\:\mathrm{time}\:\mathrm{right}\:\mathrm{now}... \\ $$

Answered by greogoury55 last updated on 20/Mar/22

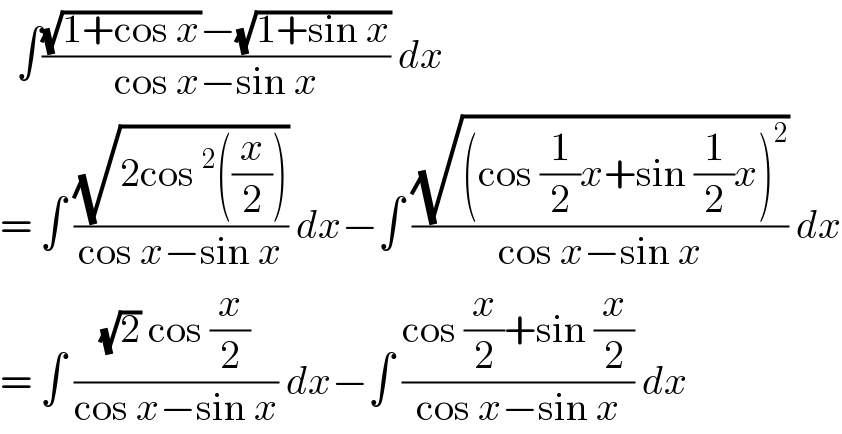
∫(((√(1+cos x))−(√(1+sin x)))/(cos x−sin x)) dx = ∫ ((√(2cos^2 ((x/2))))/(cos x−sin x)) dx−∫ ((√((cos (1/2)x+sin (1/2)x)^2 ))/(cos x−sin x)) dx = ∫ (((√2) cos (x/2))/(cos x−sin x)) dx−∫ ((cos (x/2)+sin (x/2))/(cos x−sin x)) dx

$$\:\:\int\frac{\sqrt{\mathrm{1}+\mathrm{cos}\:{x}}−\sqrt{\mathrm{1}+\mathrm{sin}\:{x}}}{\mathrm{cos}\:{x}−\mathrm{sin}\:{x}}\:{dx}\: \\ $$$$=\:\int\:\frac{\sqrt{\mathrm{2cos}\:^{\mathrm{2}} \left(\frac{{x}}{\mathrm{2}}\right)}}{\mathrm{cos}\:{x}−\mathrm{sin}\:{x}}\:{dx}−\int\:\frac{\sqrt{\left(\mathrm{cos}\:\frac{\mathrm{1}}{\mathrm{2}}{x}+\mathrm{sin}\:\frac{\mathrm{1}}{\mathrm{2}}{x}\right)^{\mathrm{2}} }}{\mathrm{cos}\:{x}−\mathrm{sin}\:{x}}\:{dx} \\ $$$$=\:\int\:\frac{\sqrt{\mathrm{2}}\:\mathrm{cos}\:\frac{{x}}{\mathrm{2}}}{\mathrm{cos}\:{x}−\mathrm{sin}\:{x}}\:{dx}−\int\:\frac{\mathrm{cos}\:\frac{{x}}{\mathrm{2}}+\mathrm{sin}\:\frac{{x}}{\mathrm{2}}}{\mathrm{cos}\:{x}−\mathrm{sin}\:{x}}\:{dx}\: \\ $$

Answered by MJS_new last updated on 20/Mar/22

∫(dx/( (√(1+cos x))+(√(1+sin x))))= [t=tan (x/2) → dx=((2dt)/(t^2 +1))] =2∫(dt/((t+1+(√2))(√(t^2 +1))))= [u=t+(√(t^2 +1)) → dt=((√(t^2 +1))/u)du] =4∫(du/(u^2 +2(1+(√2))u−1))= =(√(2−(√2)))ln ((u+1+(√2)−(√(4+2(√2))))/(u+1+(√2)+(√(4+2(√2))))) =...

$$\int\frac{{dx}}{\:\sqrt{\mathrm{1}+\mathrm{cos}\:{x}}+\sqrt{\mathrm{1}+\mathrm{sin}\:{x}}}= \\ $$$$\:\:\:\:\:\left[{t}=\mathrm{tan}\:\frac{{x}}{\mathrm{2}}\:\rightarrow\:{dx}=\frac{\mathrm{2}{dt}}{{t}^{\mathrm{2}} +\mathrm{1}}\right] \\ $$$$=\mathrm{2}\int\frac{{dt}}{\left({t}+\mathrm{1}+\sqrt{\mathrm{2}}\right)\sqrt{{t}^{\mathrm{2}} +\mathrm{1}}}= \\ $$$$\:\:\:\:\:\left[{u}={t}+\sqrt{{t}^{\mathrm{2}} +\mathrm{1}}\:\rightarrow\:{dt}=\frac{\sqrt{{t}^{\mathrm{2}} +\mathrm{1}}}{{u}}{du}\right] \\ $$$$=\mathrm{4}\int\frac{{du}}{{u}^{\mathrm{2}} +\mathrm{2}\left(\mathrm{1}+\sqrt{\mathrm{2}}\right){u}−\mathrm{1}}= \\ $$$$=\sqrt{\mathrm{2}−\sqrt{\mathrm{2}}}\mathrm{ln}\:\frac{{u}+\mathrm{1}+\sqrt{\mathrm{2}}−\sqrt{\mathrm{4}+\mathrm{2}\sqrt{\mathrm{2}}}}{{u}+\mathrm{1}+\sqrt{\mathrm{2}}+\sqrt{\mathrm{4}+\mathrm{2}\sqrt{\mathrm{2}}}}\:=... \\ $$

Commented by peter frank last updated on 21/Mar/22

thank you

$$\mathrm{thank}\:\mathrm{you} \\ $$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com