
Question Number 62833 by aliesam last updated on 25/Jun/19
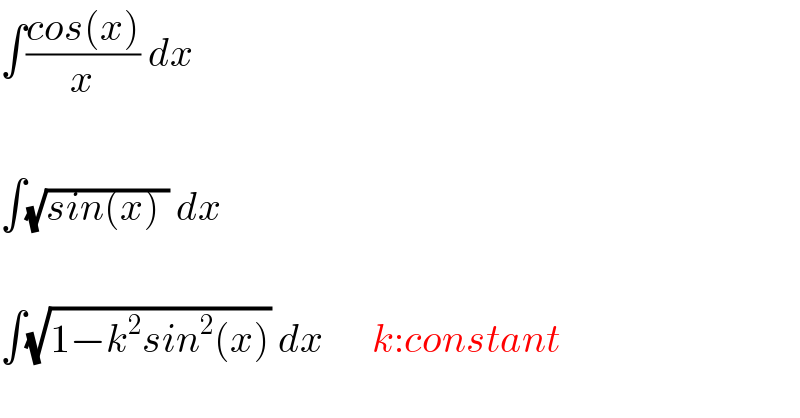
$$\int\frac{{cos}\left({x}\right)}{{x}}\:{dx} \\ $$$$ \\ $$$$\int\sqrt{{sin}\left({x}\right)\:}\:{dx} \\ $$$$ \\ $$$$\int\sqrt{\mathrm{1}−{k}^{\mathrm{2}} {sin}^{\mathrm{2}} \left({x}\right)}\:{dx}\:\:\:\:\:\:{k}:{constant} \\ $$
Commented by mathmax by abdo last updated on 26/Jun/19
![x→((cosx)/x) is not integrable at x_0 =0 let put A(x) =∫_1 ^x ((cost)/t) dt and let f(u) =∫_1 ^x ((cost)/t) e^(−ut) dt defined on[0,+∞[ we have f^′ (u) = − ∫_1 ^x cost e^(−ut) dt =−Re( ∫_1 ^x e^(it−ut) dt) ∫_1 ^x e^((−u+i)t) dt =[(1/(−u+i)) e^((−u+i)t) ]_1 ^x =−(1/(u−i)){ e^((−u+i)x) −e^(−u+i) } =−(e^(−u) /(u−i)){ cosx +isinx −cos1 −isin1} =−(((u+i) e^(−u) )/(1+u^2 )){ cosx −cos1 +i(sinx −sin1)} =−(e^(−u) /(1+u^2 )){ u(cosx−cos1)+iu(sinx−sin1)+i(cosx−cos1)−(sinx−sin1)} ⇒f^′ (u) =−(e^(−u) /(1+u^2 )){ u(cosx−cos1)−sinx+sin1}⇒ f(u) =−∫_1 ^u ((t(cosx−cos1)−sinx +sin1)/(1+t^2 )) e^(−t) dt +λ f(u) =(cos1−cosx) ∫_1 ^u ((t e^(−t) )/(1+t^2 ))dt +(sin1−sinx) ∫_1 ^u (e^(−t) /(1+t^2 ))dt +λ λ =f(1) and A(x) =lim_(u→0) f(u) =(cos1−cosx) ∫_1 ^0 ((t e^(−t) )/(1+t^2 ))dt +(sin1 −sinx)∫_1 ^0 (e^(−t) /(1+t^2 )) dt +f(1) =(cosx−cos1)∫_0 ^1 ((t e^(−t) )/(1+t^2 ))dt +(sinx−sin1) ∫_0 ^1 (e^(−t) /(1+t^2 )) dt +f(1) ....be continued...](Q62853.png)
$${x}\rightarrow\frac{{cosx}}{{x}}\:{is}\:{not}\:{integrable}\:{at}\:{x}_{\mathrm{0}} =\mathrm{0}\:{let}\:{put}\:\:{A}\left({x}\right)\:=\int_{\mathrm{1}} ^{{x}} \:\frac{{cost}}{{t}}\:{dt}\:\:{and}\:{let} \\ $$$${f}\left({u}\right)\:=\int_{\mathrm{1}} ^{{x}} \:\frac{{cost}}{{t}}\:{e}^{−{ut}} \:{dt}\:\:\:\:{defined}\:{on}\left[\mathrm{0},+\infty\left[\right.\right. \\ $$$${we}\:{have}\:{f}^{'} \left({u}\right)\:=\:−\:\int_{\mathrm{1}} ^{{x}} \:{cost}\:{e}^{−{ut}} {dt}\:=−{Re}\left(\:\int_{\mathrm{1}} ^{{x}} \:{e}^{{it}−{ut}} {dt}\right) \\ $$$$\int_{\mathrm{1}} ^{{x}} \:\:{e}^{\left(−{u}+{i}\right){t}} {dt}\:=\left[\frac{\mathrm{1}}{−{u}+{i}}\:{e}^{\left(−{u}+{i}\right){t}} \right]_{\mathrm{1}} ^{{x}} \:=−\frac{\mathrm{1}}{{u}−{i}}\left\{\:{e}^{\left(−{u}+{i}\right){x}} −{e}^{−{u}+{i}} \right\} \\ $$$$=−\frac{{e}^{−{u}} }{{u}−{i}}\left\{\:{cosx}\:+{isinx}\:−{cos}\mathrm{1}\:−{isin}\mathrm{1}\right\} \\ $$$$=−\frac{\left({u}+{i}\right)\:{e}^{−{u}} }{\mathrm{1}+{u}^{\mathrm{2}} }\left\{\:{cosx}\:−{cos}\mathrm{1}\:+{i}\left({sinx}\:−{sin}\mathrm{1}\right)\right\} \\ $$$$=−\frac{{e}^{−{u}} }{\mathrm{1}+{u}^{\mathrm{2}} }\left\{\:{u}\left({cosx}−{cos}\mathrm{1}\right)+{iu}\left({sinx}−{sin}\mathrm{1}\right)+{i}\left({cosx}−{cos}\mathrm{1}\right)−\left({sinx}−{sin}\mathrm{1}\right)\right\} \\ $$$$\Rightarrow{f}^{'} \left({u}\right)\:=−\frac{{e}^{−{u}} }{\mathrm{1}+{u}^{\mathrm{2}} }\left\{\:{u}\left({cosx}−{cos}\mathrm{1}\right)−{sinx}+{sin}\mathrm{1}\right\}\Rightarrow \\ $$$${f}\left({u}\right)\:=−\int_{\mathrm{1}} ^{{u}} \:\:\frac{{t}\left({cosx}−{cos}\mathrm{1}\right)−{sinx}\:+{sin}\mathrm{1}}{\mathrm{1}+{t}^{\mathrm{2}} }\:{e}^{−{t}} \:{dt}\:\:+\lambda \\ $$$${f}\left({u}\right)\:=\left({cos}\mathrm{1}−{cosx}\right)\:\int_{\mathrm{1}} ^{{u}} \:\:\:\frac{{t}\:{e}^{−{t}} }{\mathrm{1}+{t}^{\mathrm{2}} }{dt}\:\:+\left({sin}\mathrm{1}−{sinx}\right)\:\int_{\mathrm{1}} ^{{u}} \:\:\frac{{e}^{−{t}} }{\mathrm{1}+{t}^{\mathrm{2}} }{dt}\:+\lambda \\ $$$$\lambda\:={f}\left(\mathrm{1}\right)\:\:{and}\:{A}\left({x}\right)\:={lim}_{{u}\rightarrow\mathrm{0}} {f}\left({u}\right) \\ $$$$=\left({cos}\mathrm{1}−{cosx}\right)\:\int_{\mathrm{1}} ^{\mathrm{0}} \:\:\frac{{t}\:{e}^{−{t}} }{\mathrm{1}+{t}^{\mathrm{2}} }{dt}\:+\left({sin}\mathrm{1}\:−{sinx}\right)\int_{\mathrm{1}} ^{\mathrm{0}} \:\:\frac{{e}^{−{t}} }{\mathrm{1}+{t}^{\mathrm{2}} }\:{dt}\:+{f}\left(\mathrm{1}\right) \\ $$$$=\left({cosx}−{cos}\mathrm{1}\right)\int_{\mathrm{0}} ^{\mathrm{1}} \:\:\frac{{t}\:{e}^{−{t}} }{\mathrm{1}+{t}^{\mathrm{2}} }{dt}\:+\left({sinx}−{sin}\mathrm{1}\right)\:\int_{\mathrm{0}} ^{\mathrm{1}} \:\frac{{e}^{−{t}} }{\mathrm{1}+{t}^{\mathrm{2}} }\:{dt}\:\:+{f}\left(\mathrm{1}\right) \\ $$$$....{be}\:{continued}... \\ $$
Commented by mathmax by abdo last updated on 26/Jun/19
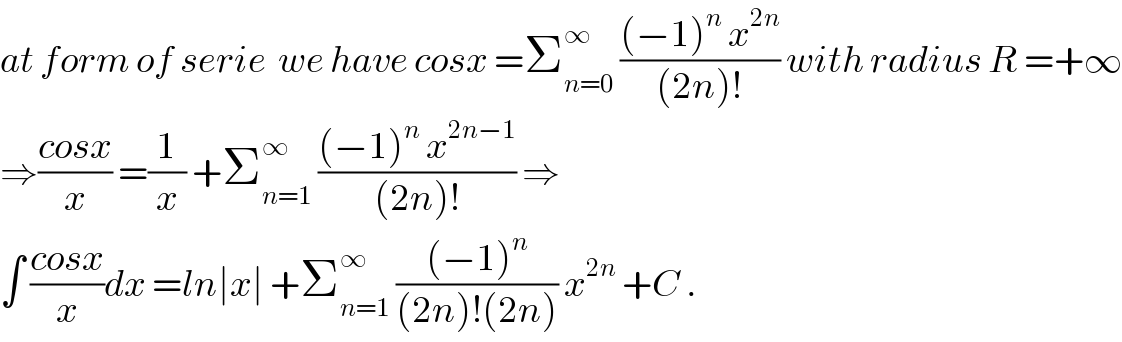
$${at}\:{form}\:{of}\:{serie}\:\:{we}\:{have}\:{cosx}\:=\sum_{{n}=\mathrm{0}} ^{\infty} \:\frac{\left(−\mathrm{1}\right)^{{n}} \:{x}^{\mathrm{2}{n}} }{\left(\mathrm{2}{n}\right)!}\:{with}\:{radius}\:{R}\:=+\infty \\ $$$$\Rightarrow\frac{{cosx}}{{x}}\:=\frac{\mathrm{1}}{{x}}\:+\sum_{{n}=\mathrm{1}} ^{\infty} \:\frac{\left(−\mathrm{1}\right)^{{n}} \:{x}^{\mathrm{2}{n}−\mathrm{1}} }{\left(\mathrm{2}{n}\right)!}\:\Rightarrow \\ $$$$\int\:\frac{{cosx}}{{x}}{dx}\:={ln}\mid{x}\mid\:+\sum_{{n}=\mathrm{1}} ^{\infty} \:\frac{\left(−\mathrm{1}\right)^{{n}} }{\left(\mathrm{2}{n}\right)!\left(\mathrm{2}{n}\right)}\:{x}^{\mathrm{2}{n}} \:+{C}\:. \\ $$
