Question and Answers Forum

All Questions      Topic List

Integration Questions

Previous in All Question      Next in All Question      

Previous in Integration      Next in Integration      

Question Number 88586 by M±th+et£s last updated on 11/Apr/20

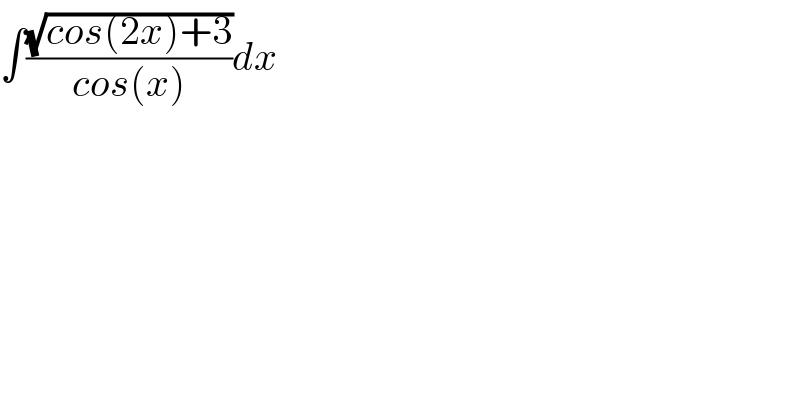
∫((√(cos(2x)+3))/(cos(x)))dx

$$\int\frac{\sqrt{{cos}\left(\mathrm{2}{x}\right)+\mathrm{3}}}{{cos}\left({x}\right)}{dx} \\ $$

Answered by TANMAY PANACEA. last updated on 11/Apr/20

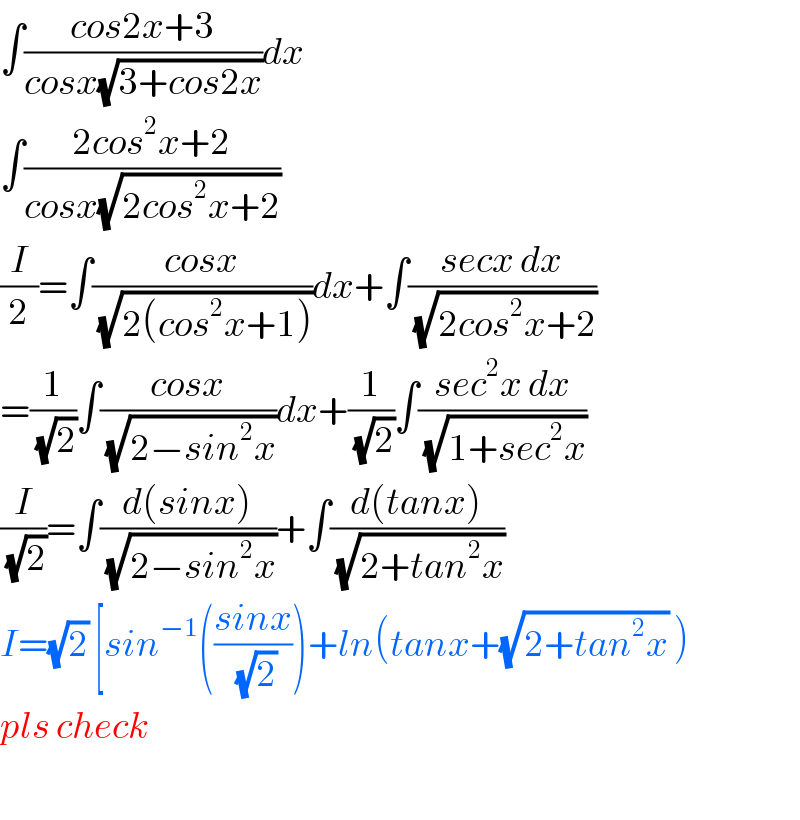
∫((cos2x+3)/(cosx(√(3+cos2x))))dx ∫((2cos^2 x+2)/(cosx(√(2cos^2 x+2)))) (I/2)=∫((cosx)/(√(2(cos^2 x+1))))dx+∫((secx dx)/(√(2cos^2 x+2))) =(1/(√2))∫((cosx)/(√(2−sin^2 x)))dx+(1/(√2))∫((sec^2 x dx)/(√(1+sec^2 x))) (I/(√2))=∫((d(sinx))/(√(2−sin^2 x)))+∫((d(tanx))/(√(2+tan^2 x))) I=(√2) [sin^(−1) (((sinx)/(√2)))+ln(tanx+(√(2+tan^2 x)) ) pls check

$$\int\frac{{cos}\mathrm{2}{x}+\mathrm{3}}{{cosx}\sqrt{\mathrm{3}+{cos}\mathrm{2}{x}}}{dx} \\ $$$$\int\frac{\mathrm{2}{cos}^{\mathrm{2}} {x}+\mathrm{2}}{{cosx}\sqrt{\mathrm{2}{cos}^{\mathrm{2}} {x}+\mathrm{2}}} \\ $$$$\frac{{I}}{\mathrm{2}}=\int\frac{{cosx}}{\sqrt{\mathrm{2}\left({cos}^{\mathrm{2}} {x}+\mathrm{1}\right)}}{dx}+\int\frac{{secx}\:{dx}}{\sqrt{\mathrm{2}{cos}^{\mathrm{2}} {x}+\mathrm{2}}} \\ $$$$=\frac{\mathrm{1}}{\sqrt{\mathrm{2}}}\int\frac{{cosx}}{\sqrt{\mathrm{2}−{sin}^{\mathrm{2}} {x}}}{dx}+\frac{\mathrm{1}}{\sqrt{\mathrm{2}}}\int\frac{{sec}^{\mathrm{2}} {x}\:{dx}}{\sqrt{\mathrm{1}+{sec}^{\mathrm{2}} {x}}} \\ $$$$\frac{{I}}{\sqrt{\mathrm{2}}}=\int\frac{{d}\left({sinx}\right)}{\sqrt{\mathrm{2}−{sin}^{\mathrm{2}} {x}}}+\int\frac{{d}\left({tanx}\right)}{\sqrt{\mathrm{2}+{tan}^{\mathrm{2}} {x}}} \\ $$$${I}=\sqrt{\mathrm{2}}\:\left[{sin}^{−\mathrm{1}} \left(\frac{{sinx}}{\sqrt{\mathrm{2}}}\right)+{ln}\left({tanx}+\sqrt{\mathrm{2}+{tan}^{\mathrm{2}} {x}}\:\right)\right. \\ $$$${pls}\:{check} \\ $$$$ \\ $$

Commented by M±th+et£s last updated on 11/Apr/20

correct solution thank you sir

$${correct}\:{solution}\:{thank}\:{you}\:{sir} \\ $$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com