
Question Number 31069 by abdo imad last updated on 02/Mar/18
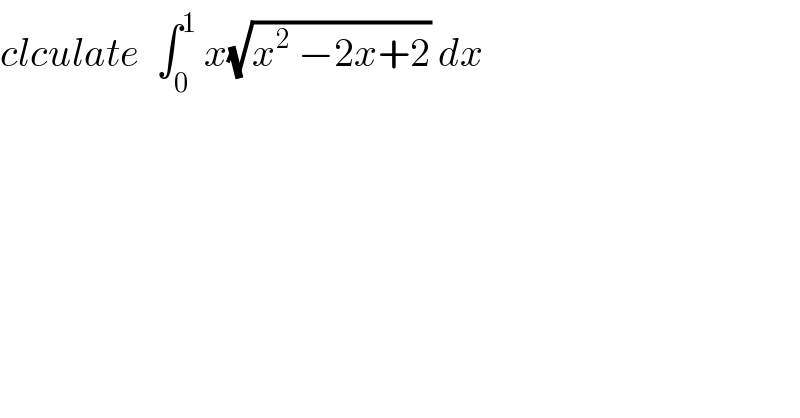
$${clculate}\:\:\int_{\mathrm{0}} ^{\mathrm{1}} \:{x}\sqrt{{x}^{\mathrm{2}} \:−\mathrm{2}{x}+\mathrm{2}}\:{dx} \\ $$
Commented by abdo imad last updated on 03/Mar/18
![let put I=∫_0 ^1 x(√(x^2 −2x +2dx)) I= ∫_0 ^1 x(√((x−1)^2 +1)) dx the ch. x−1=sht I= ∫_(argsh(−1)) ^o (1+sht)cht chtdt=−∫_0 ^(ln(−1+(√2))) ch^2 tdt −∫_0 ^(ln(−1+(√2))) sht ch^2 tdt but wehave ∫_0 ^(ln(−1+(√2))) ch^2 tdt= ∫_0 ^(ln(−1+(√2))) ((1+ch(2t))/2)dt =(1/2)ln(−1+(√2)) +(1/4)[sh(2t)]_0 ^(ln(−1+(√2) )) =(1/2)ln(−1+(√2)) +(1/4)sh(2ln(−1+(√2))) and ∫_0 ^(ln(−1+(√2))) sht ch^2 t dt=(1/3)[ ch^3 t]_0 ^(ln(−1+(√2))) =(1/3) (ch^3 (ln(−1+(√2))−1) by using shu =((e^u −e^(−u) )/2) and chu= ((e^u +e^(−u) )/2) tbe value of I isdetermined.](Q31206.png)
$${let}\:{put}\:{I}=\int_{\mathrm{0}} ^{\mathrm{1}} \:{x}\sqrt{{x}^{\mathrm{2}} \:−\mathrm{2}{x}\:+\mathrm{2}{dx}}\: \\ $$$${I}=\:\int_{\mathrm{0}} ^{\mathrm{1}} \:{x}\sqrt{\left({x}−\mathrm{1}\right)^{\mathrm{2}} \:+\mathrm{1}}\:{dx}\:{the}\:{ch}.\:{x}−\mathrm{1}={sht} \\ $$$${I}=\:\int_{{argsh}\left(−\mathrm{1}\right)} ^{{o}} \:\left(\mathrm{1}+{sht}\right){cht}\:{chtdt}=−\int_{\mathrm{0}} ^{{ln}\left(−\mathrm{1}+\sqrt{\mathrm{2}}\right)} {ch}^{\mathrm{2}} {tdt} \\ $$$$−\int_{\mathrm{0}} ^{{ln}\left(−\mathrm{1}+\sqrt{\mathrm{2}}\right)} {sht}\:{ch}^{\mathrm{2}} {tdt}\:\:{but}\:{wehave} \\ $$$$\int_{\mathrm{0}} ^{{ln}\left(−\mathrm{1}+\sqrt{\mathrm{2}}\right)} {ch}^{\mathrm{2}} {tdt}=\:\int_{\mathrm{0}} ^{{ln}\left(−\mathrm{1}+\sqrt{\mathrm{2}}\right)} \frac{\mathrm{1}+{ch}\left(\mathrm{2}{t}\right)}{\mathrm{2}}{dt} \\ $$$$=\frac{\mathrm{1}}{\mathrm{2}}{ln}\left(−\mathrm{1}+\sqrt{\mathrm{2}}\right)\:+\frac{\mathrm{1}}{\mathrm{4}}\left[{sh}\left(\mathrm{2}{t}\right)\right]_{\mathrm{0}} ^{{ln}\left(−\mathrm{1}+\sqrt{\mathrm{2}}\:\right)} \\ $$$$=\frac{\mathrm{1}}{\mathrm{2}}{ln}\left(−\mathrm{1}+\sqrt{\mathrm{2}}\right)\:+\frac{\mathrm{1}}{\mathrm{4}}{sh}\left(\mathrm{2}{ln}\left(−\mathrm{1}+\sqrt{\mathrm{2}}\right)\right)\:{and} \\ $$$$\int_{\mathrm{0}} ^{{ln}\left(−\mathrm{1}+\sqrt{\mathrm{2}}\right)} {sht}\:{ch}^{\mathrm{2}} {t}\:{dt}=\frac{\mathrm{1}}{\mathrm{3}}\left[\:{ch}^{\mathrm{3}} {t}\right]_{\mathrm{0}} ^{{ln}\left(−\mathrm{1}+\sqrt{\mathrm{2}}\right)} \\ $$$$=\frac{\mathrm{1}}{\mathrm{3}}\:\left({ch}^{\mathrm{3}} \left({ln}\left(−\mathrm{1}+\sqrt{\mathrm{2}}\right)−\mathrm{1}\right)\:{by}\:{using}\:{shu}\:=\frac{{e}^{{u}} \:−{e}^{−{u}} }{\mathrm{2}}\:{and}\right. \\ $$$${chu}=\:\frac{{e}^{{u}} \:+{e}^{−{u}} }{\mathrm{2}}\:{tbe}\:{value}\:{of}\:{I}\:{isdetermined}. \\ $$
Answered by Joel578 last updated on 02/Mar/18
![I = ∫_0 ^1 (x − 1 + 1)(√(x^2 − 2x + 2)) dx = ∫_0 ^1 (x − 1)(√(x^2 − 2x + 2)) dx + ∫_0 ^1 (√((x −1)^2 + 1)) dx I_1 = ∫ (x − 1)(√(x^2 − 2x + 2)) dx u = x^2 − 2x + 2 → du = 2(x −1) dx I_1 = (1/2)∫ (x − 1)(√u) . (du/(x − 1)) = (1/3)u(√u) = (1/3)(x^2 − 2x + 2)^(3/2) + C I_2 = ∫ (√((x − 1)^2 + 1)) dx x − 1 = tan θ → dx = sec^2 θ dθ I_2 = ∫ (√(tan^2 θ + 1)) . sec^2 θ dθ = ∫ sec^3 θ dθ = ((tan θ sec θ)/2) − (1/2)ln ∣sec θ + tan θ∣ + C = (((x − 1)(√(x^2 − 2x + 2)))/2) − (1/2)ln ∣(√(x^2 − 2x + 2)) + x − 1∣ + C I = [I_1 + I_2 ]_0 ^1 = [(1/3)(x^2 − 2x + 2)^(3/2) + (((x − 1)(√(x^2 − 2x + 2)))/2) − (1/2)ln ∣(√(x^2 − 2x + 2)) + x − 1∣]_0 ^1 = ((1/3) + 0 − 0) − (((2(√2))/3) − ((√2)/2) − (1/2)ln ((√2) − 1)) = ((1 − 2(√2))/3) + (1/2)((√2) + ln ((√2) − 1))](Q31110.png)
$${I}\:=\:\int_{\mathrm{0}} ^{\mathrm{1}} \:\left({x}\:−\:\mathrm{1}\:+\:\mathrm{1}\right)\sqrt{{x}^{\mathrm{2}} \:−\:\mathrm{2}{x}\:+\:\mathrm{2}}\:{dx} \\ $$$$\:\:\:=\:\int_{\mathrm{0}} ^{\mathrm{1}} \:\left({x}\:−\:\mathrm{1}\right)\sqrt{{x}^{\mathrm{2}} \:−\:\mathrm{2}{x}\:+\:\mathrm{2}}\:{dx}\:+\:\int_{\mathrm{0}} ^{\mathrm{1}} \:\sqrt{\left({x}\:−\mathrm{1}\right)^{\mathrm{2}} \:+\:\mathrm{1}}\:{dx} \\ $$$$ \\ $$$${I}_{\mathrm{1}} \:=\:\int\:\left({x}\:−\:\mathrm{1}\right)\sqrt{{x}^{\mathrm{2}} \:−\:\mathrm{2}{x}\:+\:\mathrm{2}}\:{dx} \\ $$$${u}\:=\:{x}^{\mathrm{2}} \:−\:\mathrm{2}{x}\:+\:\mathrm{2}\:\:\rightarrow\:\:{du}\:=\:\mathrm{2}\left({x}\:−\mathrm{1}\right)\:{dx} \\ $$$${I}_{\mathrm{1}} \:=\:\frac{\mathrm{1}}{\mathrm{2}}\int\:\left({x}\:−\:\mathrm{1}\right)\sqrt{{u}}\:.\:\frac{{du}}{{x}\:−\:\mathrm{1}} \\ $$$$\:\:\:\:\:=\:\frac{\mathrm{1}}{\mathrm{3}}{u}\sqrt{{u}}\:=\:\frac{\mathrm{1}}{\mathrm{3}}\left({x}^{\mathrm{2}} \:−\:\mathrm{2}{x}\:+\:\mathrm{2}\right)^{\frac{\mathrm{3}}{\mathrm{2}}} \:+\:{C} \\ $$$$ \\ $$$${I}_{\mathrm{2}} \:=\:\int\:\sqrt{\left({x}\:−\:\mathrm{1}\right)^{\mathrm{2}} \:+\:\mathrm{1}}\:{dx} \\ $$$${x}\:−\:\mathrm{1}\:=\:\mathrm{tan}\:\theta\:\:\rightarrow\:\:{dx}\:=\:\mathrm{sec}^{\mathrm{2}} \:\theta\:{d}\theta \\ $$$${I}_{\mathrm{2}} \:=\:\int\:\sqrt{\mathrm{tan}^{\mathrm{2}} \:\theta\:+\:\mathrm{1}}\:.\:\mathrm{sec}^{\mathrm{2}} \:\theta\:{d}\theta \\ $$$$\:\:\:\:\:=\:\int\:\mathrm{sec}^{\mathrm{3}} \:\theta\:{d}\theta \\ $$$$\:\:\:\:\:=\:\frac{\mathrm{tan}\:\theta\:\mathrm{sec}\:\theta}{\mathrm{2}}\:−\:\frac{\mathrm{1}}{\mathrm{2}}\mathrm{ln}\:\mid\mathrm{sec}\:\theta\:+\:\mathrm{tan}\:\theta\mid\:+\:{C} \\ $$$$\:\:\:\:\:=\:\frac{\left({x}\:−\:\mathrm{1}\right)\sqrt{{x}^{\mathrm{2}} \:−\:\mathrm{2}{x}\:+\:\mathrm{2}}}{\mathrm{2}}\:−\:\frac{\mathrm{1}}{\mathrm{2}}\mathrm{ln}\:\mid\sqrt{{x}^{\mathrm{2}} \:−\:\mathrm{2}{x}\:+\:\mathrm{2}}\:+\:{x}\:−\:\mathrm{1}\mid\:+\:{C} \\ $$$$ \\ $$$${I}\:=\:\left[{I}_{\mathrm{1}} \:+\:{I}_{\mathrm{2}} \right]_{\mathrm{0}} ^{\mathrm{1}} \\ $$$$\:\:\:=\:\left[\frac{\mathrm{1}}{\mathrm{3}}\left({x}^{\mathrm{2}} \:−\:\mathrm{2}{x}\:+\:\mathrm{2}\right)^{\frac{\mathrm{3}}{\mathrm{2}}} \:+\:\frac{\left({x}\:−\:\mathrm{1}\right)\sqrt{{x}^{\mathrm{2}} \:−\:\mathrm{2}{x}\:+\:\mathrm{2}}}{\mathrm{2}}\:−\:\frac{\mathrm{1}}{\mathrm{2}}\mathrm{ln}\:\mid\sqrt{{x}^{\mathrm{2}} \:−\:\mathrm{2}{x}\:+\:\mathrm{2}}\:+\:{x}\:−\:\mathrm{1}\mid\right]_{\mathrm{0}} ^{\mathrm{1}} \\ $$$$\:\:\:=\:\left(\frac{\mathrm{1}}{\mathrm{3}}\:+\:\mathrm{0}\:−\:\mathrm{0}\right)\:−\:\left(\frac{\mathrm{2}\sqrt{\mathrm{2}}}{\mathrm{3}}\:−\:\frac{\sqrt{\mathrm{2}}}{\mathrm{2}}\:−\:\frac{\mathrm{1}}{\mathrm{2}}\mathrm{ln}\:\left(\sqrt{\mathrm{2}}\:−\:\mathrm{1}\right)\right) \\ $$$$\:\:\:=\:\frac{\mathrm{1}\:−\:\mathrm{2}\sqrt{\mathrm{2}}}{\mathrm{3}}\:+\:\frac{\mathrm{1}}{\mathrm{2}}\left(\sqrt{\mathrm{2}}\:+\:\mathrm{ln}\:\left(\sqrt{\mathrm{2}}\:−\:\mathrm{1}\right)\right) \\ $$
