Question and Answers Forum

All Questions      Topic List

None Questions

Previous in All Question      Next in All Question      

Previous in None      Next in None      

Question Number 218265 by SdC355 last updated on 04/Apr/25

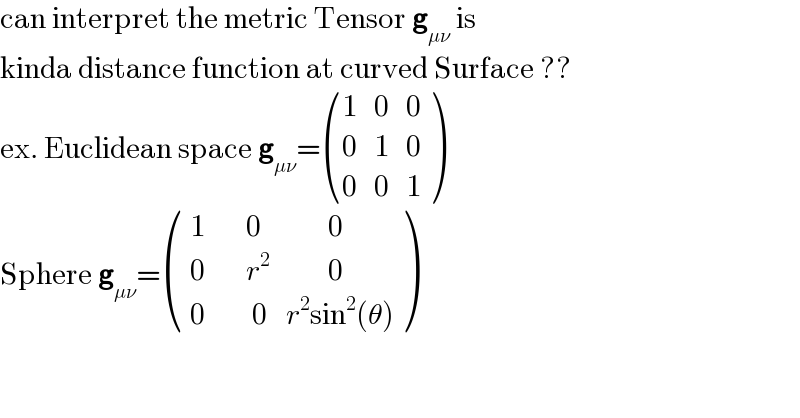
can interpret the metric Tensor g_(μν) is kinda distance function at curved Surface ?? ex. Euclidean space g_(μν) = ((1,0,0),(0,1,0),(0,0,1) ) Sphere g_(μν) = ((( 1),( 0),( 0)),(( 0),( r^2 ),( 0)),(( 0),( 0),(r^2 sin^2 (θ))) )

$$\mathrm{can}\:\mathrm{interpret}\:\mathrm{the}\:\mathrm{metric}\:\mathrm{Tensor}\:\boldsymbol{\mathrm{g}}_{\mu\nu} \:\mathrm{is}\: \\ $$$$\mathrm{kinda}\:\mathrm{distance}\:\mathrm{function}\:\mathrm{at}\:\mathrm{curved}\:\mathrm{Surface}\:?? \\ $$$$\mathrm{ex}.\:\mathrm{Euclidean}\:\mathrm{space}\:\boldsymbol{\mathrm{g}}_{\mu\nu} =\begin{pmatrix}{\mathrm{1}}&{\mathrm{0}}&{\mathrm{0}}\\{\mathrm{0}}&{\mathrm{1}}&{\mathrm{0}}\\{\mathrm{0}}&{\mathrm{0}}&{\mathrm{1}}\end{pmatrix} \\ $$$$\mathrm{Sphere}\:\boldsymbol{\mathrm{g}}_{\mu\nu} =\begin{pmatrix}{\:\mathrm{1}}&{\:\:\:\:\mathrm{0}}&{\:\:\:\:\:\:\:\mathrm{0}}\\{\:\mathrm{0}}&{\:\:\:\:{r}^{\mathrm{2}} }&{\:\:\:\:\:\:\:\mathrm{0}}\\{\:\mathrm{0}}&{\:\:\:\:\:\mathrm{0}}&{{r}^{\mathrm{2}} \mathrm{sin}^{\mathrm{2}} \left(\theta\right)}\end{pmatrix} \\ $$

Answered by MrGaster last updated on 05/Apr/25

g_(μν) ≜ds^2 =g_(μν) dx^μ □dx^ν δ_(μν) dx^μ dx^ν =dx^2 +dy^2 +dz^2 ∫_C (√(δ_(μν) (dx^μ /dt) (dx^ν /dt)))dt=(√((x_2 −x_(1 ) )^2 +(y_2 −y_1 )^2 +(z_2 −z_1 )^2 )) S^2 (r):g_(μν) = ((1,0,0),(0,r^2 ,0),(0,0,(r^2 sin^2 θ)) )⇒ds^2 =dr^2 +r^2 dθ^2 +r^2 sin^2 θ dφ^2 Constraint r=const⇒ds^2 =r^2 (dθ^2 +sin^2 θ dφ^2 ) ∫_θ_1 ^θ_2 (√(g_(θθ) ((dθ/dt))^2 ))dt=r(θ_2 −θ_1 ) ∫_0 ^(2π) (√(g_(φφ) ((dφ/dt))^2 ))dt=2πr sin θ Γ_(μν) ^λ =(1/2)g^(λσ) (∂_μ g_(σν) +∂_(ν ) g_(σμ) −∂_σ g_(μν) )⇒▽_μ g_(νρ) =0 Riem(g)=∂_ρ Γ_(μνσ) −∂_σ Γ_(μνρ) +Γ_(λρσ) Γ_(μν) ^λ −Γ_(λσ) Γ_(μν) ^λ L_(geodesic) =(√(g_(μν) x^. ^μ x^. ^ν ))⇒(D/dt)x^. ^μ +Γ_(νρ) ^μ x^. ^ν x^. ^ρ =0

$$\mathrm{g}_{\mu\nu} \triangleq{ds}^{\mathrm{2}} ={g}_{\mu\nu} {dx}^{\mu} \square{dx}^{\nu} \\ $$$$\delta_{\mu\nu} {dx}^{\mu} {dx}^{\nu} ={dx}^{\mathrm{2}} +{dy}^{\mathrm{2}} +{dz}^{\mathrm{2}} \\ $$$$\int_{{C}} \sqrt{\delta_{\mu\nu} \frac{{dx}^{\mu} }{{dt}}\:\frac{{dx}^{\nu} }{{dt}}}{dt}=\sqrt{\left({x}_{\mathrm{2}} −{x}_{\mathrm{1}\:} \right)^{\mathrm{2}} +\left({y}_{\mathrm{2}} −{y}_{\mathrm{1}} \right)^{\mathrm{2}} +\left({z}_{\mathrm{2}} −{z}_{\mathrm{1}} \right)^{\mathrm{2}} } \\ $$$$\mathbb{S}^{\mathrm{2}} \left({r}\right):\mathrm{g}_{\mu\nu} =\begin{pmatrix}{\mathrm{1}}&{\mathrm{0}}&{\mathrm{0}}\\{\mathrm{0}}&{{r}^{\mathrm{2}} }&{\mathrm{0}}\\{\mathrm{0}}&{\mathrm{0}}&{{r}^{\mathrm{2}} \mathrm{sin}^{\mathrm{2}} \theta}\end{pmatrix}\Rightarrow{ds}^{\mathrm{2}} ={dr}^{\mathrm{2}} +{r}^{\mathrm{2}} {d}\theta^{\mathrm{2}} +{r}^{\mathrm{2}} \mathrm{sin}^{\mathrm{2}} \theta\:{d}\phi^{\mathrm{2}} \\ $$$$\mathrm{Constraint}\:{r}=\mathrm{const}\Rightarrow{ds}^{\mathrm{2}} ={r}^{\mathrm{2}} \left({d}\theta^{\mathrm{2}} +\mathrm{sin}^{\mathrm{2}} \theta\:{d}\phi^{\mathrm{2}} \right) \\ $$$$\int_{\theta_{\mathrm{1}} } ^{\theta_{\mathrm{2}} } \sqrt{{g}_{\theta\theta} \left(\frac{{d}\theta}{{dt}}\right)^{\mathrm{2}} }{dt}={r}\left(\theta_{\mathrm{2}} −\theta_{\mathrm{1}} \right) \\ $$$$\int_{\mathrm{0}} ^{\mathrm{2}\pi} \sqrt{{g}_{\phi\phi} \left(\frac{{d}\phi}{{dt}}\right)^{\mathrm{2}} }{dt}=\mathrm{2}\pi{r}\:\mathrm{sin}\:\theta \\ $$$$\Gamma_{\mu\nu} ^{\lambda} =\frac{\mathrm{1}}{\mathrm{2}}{g}^{\lambda\sigma} \left(\partial_{\mu} {g}_{\sigma\nu} +\partial_{\nu\:} {g}_{\sigma\mu} −\partial_{\sigma} {g}_{\mu\nu} \right)\Rightarrow\bigtriangledown_{\mu} {g}_{\nu\rho} =\mathrm{0} \\ $$$$\mathrm{Riem}\left({g}\right)=\partial_{\rho} \Gamma_{\mu\nu\sigma} −\partial_{\sigma} \Gamma_{\mu\nu\rho} +\Gamma_{\lambda\rho\sigma} \Gamma_{\mu\nu} ^{\lambda} −\Gamma_{\lambda\sigma} \Gamma_{\mu\nu} ^{\lambda} \\ $$$$\mathcal{L}_{\mathrm{geodesic}} =\sqrt{{g}_{\mu\nu} \overset{.} {{x}}\:^{\mu} \overset{.} {{x}}\:^{\nu} }\Rightarrow\frac{{D}}{{dt}}\overset{.} {{x}}\:^{\mu} +\Gamma_{\nu\rho} ^{\mu} \overset{.} {{x}}\:^{\nu} \overset{.} {{x}}\:^{\rho} =\mathrm{0} \\ $$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com