
Question Number 34716 by abdo mathsup 649 cc last updated on 10/May/18
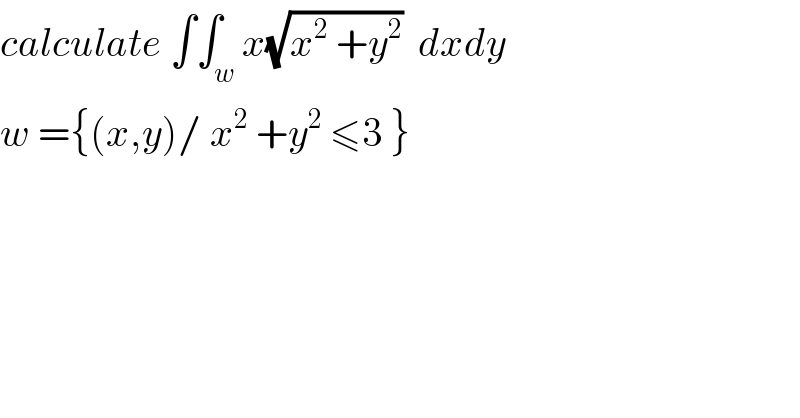
$${calculate}\:\int\int_{{w}} {x}\sqrt{{x}^{\mathrm{2}} \:+{y}^{\mathrm{2}} }\:\:{dxdy} \\ $$$${w}\:=\left\{\left({x},{y}\right)/\:{x}^{\mathrm{2}} \:+{y}^{\mathrm{2}} \:\leqslant\mathrm{3}\:\right\}\: \\ $$
Commented by prof Abdo imad last updated on 11/May/18
![let consider thediffeomorphism (r,θ)→(x,y)=(rcosθ,rsinθ) /0≤r≤(√3) and −π≤θ≤π I =∫∫_(0≤r≤(√3) and −π≤θ≤π) rcosθ r.rdrdθ = ∫_0 ^(√3) r^3 dr .∫_(−π) ^π cosθ dθ =(1/4)((√3))^3 [sinθ]_(−π) ^π =0 I =0](Q34815.png)
$${let}\:{consider}\:{thediffeomorphism} \\ $$$$\left({r},\theta\right)\rightarrow\left({x},{y}\right)=\left({rcos}\theta,{rsin}\theta\right)\:/\mathrm{0}\leqslant{r}\leqslant\sqrt{\mathrm{3}} \\ $$$${and}\:−\pi\leqslant\theta\leqslant\pi \\ $$$${I}\:\:=\int\int_{\mathrm{0}\leqslant{r}\leqslant\sqrt{\mathrm{3}}\:\:{and}\:\:−\pi\leqslant\theta\leqslant\pi} {rcos}\theta\:{r}.{rdrd}\theta \\ $$$$=\:\int_{\mathrm{0}} ^{\sqrt{\mathrm{3}}} \:{r}^{\mathrm{3}} {dr}\:.\int_{−\pi} ^{\pi} {cos}\theta\:{d}\theta\:=\frac{\mathrm{1}}{\mathrm{4}}\left(\sqrt{\mathrm{3}}\right)^{\mathrm{3}} \left[{sin}\theta\right]_{−\pi} ^{\pi} \:\:=\mathrm{0} \\ $$$${I}\:=\mathrm{0} \\ $$
