
Question Number 86643 by mathmax by abdo last updated on 29/Mar/20
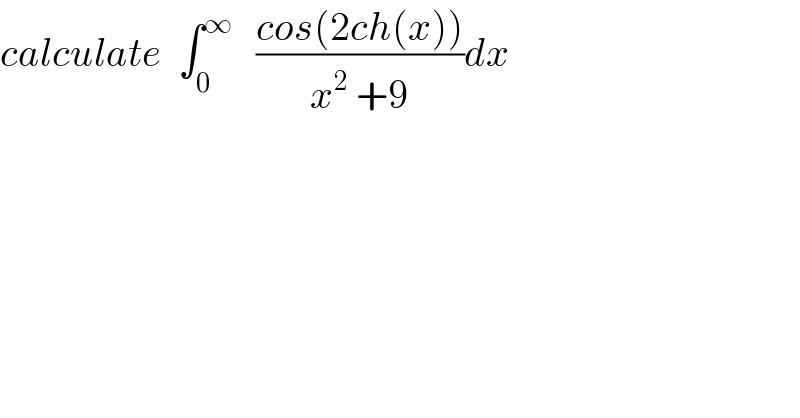
$${calculate}\:\:\int_{\mathrm{0}} ^{\infty} \:\:\:\frac{{cos}\left(\mathrm{2}{ch}\left({x}\right)\right)}{{x}^{\mathrm{2}} \:+\mathrm{9}}{dx} \\ $$
Commented by mathmax by abdo last updated on 01/Apr/20
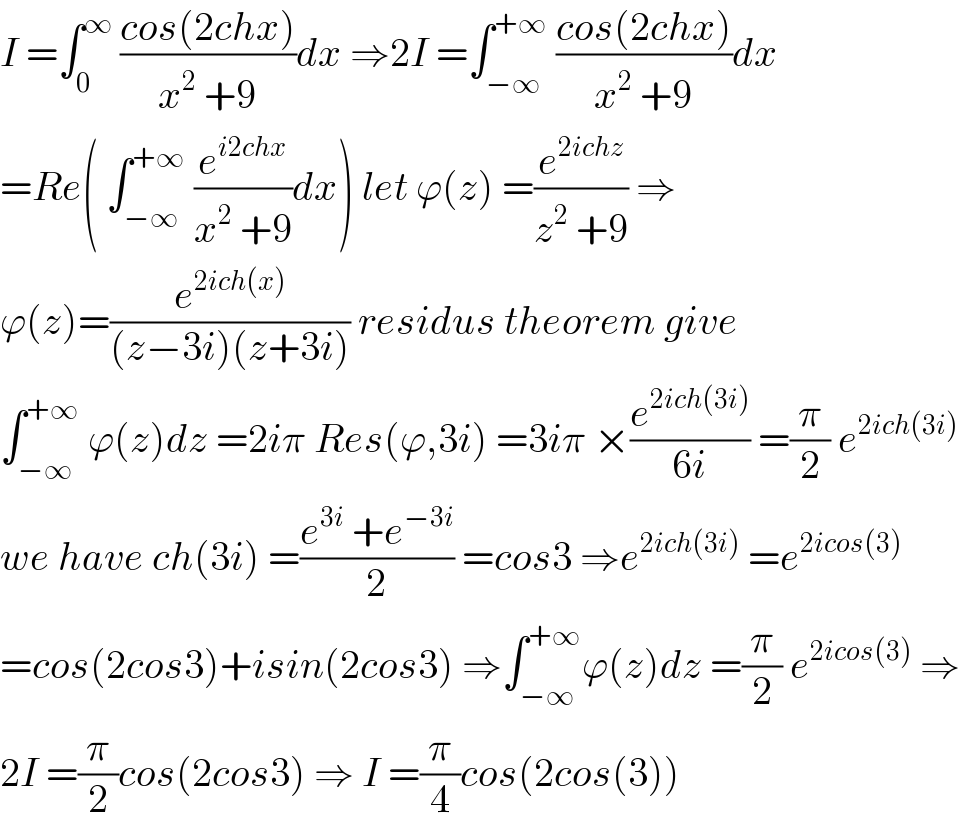
$${I}\:=\int_{\mathrm{0}} ^{\infty} \:\frac{{cos}\left(\mathrm{2}{chx}\right)}{{x}^{\mathrm{2}} \:+\mathrm{9}}{dx}\:\Rightarrow\mathrm{2}{I}\:=\int_{−\infty} ^{+\infty} \:\frac{{cos}\left(\mathrm{2}{chx}\right)}{{x}^{\mathrm{2}} \:+\mathrm{9}}{dx} \\ $$$$={Re}\left(\:\int_{−\infty} ^{+\infty} \:\frac{{e}^{{i}\mathrm{2}{chx}} }{{x}^{\mathrm{2}} \:+\mathrm{9}}{dx}\right)\:{let}\:\varphi\left({z}\right)\:=\frac{{e}^{\mathrm{2}{ichz}} }{{z}^{\mathrm{2}} \:+\mathrm{9}}\:\Rightarrow \\ $$$$\varphi\left({z}\right)=\frac{{e}^{\mathrm{2}{ich}\left({x}\right)} }{\left({z}−\mathrm{3}{i}\right)\left({z}+\mathrm{3}{i}\right)}\:{residus}\:{theorem}\:{give} \\ $$$$\int_{−\infty} ^{+\infty} \:\varphi\left({z}\right){dz}\:=\mathrm{2}{i}\pi\:{Res}\left(\varphi,\mathrm{3}{i}\right)\:=\mathrm{3}{i}\pi\:×\frac{{e}^{\mathrm{2}{ich}\left(\mathrm{3}{i}\right)} }{\mathrm{6}{i}}\:=\frac{\pi}{\mathrm{2}}\:{e}^{\mathrm{2}{ich}\left(\mathrm{3}{i}\right)} \\ $$$${we}\:{have}\:{ch}\left(\mathrm{3}{i}\right)\:=\frac{{e}^{\mathrm{3}{i}} \:+{e}^{−\mathrm{3}{i}} }{\mathrm{2}}\:={cos}\mathrm{3}\:\Rightarrow{e}^{\mathrm{2}{ich}\left(\mathrm{3}{i}\right)} \:={e}^{\mathrm{2}{icos}\left(\mathrm{3}\right)} \\ $$$$={cos}\left(\mathrm{2}{cos}\mathrm{3}\right)+{isin}\left(\mathrm{2}{cos}\mathrm{3}\right)\:\Rightarrow\int_{−\infty} ^{+\infty} \varphi\left({z}\right){dz}\:=\frac{\pi}{\mathrm{2}}\:{e}^{\mathrm{2}{icos}\left(\mathrm{3}\right)} \:\Rightarrow \\ $$$$\mathrm{2}{I}\:=\frac{\pi}{\mathrm{2}}{cos}\left(\mathrm{2}{cos}\mathrm{3}\right)\:\Rightarrow\:{I}\:=\frac{\pi}{\mathrm{4}}{cos}\left(\mathrm{2}{cos}\left(\mathrm{3}\right)\right) \\ $$
Commented by Ar Brandon last updated on 02/Apr/20
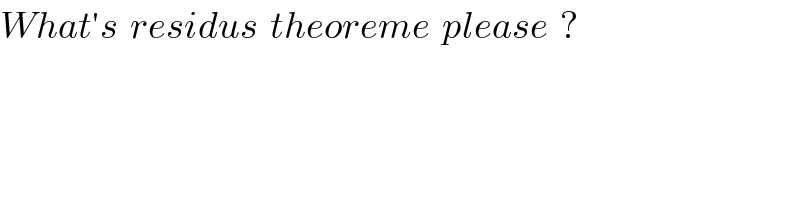
$${What}'{s}\:\:{residus}\:\:{theoreme}\:\:{please}\:\:? \\ $$
