
Question Number 163798 by kdaramaths last updated on 10/Jan/22

$$ \\ $$$$\:\:\:\:{by}\:{a}\:{simple}\:{method}\:{calculate}\:\:\: \\ $$$$\:\:\: \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\sum_{{k}=\mathrm{0}} ^{{n}} {COS}\left({kx}\right) \\ $$$$ \\ $$$$ \\ $$
Answered by Ar Brandon last updated on 10/Jan/22

$$\mathrm{Q162872} \\ $$
Answered by Kamel last updated on 10/Jan/22
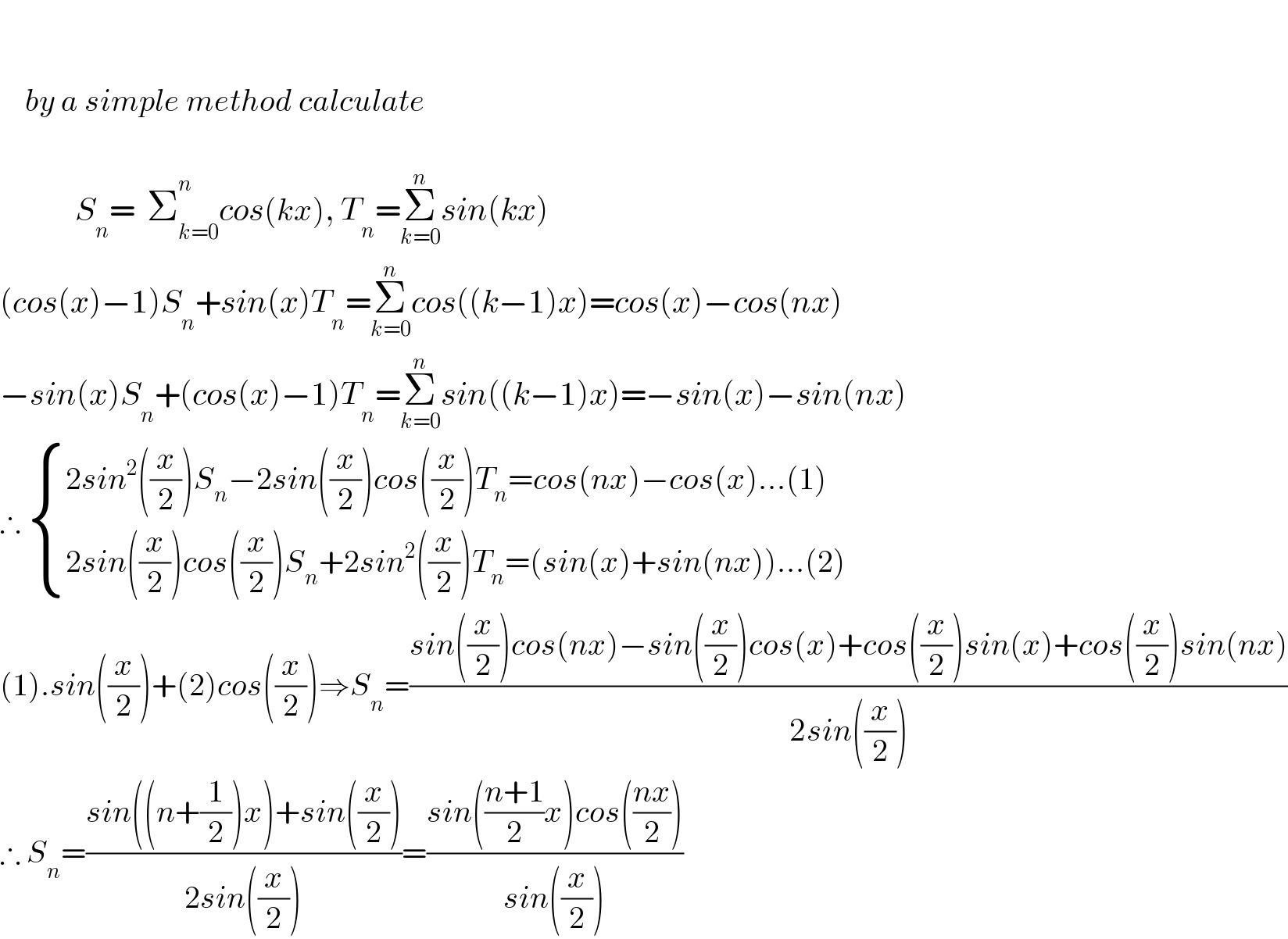
$$ \\ $$$$ \\ $$$$\:\:\:\:{by}\:{a}\:{simple}\:{method}\:{calculate}\:\:\: \\ $$$$\:\:\: \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:{S}_{{n}} =\:\:\sum_{{k}=\mathrm{0}} ^{{n}} {cos}\left({kx}\right),\:{T}_{{n}} =\underset{{k}=\mathrm{0}} {\overset{{n}} {\sum}}{sin}\left({kx}\right) \\ $$$$\left({cos}\left({x}\right)−\mathrm{1}\right){S}_{{n}} +{sin}\left({x}\right){T}_{{n}} =\underset{{k}=\mathrm{0}} {\overset{{n}} {\sum}}{cos}\left(\left({k}−\mathrm{1}\right){x}\right)={cos}\left({x}\right)−{cos}\left({nx}\right) \\ $$$$−{sin}\left({x}\right){S}_{{n}} +\left({cos}\left({x}\right)−\mathrm{1}\right){T}_{{n}} =\underset{{k}=\mathrm{0}} {\overset{{n}} {\sum}}{sin}\left(\left({k}−\mathrm{1}\right){x}\right)=−{sin}\left({x}\right)−{sin}\left({nx}\right) \\ $$$$\therefore\:\begin{cases}{\mathrm{2}{sin}^{\mathrm{2}} \left(\frac{{x}}{\mathrm{2}}\right){S}_{{n}} −\mathrm{2}{sin}\left(\frac{{x}}{\mathrm{2}}\right){cos}\left(\frac{{x}}{\mathrm{2}}\right){T}_{{n}} ={cos}\left({nx}\right)−{cos}\left({x}\right)...\left(\mathrm{1}\right)}\\{\mathrm{2}{sin}\left(\frac{{x}}{\mathrm{2}}\right){cos}\left(\frac{{x}}{\mathrm{2}}\right){S}_{{n}} +\mathrm{2}{sin}^{\mathrm{2}} \left(\frac{{x}}{\mathrm{2}}\right){T}_{{n}} =\left({sin}\left({x}\right)+{sin}\left({nx}\right)\right)...\left(\mathrm{2}\right)}\end{cases} \\ $$$$\left(\mathrm{1}\right).{sin}\left(\frac{{x}}{\mathrm{2}}\right)+\left(\mathrm{2}\right){cos}\left(\frac{{x}}{\mathrm{2}}\right)\Rightarrow{S}_{{n}} =\frac{{sin}\left(\frac{{x}}{\mathrm{2}}\right){cos}\left({nx}\right)−{sin}\left(\frac{{x}}{\mathrm{2}}\right){cos}\left({x}\right)+{cos}\left(\frac{{x}}{\mathrm{2}}\right){sin}\left({x}\right)+{cos}\left(\frac{{x}}{\mathrm{2}}\right){sin}\left({nx}\right)}{\mathrm{2}{sin}\left(\frac{{x}}{\mathrm{2}}\right)} \\ $$$$\therefore\:{S}_{{n}} =\frac{{sin}\left(\left({n}+\frac{\mathrm{1}}{\mathrm{2}}\right){x}\right)+{sin}\left(\frac{{x}}{\mathrm{2}}\right)}{\mathrm{2}{sin}\left(\frac{{x}}{\mathrm{2}}\right)}=\frac{{sin}\left(\frac{{n}+\mathrm{1}}{\mathrm{2}}{x}\right){cos}\left(\frac{{nx}}{\mathrm{2}}\right)}{{sin}\left(\frac{{x}}{\mathrm{2}}\right)} \\ $$
