Question and Answers Forum

All Questions      Topic List

Arithmetic Questions

Previous in All Question      Next in All Question      

Previous in Arithmetic      Next in Arithmetic      

Question Number 46809 by MJS last updated on 31/Oct/18

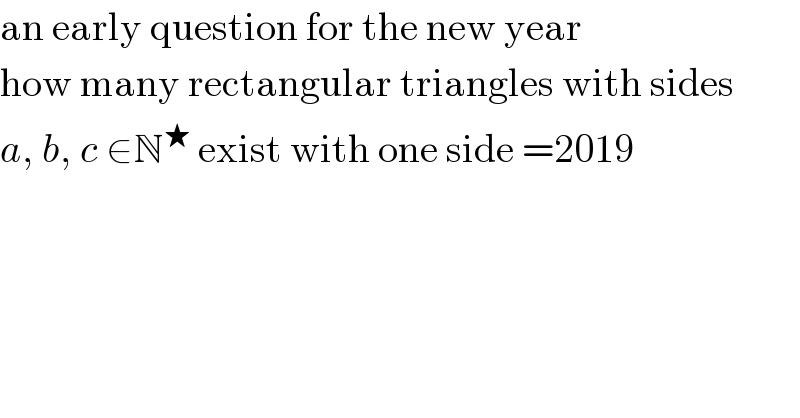
an early question for the new year how many rectangular triangles with sides a, b, c ∈N^★ exist with one side =2019

$$\mathrm{an}\:\mathrm{early}\:\mathrm{question}\:\mathrm{for}\:\mathrm{the}\:\mathrm{new}\:\mathrm{year} \\ $$$$\mathrm{how}\:\mathrm{many}\:\mathrm{rectangular}\:\mathrm{triangles}\:\mathrm{with}\:\mathrm{sides} \\ $$$${a},\:{b},\:{c}\:\in\mathbb{N}^{\bigstar} \:\mathrm{exist}\:\mathrm{with}\:\mathrm{one}\:\mathrm{side}\:=\mathrm{2019} \\ $$

Answered by MrW3 last updated on 02/Nov/18

a^2 +b^2 =c^2 case 1: with c=2019 a^2 +b^2 =2019^2 =3^2 ×673^2 let a=3p, b=3q ⇒p^2 +q^2 =673^2 it has one and only one solution: p=385, q=552 ⇒a=3×385=1155 ⇒b=3×552=1656 case 2: with a=2019 2019^2 +b^2 =c^2 ⇒c^2 −b^2 =(c−b)(c+b)=2019^2 =3^2 ×673^2 =m×n=1×4076361=3×1358787=9×452929=673×6057 c−b=m c+b=n ⇒b=((n−m)/2) ⇒c=((n+m)/2) ⇒b=((4076361−1)/2)=2038180 ⇒c=((4076361+1)/2)=2038181 or ⇒b=((1358787−3)/2)=679392 ⇒c=((1358787+3)/2)=679395 or ⇒b=((452929−9)/2)=226460 ⇒c=((452929+9)/2)=226469 or ⇒b=((6057−673)/2)=2692 ⇒c=((6057+673)/2)=3365 so totally there exist 5 such triangles: a/b/c=1155/1656/2019 a/b/c=2019/2692/3365 a/b/c=2019/226460/226469 a/b/c=2019/679392/679395 a/b/c=2019/2038180/2038181

$${a}^{\mathrm{2}} +{b}^{\mathrm{2}} ={c}^{\mathrm{2}} \\ $$$$\boldsymbol{{case}}\:\mathrm{1}:\:{with}\:{c}=\mathrm{2019} \\ $$$${a}^{\mathrm{2}} +{b}^{\mathrm{2}} =\mathrm{2019}^{\mathrm{2}} =\mathrm{3}^{\mathrm{2}} ×\mathrm{673}^{\mathrm{2}} \\ $$$${let}\:{a}=\mathrm{3}{p},\:{b}=\mathrm{3}{q} \\ $$$$\Rightarrow{p}^{\mathrm{2}} +{q}^{\mathrm{2}} =\mathrm{673}^{\mathrm{2}} \\ $$$${it}\:{has}\:{one}\:{and}\:{only}\:{one}\:{solution}: \\ $$$${p}=\mathrm{385},\:{q}=\mathrm{552} \\ $$$$\Rightarrow{a}=\mathrm{3}×\mathrm{385}=\mathrm{1155} \\ $$$$\Rightarrow{b}=\mathrm{3}×\mathrm{552}=\mathrm{1656} \\ $$$$ \\ $$$$\boldsymbol{{case}}\:\mathrm{2}:\:{with}\:{a}=\mathrm{2019} \\ $$$$\mathrm{2019}^{\mathrm{2}} +{b}^{\mathrm{2}} ={c}^{\mathrm{2}} \\ $$$$\Rightarrow{c}^{\mathrm{2}} −{b}^{\mathrm{2}} =\left({c}−{b}\right)\left({c}+{b}\right)=\mathrm{2019}^{\mathrm{2}} =\mathrm{3}^{\mathrm{2}} ×\mathrm{673}^{\mathrm{2}} ={m}×{n}=\mathrm{1}×\mathrm{4076361}=\mathrm{3}×\mathrm{1358787}=\mathrm{9}×\mathrm{452929}=\mathrm{673}×\mathrm{6057} \\ $$$${c}−{b}={m} \\ $$$${c}+{b}={n} \\ $$$$\Rightarrow{b}=\frac{{n}−{m}}{\mathrm{2}} \\ $$$$\Rightarrow{c}=\frac{{n}+{m}}{\mathrm{2}} \\ $$$$\Rightarrow{b}=\frac{\mathrm{4076361}−\mathrm{1}}{\mathrm{2}}=\mathrm{2038180} \\ $$$$\Rightarrow{c}=\frac{\mathrm{4076361}+\mathrm{1}}{\mathrm{2}}=\mathrm{2038181} \\ $$$${or} \\ $$$$\Rightarrow{b}=\frac{\mathrm{1358787}−\mathrm{3}}{\mathrm{2}}=\mathrm{679392} \\ $$$$\Rightarrow{c}=\frac{\mathrm{1358787}+\mathrm{3}}{\mathrm{2}}=\mathrm{679395} \\ $$$${or} \\ $$$$\Rightarrow{b}=\frac{\mathrm{452929}−\mathrm{9}}{\mathrm{2}}=\mathrm{226460} \\ $$$$\Rightarrow{c}=\frac{\mathrm{452929}+\mathrm{9}}{\mathrm{2}}=\mathrm{226469} \\ $$$${or} \\ $$$$\Rightarrow{b}=\frac{\mathrm{6057}−\mathrm{673}}{\mathrm{2}}=\mathrm{2692} \\ $$$$\Rightarrow{c}=\frac{\mathrm{6057}+\mathrm{673}}{\mathrm{2}}=\mathrm{3365} \\ $$$$ \\ $$$${so}\:\boldsymbol{{totally}}\:{there}\:{exist}\:\mathrm{5}\:{such}\:{triangles}: \\ $$$${a}/{b}/{c}=\mathrm{1155}/\mathrm{1656}/\mathrm{2019} \\ $$$${a}/{b}/{c}=\mathrm{2019}/\mathrm{2692}/\mathrm{3365} \\ $$$${a}/{b}/{c}=\mathrm{2019}/\mathrm{226460}/\mathrm{226469} \\ $$$${a}/{b}/{c}=\mathrm{2019}/\mathrm{679392}/\mathrm{679395} \\ $$$${a}/{b}/{c}=\mathrm{2019}/\mathrm{2038180}/\mathrm{2038181} \\ $$

Commented by MJS last updated on 02/Nov/18

you′re right, thank you!

$$\mathrm{you}'\mathrm{re}\:\mathrm{right},\:\mathrm{thank}\:\mathrm{you}! \\ $$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com