
Question Number 115621 by sachin1221 last updated on 27/Sep/20
![... advanced calculus... evaluate :: show that lim_(n→∞) (1/n)[cos^(2p) (π/(2n))+cos^(2p) ((2π)/(2n))+cos^(2p) ((3π)/(2n))......cos^(2p) (π/2)] =Π_(r=1) ^p ((p+r)/(4r))](Q115621.png)
$$ \\ $$$$\:\:\:\:\:\:\:...\:{advanced}\:\:\:{calculus}...\: \\ $$$$\:\:\:\:\:\:\:{evaluate}\::: \\ $$$${show}\:{that}\:{lim}_{{n}\rightarrow\infty} \frac{\mathrm{1}}{{n}}\left[{cos}^{\mathrm{2}{p}} \frac{\pi}{\mathrm{2}{n}}+{cos}^{\mathrm{2}{p}} \frac{\mathrm{2}\pi}{\mathrm{2}{n}}+{cos}^{\mathrm{2}{p}} \frac{\mathrm{3}\pi}{\mathrm{2}{n}}......{cos}^{\mathrm{2}{p}} \frac{\pi}{\mathrm{2}}\right]\:=\underset{{r}=\mathrm{1}} {\overset{{p}} {\prod}}\frac{{p}+{r}}{\mathrm{4}{r}} \\ $$
Answered by TANMAY PANACEA last updated on 27/Sep/20

$$\underset{{n}\rightarrow\infty} {\mathrm{lim}}\frac{\mathrm{1}}{{n}}\underset{{r}=\mathrm{1}} {\overset{{n}} {\sum}}\left({cos}\frac{{r}\pi}{\mathrm{2}{n}}\right)^{\mathrm{2}{p}} \\ $$$$\int_{\mathrm{0}} ^{\mathrm{1}} {cos}\left(\frac{{x}\pi}{\mathrm{2}}\right)^{\mathrm{2}{p}} {dx} \\ $$$${t}=\frac{{x}\pi}{\mathrm{2}}\rightarrow\frac{{dt}}{{dx}}=\frac{\pi}{\mathrm{2}} \\ $$$$\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{2}}} {cos}^{\mathrm{2}{p}} {t}×\frac{\mathrm{2}}{\pi}{dt} \\ $$$$\pi{I}=\mathrm{2}\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{2}}} {cos}^{\mathrm{2}{p}} {t}×{sin}^{\mathrm{0}} {t}\:{dt} \\ $$$${now}\:{i}\:{shall}\:{use}\:{gamma}\:{function} \\ $$$${formula} \\ $$$$\mathrm{2}\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{2}}} \left({sin}\theta\right)^{\mathrm{2}{m}−\mathrm{1}} \left({cos}\theta\right)^{\mathrm{2}{n}−\mathrm{1}} {d}\theta=\frac{\lceil\left({m}\right)\lceil\left({n}\right)}{\lceil\left({m}+{n}\right)} \\ $$$$\lceil\rightarrow{gamma}\:{operator} \\ $$$$\pi{I}=\mathrm{2}\int_{\mathrm{0}\:} ^{\frac{\pi}{\mathrm{2}}} \left({sint}\right)^{\mathrm{2}×\frac{\mathrm{1}}{\mathrm{2}}−\mathrm{1}} \left({cost}\right)^{\mathrm{2}×\frac{\mathrm{2}{p}+\mathrm{1}}{\mathrm{2}}−\mathrm{1}} {dt} \\ $$$$\boldsymbol{{I}}=\frac{\mathrm{1}}{\pi}×\frac{\lceil\left(\frac{\mathrm{1}}{\mathrm{2}}\right)×\lceil\left(\frac{\mathrm{2}{p}+\mathrm{1}}{\mathrm{2}}\right)}{\lceil\left(\frac{\mathrm{1}}{\mathrm{2}}+\frac{\mathrm{2}{p}+\mathrm{1}}{\mathrm{2}}\right)} \\ $$
Commented by Rasheed.Sindhi last updated on 27/Sep/20
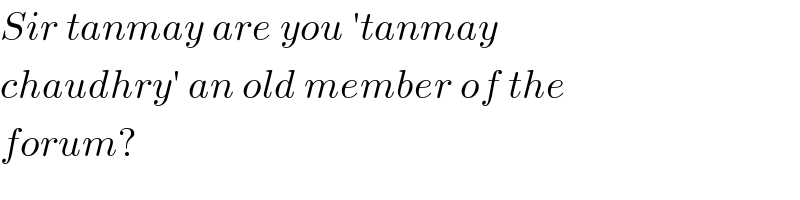
$${Sir}\:{tanmay}\:{are}\:{you}\:'{tanmay} \\ $$$${chaudhry}'\:{an}\:{old}\:{member}\:{of}\:{the} \\ $$$${forum}? \\ $$
Commented by TANMAY PANACEA last updated on 27/Sep/20
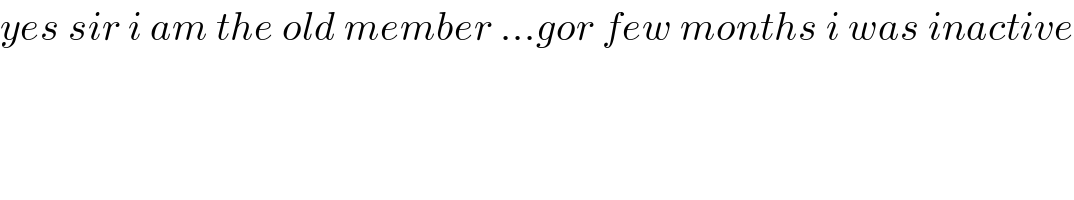
$${yes}\:{sir}\:{i}\:{am}\:{the}\:{old}\:{member}\:...{gor}\:{few}\:{months}\:{i}\:{was}\:{inactive} \\ $$
Commented by TANMAY PANACEA last updated on 27/Sep/20
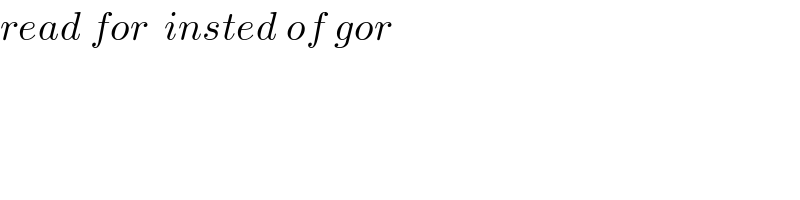
$${read}\:{for}\:\:{insted}\:{of}\:{gor} \\ $$
Commented by bemath last updated on 27/Sep/20
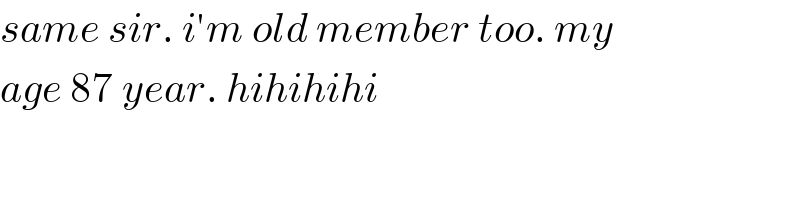
$${same}\:{sir}.\:{i}'{m}\:{old}\:{member}\:{too}.\:{my} \\ $$$${age}\:\mathrm{87}\:{year}.\:{hihihihi} \\ $$
Commented by Ar Brandon last updated on 27/Sep/20
��I now remember you Mr Tanmay. You once helped me out with Q86830. Nice to meet you again Sir��
Commented by Ar Brandon last updated on 27/Sep/20
��BeMath stop kidding bro. I once had a look at your profile picture and you aren't that old��. You're a young guy. <20 years old I guess. You rather look good.�� Are you gonna tell me now that it was your grandson ? ��
Commented by bemath last updated on 27/Sep/20

$${oohh}\:{no}\:{bro} \\ $$
Commented by Dwaipayan Shikari last updated on 27/Sep/20
An e^2.8 bro I am����
Commented by bemath last updated on 27/Sep/20
$${good}.\:{your}\:{is}\:{the}\:{santuy} \\ $$
Commented by Rasheed.Sindhi last updated on 27/Sep/20
$$\mathcal{T}{hanks}\:{tanmay}\:{sir}! \\ $$
Answered by Ar Brandon last updated on 27/Sep/20
![S=lim_(n→∞) (1/n)[cos^(2p) ((π/(2n)))+cos^(2p) (((2π)/(2n)))+cos^(2p) (((3π)/(2n)))+∙∙∙+cos^(2p) ((π/2))] =lim_(n→∞) (1/n)Σ_(k=1) ^n cos^(2p) (((kπ)/(2n)))=∫_0 ^1 cos^(2p) (((πx)/2))dx (Reimann′s Sum) S=∫_0 ^1 cos^(2p) (((πx)/2))dx=(2/π)∫_0 ^(π/2) cos^(2p) (u)du {setting ((πx)/2)=u} =(2/π)[(1/2)∙(3/4)∙(5/6)∙∙∙((2p−5)/(2p−4))∙((2p−3)/(2p−2))∙((2p−1)/(2p))∙(π/2)] (Walli′s method) =(2/π)∙(π/2)∙Π_(r=0) ^(p−1) ((2p−(2r+1))/(2p−2r))=Π_(r=0) ^(p−1) ((2p−(2r+1))/(2p−2r))](Q115644.png)
$$\mathrm{S}=\underset{\mathrm{n}\rightarrow\infty} {\mathrm{lim}}\frac{\mathrm{1}}{\mathrm{n}}\left[\mathrm{cos}^{\mathrm{2p}} \left(\frac{\pi}{\mathrm{2n}}\right)+\mathrm{cos}^{\mathrm{2p}} \left(\frac{\mathrm{2}\pi}{\mathrm{2n}}\right)+\mathrm{cos}^{\mathrm{2p}} \left(\frac{\mathrm{3}\pi}{\mathrm{2n}}\right)+\centerdot\centerdot\centerdot+\mathrm{cos}^{\mathrm{2p}} \left(\frac{\pi}{\mathrm{2}}\right)\right] \\ $$$$\:\:\:=\underset{\mathrm{n}\rightarrow\infty} {\mathrm{lim}}\frac{\mathrm{1}}{\mathrm{n}}\underset{\mathrm{k}=\mathrm{1}} {\overset{\mathrm{n}} {\sum}}\mathrm{cos}^{\mathrm{2p}} \left(\frac{\mathrm{k}\pi}{\mathrm{2n}}\right)=\int_{\mathrm{0}} ^{\mathrm{1}} \mathrm{cos}^{\mathrm{2p}} \left(\frac{\pi{x}}{\mathrm{2}}\right)\mathrm{d}{x}\:\:\left({Reimann}'{s}\:{Sum}\right) \\ $$$$\mathrm{S}=\int_{\mathrm{0}} ^{\mathrm{1}} \mathrm{cos}^{\mathrm{2p}} \left(\frac{\pi{x}}{\mathrm{2}}\right)\mathrm{d}{x}=\frac{\mathrm{2}}{\pi}\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{2}}} \mathrm{cos}^{\mathrm{2p}} \left(\mathrm{u}\right)\mathrm{du}\:\:\left\{{setting}\:\frac{\pi{x}}{\mathrm{2}}=\mathrm{u}\right\} \\ $$$$\:\:\:=\frac{\mathrm{2}}{\pi}\left[\frac{\mathrm{1}}{\mathrm{2}}\centerdot\frac{\mathrm{3}}{\mathrm{4}}\centerdot\frac{\mathrm{5}}{\mathrm{6}}\centerdot\centerdot\centerdot\frac{\mathrm{2p}−\mathrm{5}}{\mathrm{2p}−\mathrm{4}}\centerdot\frac{\mathrm{2p}−\mathrm{3}}{\mathrm{2p}−\mathrm{2}}\centerdot\frac{\mathrm{2p}−\mathrm{1}}{\mathrm{2p}}\centerdot\frac{\pi}{\mathrm{2}}\right]\:\:\left({Walli}'{s}\:{method}\right) \\ $$$$\:\:\:=\frac{\mathrm{2}}{\pi}\centerdot\frac{\pi}{\mathrm{2}}\centerdot\underset{\mathrm{r}=\mathrm{0}} {\overset{\mathrm{p}−\mathrm{1}} {\prod}}\frac{\mathrm{2p}−\left(\mathrm{2r}+\mathrm{1}\right)}{\mathrm{2p}−\mathrm{2r}}=\underset{\mathrm{r}=\mathrm{0}} {\overset{\mathrm{p}−\mathrm{1}} {\prod}}\frac{\mathrm{2p}−\left(\mathrm{2r}+\mathrm{1}\right)}{\mathrm{2p}−\mathrm{2r}} \\ $$
