Question and Answers Forum

All Questions      Topic List

None Questions

Previous in All Question      Next in All Question      

Previous in None      Next in None      

Question Number 118650 by Hassen_Timol last updated on 18/Oct/20

What is the (explicit) formula of this sequence ? 19, 151, 1021, 50389

$$\mathrm{What}\:\mathrm{is}\:\mathrm{the}\:\left(\mathrm{explicit}\right)\:\mathrm{formula}\:\mathrm{of}\:\mathrm{this}\:\mathrm{sequence}\:? \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\mathrm{19},\:\mathrm{151},\:\mathrm{1021},\:\mathrm{50389} \\ $$

Answered by MJS_new last updated on 18/Oct/20

zillions of possible formulas i.e. a_n =7 960n^3 −47 391n^2 +86 585n−47 135 b_n =((147 601 927 861n^3 )/(694 834 860n^3 −6 153 076 035n^2 +13 646 831 254n−420 067 560))

$$\mathrm{zillions}\:\mathrm{of}\:\mathrm{possible}\:\mathrm{formulas} \\ $$$$\mathrm{i}.\mathrm{e}. \\ $$$${a}_{{n}} =\mathrm{7}\:\mathrm{960}{n}^{\mathrm{3}} −\mathrm{47}\:\mathrm{391}{n}^{\mathrm{2}} +\mathrm{86}\:\mathrm{585}{n}−\mathrm{47}\:\mathrm{135} \\ $$$${b}_{{n}} =\frac{\mathrm{147}\:\mathrm{601}\:\mathrm{927}\:\mathrm{861}{n}^{\mathrm{3}} }{\mathrm{694}\:\mathrm{834}\:\mathrm{860}{n}^{\mathrm{3}} −\mathrm{6}\:\mathrm{153}\:\mathrm{076}\:\mathrm{035}{n}^{\mathrm{2}} +\mathrm{13}\:\mathrm{646}\:\mathrm{831}\:\mathrm{254}{n}−\mathrm{420}\:\mathrm{067}\:\mathrm{560}} \\ $$

Commented by Hassen_Timol last updated on 18/Oct/20

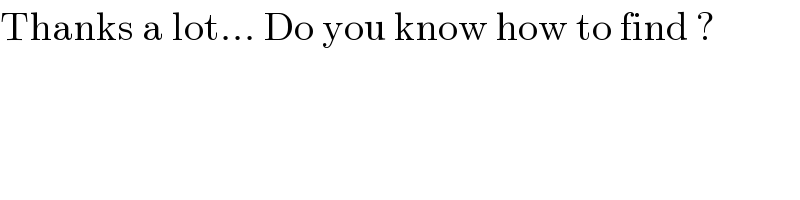
Thanks a lot... Do you know how to find ?

$$\mathrm{Thanks}\:\mathrm{a}\:\mathrm{lot}...\:\mathrm{Do}\:\mathrm{you}\:\mathrm{know}\:\mathrm{how}\:\mathrm{to}\:\mathrm{find}\:? \\ $$

Commented by MJS_new last updated on 19/Oct/20

the easiest and most usual is to find a polynome we′ve got 4 numbers y_1 , y_2 , y_3 , y_4 ⇒ polynome of degree 3 y_j =aj^3 +bj^2 +cj+d, j=1, 2, 3, 4 this leads to a linear system if 4 equations in 4 unknowns a, b, c, d. solve it and you′re done. in this case it leads to my first solution

$$\mathrm{the}\:\mathrm{easiest}\:\mathrm{and}\:\mathrm{most}\:\mathrm{usual}\:\mathrm{is}\:\mathrm{to}\:\mathrm{find}\:\mathrm{a} \\ $$$$\mathrm{polynome} \\ $$$$\mathrm{we}'\mathrm{ve}\:\mathrm{got}\:\mathrm{4}\:\mathrm{numbers}\:{y}_{\mathrm{1}} ,\:{y}_{\mathrm{2}} ,\:{y}_{\mathrm{3}} ,\:{y}_{\mathrm{4}} \\ $$$$\Rightarrow\:\mathrm{polynome}\:\mathrm{of}\:\mathrm{degree}\:\mathrm{3} \\ $$$${y}_{{j}} ={aj}^{\mathrm{3}} +{bj}^{\mathrm{2}} +{cj}+{d},\:{j}=\mathrm{1},\:\mathrm{2},\:\mathrm{3},\:\mathrm{4} \\ $$$$\mathrm{this}\:\mathrm{leads}\:\mathrm{to}\:\mathrm{a}\:\mathrm{linear}\:\mathrm{system}\:\mathrm{if}\:\mathrm{4}\:\mathrm{equations} \\ $$$$\mathrm{in}\:\mathrm{4}\:\mathrm{unknowns}\:{a},\:{b},\:{c},\:{d}.\:\mathrm{solve}\:\mathrm{it}\:\mathrm{and}\:\mathrm{you}'\mathrm{re} \\ $$$$\mathrm{done}.\:\mathrm{in}\:\mathrm{this}\:\mathrm{case}\:\mathrm{it}\:\mathrm{leads}\:\mathrm{to}\:\mathrm{my}\:\mathrm{first}\:\mathrm{solution} \\ $$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com