
Question Number 191731 by Spillover last updated on 29/Apr/23
![Use laws of algebra to prove the following (a)[(B−A)u(A−B)]=[(AuB)−(AnB)] (b)A▽(AnB)=A−B](Q191731.png)
$$\mathrm{Use}\:\mathrm{laws}\:\mathrm{of}\:\mathrm{algebra}\:\mathrm{to}\:\mathrm{prove}\:\mathrm{the}\:\mathrm{following} \\ $$$$\left(\mathrm{a}\right)\left[\left(\mathrm{B}−\mathrm{A}\right)\mathrm{u}\left(\mathrm{A}−\mathrm{B}\right)\right]=\left[\left(\mathrm{AuB}\right)−\left(\mathrm{AnB}\right)\right] \\ $$$$\left(\mathrm{b}\right)\mathrm{A}\bigtriangledown\left(\mathrm{AnB}\right)=\mathrm{A}−\mathrm{B} \\ $$
Answered by deleteduser1 last updated on 29/Apr/23
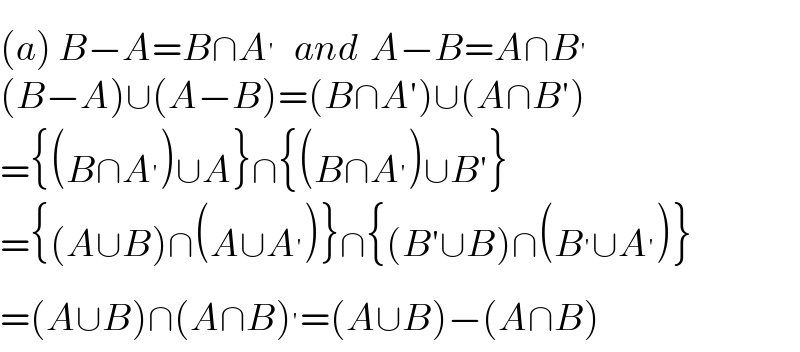
$$\left({a}\right)\:{B}−{A}={B}\cap{A}^{'} \:\:\:{and}\:\:{A}−{B}={A}\cap{B}^{'} \\ $$$$\left({B}−{A}\right)\cup\left({A}−{B}\right)=\left({B}\cap{A}'\right)\cup\left({A}\cap{B}'\right) \\ $$$$=\left\{\left({B}\cap{A}^{'} \right)\cup{A}\right\}\cap\left\{\left({B}\cap{A}^{'} \right)\cup{B}'\right\} \\ $$$$=\left\{\left({A}\cup{B}\right)\cap\left({A}\cup{A}^{'} \right)\right\}\cap\left\{\left({B}'\cup{B}\right)\cap\left({B}^{'} \cup{A}^{'} \right)\right\} \\ $$$$=\left({A}\cup{B}\right)\cap\left({A}\cap{B}\right)^{'} =\left({A}\cup{B}\right)−\left({A}\cap{B}\right) \\ $$
