Question and Answers Forum

All Questions      Topic List

Coordinate Geometry Questions

Previous in All Question      Next in All Question      

Previous in Coordinate Geometry      Next in Coordinate Geometry      

Question Number 191639 by a.lgnaoui last updated on 28/Apr/23

Une feuille de papier format A4 est plie n fois simultanement en longueur et largeur (total plie=2n) −Quel son les nouvelles dimensions de cette feuille la feuille a la fin . −quel seront ses dimensions apres 6 pliages −Quel seront les dimensions pour une feuille care de 30 cm plie 6 fois succrssivement en long et large

$$\mathrm{Une}\:\mathrm{feuille}\:\mathrm{de}\:\mathrm{papier}\:\mathrm{format}\:\boldsymbol{\mathrm{A}}\mathrm{4}\:\mathrm{est}\:\mathrm{plie} \\ $$$$\:\boldsymbol{\mathrm{n}}\:\mathrm{fois}\:\mathrm{simultanement}\:\mathrm{en}\:\mathrm{longueur}\:\mathrm{et} \\ $$$$\mathrm{largeur}\:\left(\mathrm{total}\:\mathrm{plie}=\mathrm{2n}\right) \\ $$$$−\mathrm{Quel}\:\mathrm{son}\:\mathrm{les}\:\mathrm{nouvelles}\:\mathrm{dimensions}\:\mathrm{de}\:\mathrm{cette}\:\mathrm{feuille} \\ $$$$\:\:\:\mathrm{la}\:\mathrm{feuille}\:\mathrm{a}\:\mathrm{la}\:\mathrm{fin}\:. \\ $$$$−\mathrm{quel}\:\mathrm{seront}\:\mathrm{ses}\:\mathrm{dimensions}\:\:\mathrm{apres}\: \\ $$$$\:\:\:\:\mathrm{6}\:\boldsymbol{\mathrm{pliages}} \\ $$$$−\mathrm{Quel}\:\mathrm{seront}\:\:\mathrm{les}\:\mathrm{dimensions}\:\mathrm{pour}\:\mathrm{une}\:\mathrm{feuille}\:\boldsymbol{\mathrm{care}}\:\mathrm{de}\:\mathrm{30}\:\boldsymbol{\mathrm{cm}}\: \\ $$$$\:\:\:\mathrm{plie}\:\mathrm{6}\:\boldsymbol{\mathrm{fois}}\:\:\mathrm{succrssivement}\:\mathrm{en}\:\mathrm{long}\:\:\mathrm{et}\:\mathrm{large} \\ $$$$ \\ $$

Commented by a.lgnaoui last updated on 28/Apr/23

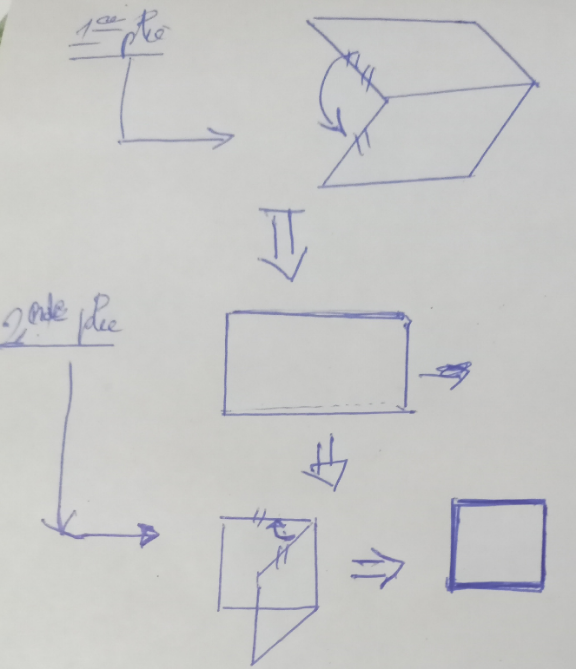
Answered by a.lgnaoui last updated on 29/Apr/23

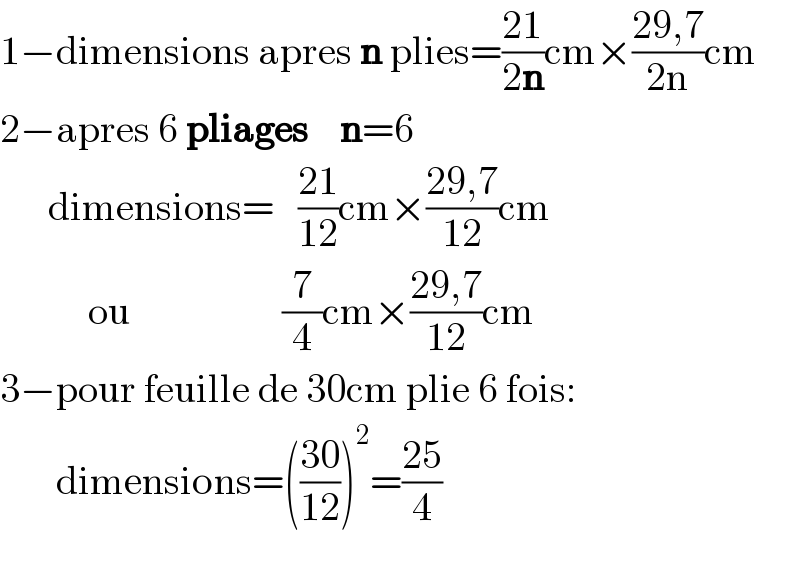
1−dimensions apres n plies=((21)/(2n))cm×((29,7)/(2n))cm 2−apres 6 pliages n=6 dimensions= ((21)/(12))cm×((29,7)/(12))cm ou (7/4)cm×((29,7)/(12))cm 3−pour feuille de 30cm plie 6 fois: dimensions=(((30)/(12)))^2 =((25)/4)

$$\mathrm{1}−\mathrm{dimensions}\:\mathrm{apres}\:\boldsymbol{\mathrm{n}}\:\mathrm{plies}=\frac{\mathrm{21}}{\mathrm{2}\boldsymbol{\mathrm{n}}}\mathrm{cm}×\frac{\mathrm{29},\mathrm{7}}{\mathrm{2n}}\mathrm{cm} \\ $$$$\mathrm{2}−\mathrm{apres}\:\mathrm{6}\:\boldsymbol{\mathrm{pliages}}\:\:\:\:\boldsymbol{\mathrm{n}}=\mathrm{6}\:\:\:\: \\ $$$$\:\:\:\:\:\:\mathrm{dimensions}=\:\:\:\frac{\mathrm{21}}{\mathrm{12}}\mathrm{cm}×\frac{\mathrm{29},\mathrm{7}}{\mathrm{12}}\mathrm{cm} \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\mathrm{ou}\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\frac{\mathrm{7}}{\mathrm{4}}\mathrm{cm}×\frac{\mathrm{29},\mathrm{7}}{\mathrm{12}}\mathrm{cm} \\ $$$$\mathrm{3}−\mathrm{pour}\:\mathrm{feuille}\:\mathrm{de}\:\mathrm{30cm}\:\mathrm{plie}\:\mathrm{6}\:\mathrm{fois}: \\ $$$$\:\:\:\:\:\:\:\mathrm{dimensions}=\left(\frac{\mathrm{30}}{\mathrm{12}}\right)^{\mathrm{2}} =\frac{\mathrm{25}}{\mathrm{4}} \\ $$$$ \\ $$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com