Question and Answers Forum

All Questions      Topic List

Coordinate Geometry Questions

Previous in All Question      Next in All Question      

Previous in Coordinate Geometry      Next in Coordinate Geometry      

Question Number 62753 by peter frank last updated on 24/Jun/19

The normal at the point P(4cos θ,3sin θ) on the ellipse (x^2 /(16)) +(y^2 /9)=1 meets the x−axis and y−axis at A and B respectively show that locus of the mid−point of AB is an ellipse with the same eccentricity as given ellipse.

$${The}\:{normal}\:{at}\:{the}\:{point} \\ $$$${P}\left(\mathrm{4cos}\:\theta,\mathrm{3sin}\:\theta\right)\:{on}\:{the} \\ $$$${ellipse}\:\frac{{x}^{\mathrm{2}} }{\mathrm{16}}\:+\frac{{y}^{\mathrm{2}} }{\mathrm{9}}=\mathrm{1}\:{meets} \\ $$$${the}\:{x}−{axis}\:{and}\:{y}−{axis} \\ $$$${at}\:{A}\:{and}\:{B}\:{respectively} \\ $$$${show}\:{that}\:{locus}\:{of}\:{the} \\ $$$${mid}−{point}\:{of}\:{AB}\:{is}\:{an} \\ $$$${ellipse}\:{with}\:{the}\:{same} \\ $$$${eccentricity}\:{as}\:{given} \\ $$$${ellipse}. \\ $$

Answered by Hope last updated on 25/Jun/19

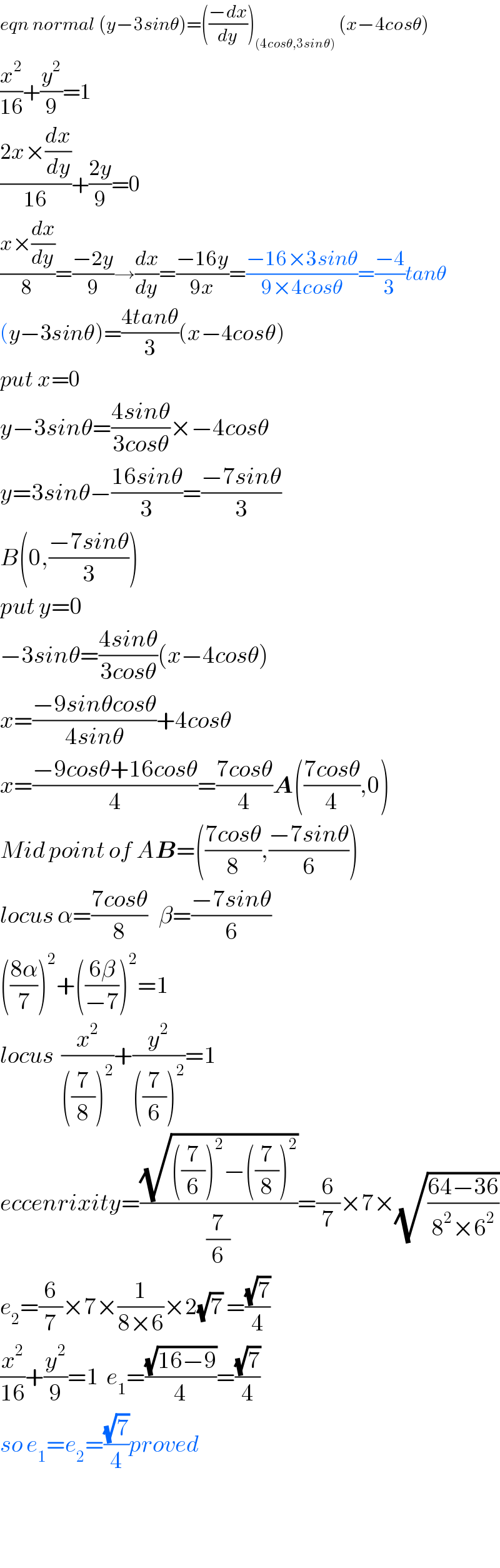
eqn normal (y−3sinθ)=(((−dx)/dy))_((4cosθ,3sinθ)) (x−4cosθ) (x^2 /(16))+(y^2 /9)=1 ((2x×(dx/dy))/(16))+((2y)/9)=0 ((x×(dx/dy))/8)=((−2y)/9)→(dx/dy)=((−16y)/(9x))=((−16×3sinθ)/(9×4cosθ))=((−4)/3)tanθ (y−3sinθ)=((4tanθ)/3)(x−4cosθ) put x=0 y−3sinθ=((4sinθ)/(3cosθ))×−4cosθ y=3sinθ−((16sinθ)/3)=((−7sinθ)/3) B(0,((−7sinθ)/3)) put y=0 −3sinθ=((4sinθ)/(3cosθ))(x−4cosθ) x=((−9sinθcosθ)/(4sinθ))+4cosθ x=((−9cosθ+16cosθ)/4)=((7cosθ)/4)A(((7cosθ)/4),0) Mid point of AB=(((7cosθ)/8),((−7sinθ)/6)) locus α=((7cosθ)/8) β=((−7sinθ)/6) (((8α)/7))^2 +(((6β)/(−7)))^2 =1 locus (x^2 /(((7/8))^2 ))+(y^2 /(((7/6))^2 ))=1 eccenrixity=((√(((7/6))^2 −((7/8))^2 ))/(7/6))=(6/7)×7×(√((64−36)/(8^2 ×6^2 ))) e_2 =(6/7)×7×(1/(8×6))×2(√7) =((√7)/4) (x^2 /(16))+(y^2 /9)=1 e_1 =((√(16−9))/4)=((√7)/4) so e_1 =e_2 =((√7)/4)proved

$${eqn}\:{normal}\:\left({y}−\mathrm{3}{sin}\theta\right)=\left(\frac{−{dx}}{{dy}}\right)_{\left(\mathrm{4}{cos}\theta,\mathrm{3}{sin}\theta\right)} \:\left({x}−\mathrm{4}{cos}\theta\right) \\ $$$$\frac{{x}^{\mathrm{2}} }{\mathrm{16}}+\frac{{y}^{\mathrm{2}} }{\mathrm{9}}=\mathrm{1} \\ $$$$\frac{\mathrm{2}{x}×\frac{{dx}}{{dy}}}{\mathrm{16}}+\frac{\mathrm{2}{y}}{\mathrm{9}}=\mathrm{0} \\ $$$$\frac{{x}×\frac{{dx}}{{dy}}}{\mathrm{8}}=\frac{−\mathrm{2}{y}}{\mathrm{9}}\rightarrow\frac{{dx}}{{dy}}=\frac{−\mathrm{16}{y}}{\mathrm{9}{x}}=\frac{−\mathrm{16}×\mathrm{3}{sin}\theta}{\mathrm{9}×\mathrm{4}{cos}\theta}=\frac{−\mathrm{4}}{\mathrm{3}}{tan}\theta \\ $$$$\left({y}−\mathrm{3}{sin}\theta\right)=\frac{\mathrm{4}{tan}\theta}{\mathrm{3}}\left({x}−\mathrm{4}{cos}\theta\right) \\ $$$${put}\:{x}=\mathrm{0} \\ $$$${y}−\mathrm{3}{sin}\theta=\frac{\mathrm{4}{sin}\theta}{\mathrm{3}{cos}\theta}×−\mathrm{4}{cos}\theta \\ $$$${y}=\mathrm{3}{sin}\theta−\frac{\mathrm{16}{sin}\theta}{\mathrm{3}}=\frac{−\mathrm{7}{sin}\theta}{\mathrm{3}} \\ $$$${B}\left(\mathrm{0},\frac{−\mathrm{7}{sin}\theta}{\mathrm{3}}\right) \\ $$$${put}\:{y}=\mathrm{0} \\ $$$$−\mathrm{3}{sin}\theta=\frac{\mathrm{4}{sin}\theta}{\mathrm{3}{cos}\theta}\left({x}−\mathrm{4}{cos}\theta\right) \\ $$$${x}=\frac{−\mathrm{9}{sin}\theta{cos}\theta}{\mathrm{4}{sin}\theta}+\mathrm{4}{cos}\theta \\ $$$${x}=\frac{−\mathrm{9}{cos}\theta+\mathrm{16}{cos}\theta}{\mathrm{4}}=\frac{\mathrm{7}{cos}\theta}{\mathrm{4}}\boldsymbol{{A}}\left(\frac{\mathrm{7}{cos}\theta}{\mathrm{4}},\mathrm{0}\right) \\ $$$${Mid}\:{point}\:{of}\:{A}\boldsymbol{{B}}=\left(\frac{\mathrm{7}{cos}\theta}{\mathrm{8}},\frac{−\mathrm{7}{sin}\theta}{\mathrm{6}}\right) \\ $$$${locus}\:\alpha=\frac{\mathrm{7}{cos}\theta}{\mathrm{8}}\:\:\:\beta=\frac{−\mathrm{7}{sin}\theta}{\mathrm{6}} \\ $$$$\left(\frac{\mathrm{8}\alpha}{\mathrm{7}}\right)^{\mathrm{2}} +\left(\frac{\mathrm{6}\beta}{−\mathrm{7}}\right)^{\mathrm{2}} =\mathrm{1} \\ $$$${locus}\:\:\frac{{x}^{\mathrm{2}} }{\left(\frac{\mathrm{7}}{\mathrm{8}}\right)^{\mathrm{2}} }+\frac{{y}^{\mathrm{2}} }{\left(\frac{\mathrm{7}}{\mathrm{6}}\right)^{\mathrm{2}} }=\mathrm{1} \\ $$$${eccenrixity}=\frac{\sqrt{\left(\frac{\mathrm{7}}{\mathrm{6}}\right)^{\mathrm{2}} −\left(\frac{\mathrm{7}}{\mathrm{8}}\right)^{\mathrm{2}} }}{\frac{\mathrm{7}}{\mathrm{6}}}=\frac{\mathrm{6}}{\mathrm{7}}×\mathrm{7}×\sqrt{\frac{\mathrm{64}−\mathrm{36}}{\mathrm{8}^{\mathrm{2}} ×\mathrm{6}^{\mathrm{2}} }} \\ $$$${e}_{\mathrm{2}} =\frac{\mathrm{6}}{\mathrm{7}}×\mathrm{7}×\frac{\mathrm{1}}{\mathrm{8}×\mathrm{6}}×\mathrm{2}\sqrt{\mathrm{7}}\:=\frac{\sqrt{\mathrm{7}}}{\mathrm{4}} \\ $$$$\frac{{x}^{\mathrm{2}} }{\mathrm{16}}+\frac{{y}^{\mathrm{2}} }{\mathrm{9}}=\mathrm{1}\:\:{e}_{\mathrm{1}} =\frac{\sqrt{\mathrm{16}−\mathrm{9}}}{\mathrm{4}}=\frac{\sqrt{\mathrm{7}}}{\mathrm{4}} \\ $$$${so}\:{e}_{\mathrm{1}} ={e}_{\mathrm{2}} =\frac{\sqrt{\mathrm{7}}}{\mathrm{4}}{proved} \\ $$$$ \\ $$$$ \\ $$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com