
Question Number 52124 by 786786AM last updated on 03/Jan/19

$$\mathrm{The}\:\mathrm{curve}\:\mathrm{y}\:=\:\mathrm{ax}\:+\:\frac{\mathrm{b}}{\mathrm{2x}−\:\mathrm{1}}\:\mathrm{has}\:\mathrm{the}\:\mathrm{stationary}\:\mathrm{point}\:\mathrm{at}\:\:\left(\mathrm{2},\:\mathrm{7}\right)\:.\:\mathrm{Find}\:\mathrm{the}\:\mathrm{value}\:\mathrm{of}\:\mathrm{a}\:\mathrm{and}\:\mathrm{b}\:. \\ $$
Answered by tanmay.chaudhury50@gmail.com last updated on 03/Jan/19
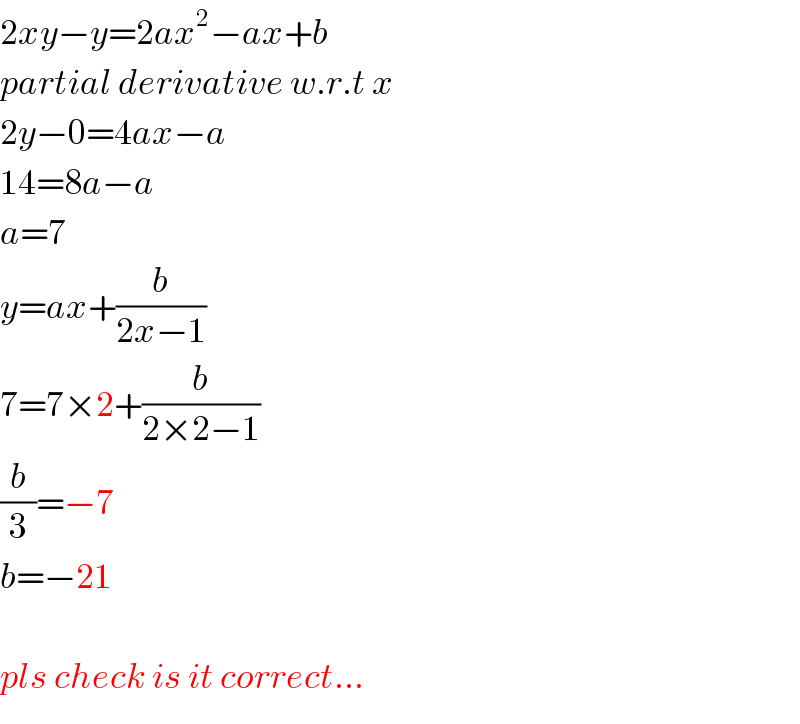
$$\mathrm{2}{xy}−{y}=\mathrm{2}{ax}^{\mathrm{2}} −{ax}+{b} \\ $$$${partial}\:{derivative}\:{w}.{r}.{t}\:{x} \\ $$$$\mathrm{2}{y}−\mathrm{0}=\mathrm{4}{ax}−{a} \\ $$$$\mathrm{14}=\mathrm{8}{a}−{a} \\ $$$${a}=\mathrm{7} \\ $$$${y}={ax}+\frac{{b}}{\mathrm{2}{x}−\mathrm{1}} \\ $$$$\mathrm{7}=\mathrm{7}×\mathrm{2}+\frac{{b}}{\mathrm{2}×\mathrm{2}−\mathrm{1}} \\ $$$$\frac{{b}}{\mathrm{3}}=−\mathrm{7} \\ $$$${b}=−\mathrm{21} \\ $$$$ \\ $$$${pls}\:{check}\:{is}\:{it}\:{correct}... \\ $$
