
Question Number 217424 by peter frank last updated on 13/Mar/25

The angle of elevation of the top of a building 24 m high is observed from the top and from the bottom of a vertical ladder, and found to be 45° and 60° respectively. Find the height of the ladder.
Answered by mr W last updated on 13/Mar/25
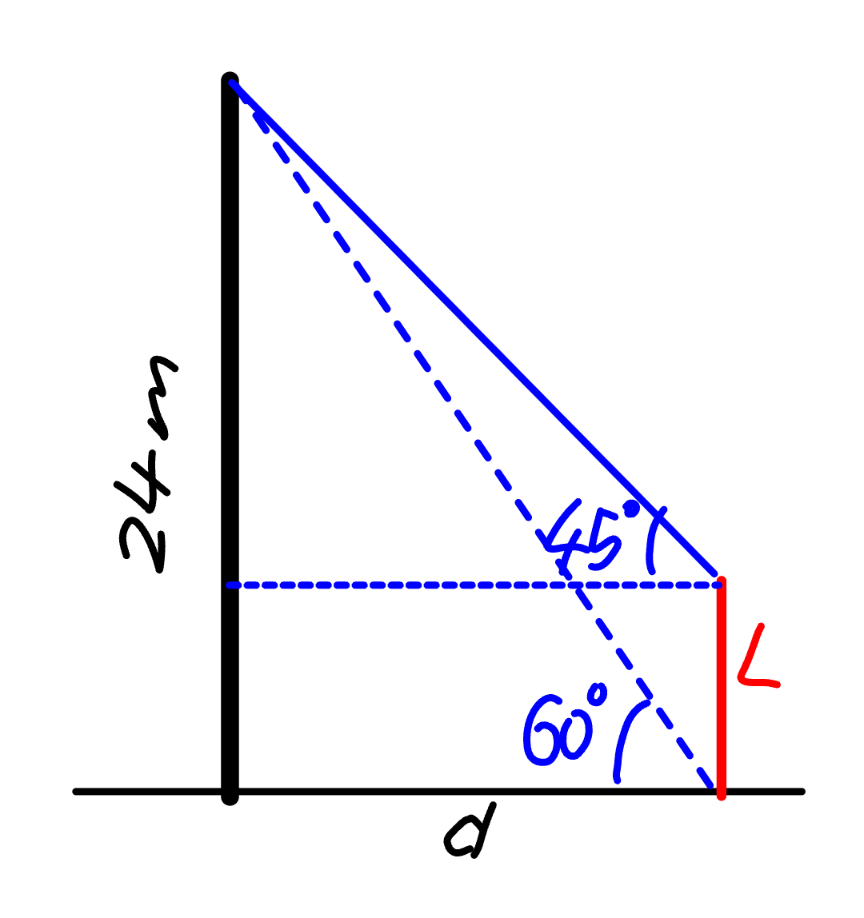
Commented by mr W last updated on 13/Mar/25
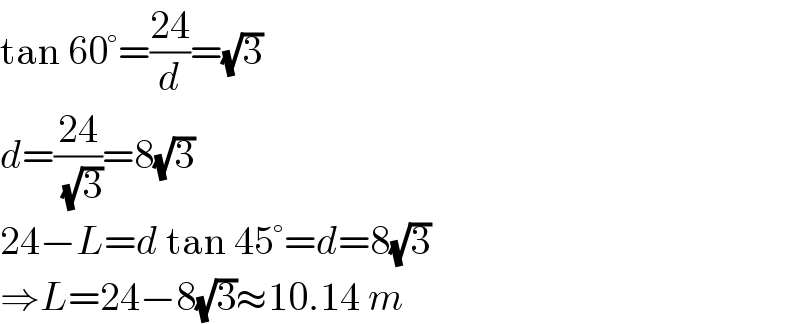
$$\mathrm{tan}\:\mathrm{60}°=\frac{\mathrm{24}}{{d}}=\sqrt{\mathrm{3}} \\ $$$${d}=\frac{\mathrm{24}}{\:\sqrt{\mathrm{3}}}=\mathrm{8}\sqrt{\mathrm{3}} \\ $$$$\mathrm{24}−{L}={d}\:\mathrm{tan}\:\mathrm{45}°={d}=\mathrm{8}\sqrt{\mathrm{3}} \\ $$$$\Rightarrow{L}=\mathrm{24}−\mathrm{8}\sqrt{\mathrm{3}}\approx\mathrm{10}.\mathrm{14}\:{m} \\ $$
Commented by peter frank last updated on 14/Mar/25

$$\mathrm{thank}\:\mathrm{you} \\ $$
Answered by Spillover last updated on 13/Mar/25
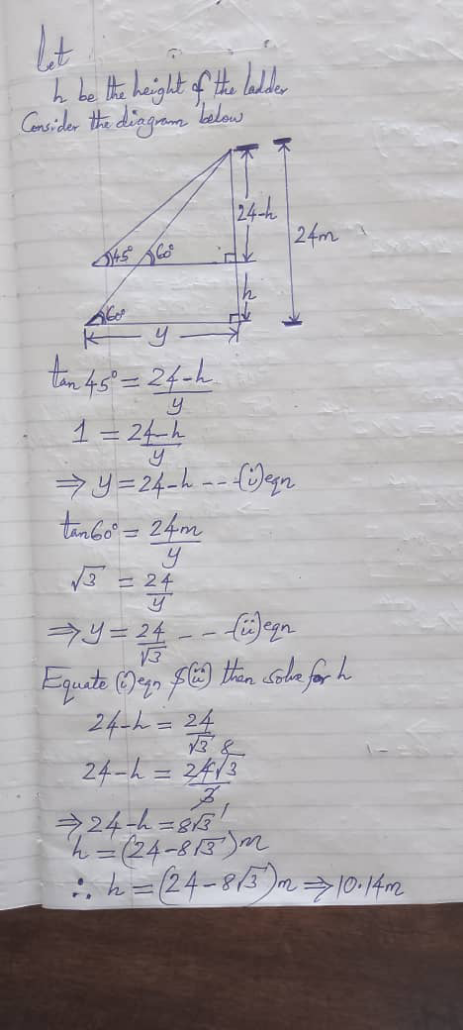
Commented by peter frank last updated on 14/Mar/25

$$\mathrm{thank}\:\mathrm{you} \\ $$
