Question and Answers Forum

All Questions      Topic List

Differential Equation Questions

Previous in All Question      Next in All Question      

Previous in Differential Equation      Next in Differential Equation      

Question Number 196199 by Frix last updated on 19/Aug/23

Solve: y′′′=((3y′(y′′)^2 )/(1+(y′)^2 ))

$$\mathrm{Solve}: \\ $$$${y}'''=\frac{\mathrm{3}{y}'\left({y}''\right)^{\mathrm{2}} }{\mathrm{1}+\left({y}'\right)^{\mathrm{2}} } \\ $$

Answered by aleks041103 last updated on 20/Aug/23

(ln(y′′))′=((y′′′)/(y′′))=((3y′y′′)/(1+(y′)^2 ))=(3/2) ((((y′)^2 )′)/(1+(y′)^2 ))=(3/2)(ln(1+(y′)^2 ))′ ⇒ln(y′′)−ln(A)=(3/2)ln(1+(y′)^2 ) y′′=A(1+(y′)^2 )^(3/2) ⇒(((y′)′)/((1+(y′)^2 )^(3/2) ))=A ∫(dx/((1+x^2 )^(3/2) ))=∫((d(sinh(t)))/((1+sinh^2 t)^(3/2) ))=∫ ((cosh(t)dt)/(cosh^3 t))= =∫sech^2 (t)dt=tanh(t)+C=((sinh(t))/( (√(1+sinh^2 (t)))))+C=(x/( (√(1+x^2 ))))+C ⇒(((y′)/( (√(1+(y′)^2 )))))′=A ⇒((y′)/( (√(1+(y′)^2 ))))=Ax+B=(z/( (√(1+z^2 ))))=F (z^2 /(1+z^2 ))=F^2 ⇒(1−F^2 )z^2 =F^2 ⇒z=±(F/( (√(1−F^2 )))) ⇒y′=± ((Ax+B)/( (√(1−(Ax+B)^2 )))) ⇒y(x)=C±∫((Ax+B)/( (√(1−(Ax+B)^2 ))))dx y=C±(1/A)∫((zdz)/( (√(1−z^2 )))) , z=Ax+B y=C∓(1/A)∫((d(1−z^2 ))/(2(√(1−z^2 ))))=C∓((√(1−(Ax+B)^2 ))/A) ⇒y(x)=C±(1/A)(√(1−(Ax+B)^2 ))

$$\left({ln}\left({y}''\right)\right)'=\frac{{y}'''}{{y}''}=\frac{\mathrm{3}{y}'{y}''}{\mathrm{1}+\left({y}'\right)^{\mathrm{2}} }=\frac{\mathrm{3}}{\mathrm{2}}\:\frac{\left(\left({y}'\right)^{\mathrm{2}} \right)'}{\mathrm{1}+\left({y}'\right)^{\mathrm{2}} }=\frac{\mathrm{3}}{\mathrm{2}}\left({ln}\left(\mathrm{1}+\left({y}'\right)^{\mathrm{2}} \right)\right)' \\ $$$$\Rightarrow{ln}\left({y}''\right)−{ln}\left({A}\right)=\frac{\mathrm{3}}{\mathrm{2}}{ln}\left(\mathrm{1}+\left({y}'\right)^{\mathrm{2}} \right) \\ $$$${y}''={A}\left(\mathrm{1}+\left({y}'\right)^{\mathrm{2}} \right)^{\mathrm{3}/\mathrm{2}} \\ $$$$\Rightarrow\frac{\left({y}'\right)'}{\left(\mathrm{1}+\left({y}'\right)^{\mathrm{2}} \right)^{\mathrm{3}/\mathrm{2}} }={A} \\ $$$$\int\frac{{dx}}{\left(\mathrm{1}+{x}^{\mathrm{2}} \right)^{\mathrm{3}/\mathrm{2}} }=\int\frac{{d}\left({sinh}\left({t}\right)\right)}{\left(\mathrm{1}+{sinh}^{\mathrm{2}} {t}\right)^{\mathrm{3}/\mathrm{2}} }=\int\:\frac{{cosh}\left({t}\right){dt}}{{cosh}^{\mathrm{3}} {t}}= \\ $$$$=\int{sech}^{\mathrm{2}} \left({t}\right){dt}={tanh}\left({t}\right)+{C}=\frac{{sinh}\left({t}\right)}{\:\sqrt{\mathrm{1}+{sinh}^{\mathrm{2}} \left({t}\right)}}+{C}=\frac{{x}}{\:\sqrt{\mathrm{1}+{x}^{\mathrm{2}} }}+{C} \\ $$$$\Rightarrow\left(\frac{{y}'}{\:\sqrt{\mathrm{1}+\left({y}'\right)^{\mathrm{2}} }}\right)'={A} \\ $$$$\Rightarrow\frac{{y}'}{\:\sqrt{\mathrm{1}+\left({y}'\right)^{\mathrm{2}} }}={Ax}+{B}=\frac{{z}}{\:\sqrt{\mathrm{1}+{z}^{\mathrm{2}} }}={F} \\ $$$$\frac{{z}^{\mathrm{2}} }{\mathrm{1}+{z}^{\mathrm{2}} }={F}^{\mathrm{2}} \Rightarrow\left(\mathrm{1}−{F}^{\mathrm{2}} \right){z}^{\mathrm{2}} ={F}^{\mathrm{2}} \Rightarrow{z}=\pm\frac{{F}}{\:\sqrt{\mathrm{1}−{F}^{\mathrm{2}} }} \\ $$$$\Rightarrow{y}'=\pm\:\frac{{Ax}+{B}}{\:\sqrt{\mathrm{1}−\left({Ax}+{B}\right)^{\mathrm{2}} }} \\ $$$$\Rightarrow{y}\left({x}\right)={C}\pm\int\frac{{Ax}+{B}}{\:\sqrt{\mathrm{1}−\left({Ax}+{B}\right)^{\mathrm{2}} }}{dx} \\ $$$${y}={C}\pm\frac{\mathrm{1}}{{A}}\int\frac{{zdz}}{\:\sqrt{\mathrm{1}−{z}^{\mathrm{2}} }}\:,\:\:{z}={Ax}+{B} \\ $$$${y}={C}\mp\frac{\mathrm{1}}{{A}}\int\frac{{d}\left(\mathrm{1}−{z}^{\mathrm{2}} \right)}{\mathrm{2}\sqrt{\mathrm{1}−{z}^{\mathrm{2}} }}={C}\mp\frac{\sqrt{\mathrm{1}−\left({Ax}+{B}\right)^{\mathrm{2}} }}{{A}} \\ $$$$\Rightarrow{y}\left({x}\right)={C}\pm\frac{\mathrm{1}}{{A}}\sqrt{\mathrm{1}−\left({Ax}+{B}\right)^{\mathrm{2}} } \\ $$$$ \\ $$

Commented by aleks041103 last updated on 20/Aug/23

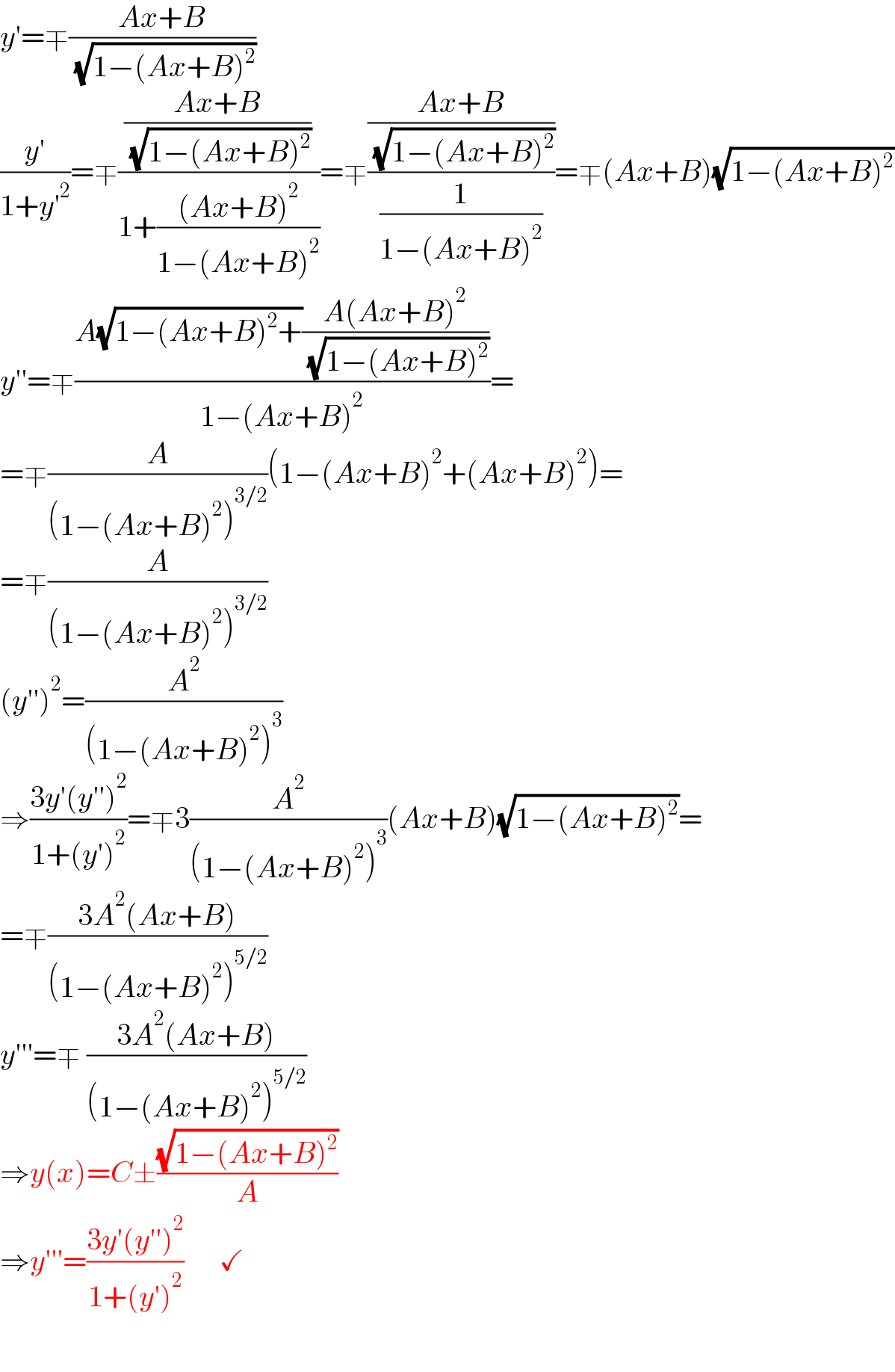
y′=∓((Ax+B)/( (√(1−(Ax+B)^2 )))) ((y′)/(1+y′^2 ))=∓(((Ax+B)/( (√(1−(Ax+B)^2 ))))/(1+(((Ax+B)^2 )/(1−(Ax+B)^2 ))))=∓(((Ax+B)/( (√(1−(Ax+B)^2 ))))/(1/(1−(Ax+B)^2 )))=∓(Ax+B)(√(1−(Ax+B)^2 )) y′′=∓((A(√(1−(Ax+B)^2 +))((A(Ax+B)^2 )/( (√(1−(Ax+B)^2 )))))/(1−(Ax+B)^2 ))= =∓(A/((1−(Ax+B)^2 )^(3/2) ))(1−(Ax+B)^2 +(Ax+B)^2 )= =∓(A/((1−(Ax+B)^2 )^(3/2) )) (y′′)^2 =(A^2 /((1−(Ax+B)^2 )^3 )) ⇒((3y′(y′′)^2 )/(1+(y′)^2 ))=∓3(A^2 /((1−(Ax+B)^2 )^3 ))(Ax+B)(√(1−(Ax+B)^2 ))= =∓((3A^2 (Ax+B))/((1−(Ax+B)^2 )^(5/2) )) y′′′=∓ ((3A^2 (Ax+B))/((1−(Ax+B)^2 )^(5/2) )) ⇒y(x)=C±((√(1−(Ax+B)^2 ))/A) ⇒y′′′=((3y′(y′′)^2 )/(1+(y′)^2 )) ✓

$${y}'=\mp\frac{{Ax}+{B}}{\:\sqrt{\mathrm{1}−\left({Ax}+{B}\right)^{\mathrm{2}} }} \\ $$$$\frac{{y}'}{\mathrm{1}+{y}'^{\mathrm{2}} }=\mp\frac{\frac{{Ax}+{B}}{\:\sqrt{\mathrm{1}−\left({Ax}+{B}\right)^{\mathrm{2}} }}}{\mathrm{1}+\frac{\left({Ax}+{B}\right)^{\mathrm{2}} }{\mathrm{1}−\left({Ax}+{B}\right)^{\mathrm{2}} }}=\mp\frac{\frac{{Ax}+{B}}{\:\sqrt{\mathrm{1}−\left({Ax}+{B}\right)^{\mathrm{2}} }}}{\frac{\mathrm{1}}{\mathrm{1}−\left({Ax}+{B}\right)^{\mathrm{2}} }}=\mp\left({Ax}+{B}\right)\sqrt{\mathrm{1}−\left({Ax}+{B}\right)^{\mathrm{2}} } \\ $$$${y}''=\mp\frac{{A}\sqrt{\mathrm{1}−\left({Ax}+{B}\right)^{\mathrm{2}} +}\frac{{A}\left({Ax}+{B}\right)^{\mathrm{2}} }{\:\sqrt{\mathrm{1}−\left({Ax}+{B}\right)^{\mathrm{2}} }}}{\mathrm{1}−\left({Ax}+{B}\right)^{\mathrm{2}} }= \\ $$$$=\mp\frac{{A}}{\left(\mathrm{1}−\left({Ax}+{B}\right)^{\mathrm{2}} \right)^{\mathrm{3}/\mathrm{2}} }\left(\mathrm{1}−\left({Ax}+{B}\right)^{\mathrm{2}} +\left({Ax}+{B}\right)^{\mathrm{2}} \right)= \\ $$$$=\mp\frac{{A}}{\left(\mathrm{1}−\left({Ax}+{B}\right)^{\mathrm{2}} \right)^{\mathrm{3}/\mathrm{2}} } \\ $$$$\left({y}''\right)^{\mathrm{2}} =\frac{{A}^{\mathrm{2}} }{\left(\mathrm{1}−\left({Ax}+{B}\right)^{\mathrm{2}} \right)^{\mathrm{3}} } \\ $$$$\Rightarrow\frac{\mathrm{3}{y}'\left({y}''\right)^{\mathrm{2}} }{\mathrm{1}+\left({y}'\right)^{\mathrm{2}} }=\mp\mathrm{3}\frac{{A}^{\mathrm{2}} }{\left(\mathrm{1}−\left({Ax}+{B}\right)^{\mathrm{2}} \right)^{\mathrm{3}} }\left({Ax}+{B}\right)\sqrt{\mathrm{1}−\left({Ax}+{B}\right)^{\mathrm{2}} }= \\ $$$$=\mp\frac{\mathrm{3}{A}^{\mathrm{2}} \left({Ax}+{B}\right)}{\left(\mathrm{1}−\left({Ax}+{B}\right)^{\mathrm{2}} \right)^{\mathrm{5}/\mathrm{2}} } \\ $$$${y}'''=\mp\:\frac{\mathrm{3}{A}^{\mathrm{2}} \left({Ax}+{B}\right)}{\left(\mathrm{1}−\left({Ax}+{B}\right)^{\mathrm{2}} \right)^{\mathrm{5}/\mathrm{2}} } \\ $$$$\Rightarrow{y}\left({x}\right)={C}\pm\frac{\sqrt{\mathrm{1}−\left({Ax}+{B}\right)^{\mathrm{2}} }}{{A}} \\ $$$$\Rightarrow{y}'''=\frac{\mathrm{3}{y}'\left({y}''\right)^{\mathrm{2}} }{\mathrm{1}+\left({y}'\right)^{\mathrm{2}} }\:\:\:\:\:\:\checkmark \\ $$$$ \\ $$

Commented by mr W last updated on 20/Aug/23

great solution!

$${great}\:{solution}! \\ $$

Commented by Frix last updated on 20/Aug/23

Thank you!

$$\mathrm{Thank}\:\mathrm{you}! \\ $$

Commented by aleks041103 last updated on 20/Aug/23

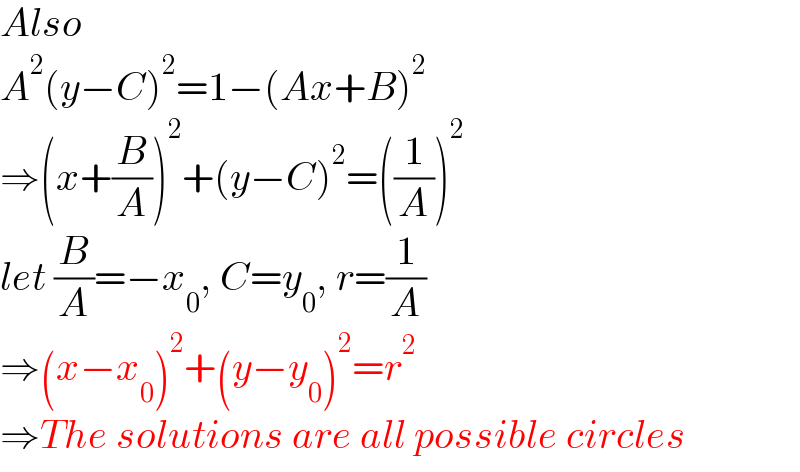
Also A^2 (y−C)^2 =1−(Ax+B)^2 ⇒(x+(B/A))^2 +(y−C)^2 =((1/A))^2 let (B/A)=−x_0 , C=y_0 , r=(1/A) ⇒(x−x_0 )^2 +(y−y_0 )^2 =r^2 ⇒The solutions are all possible circles

$${Also} \\ $$$${A}^{\mathrm{2}} \left({y}−{C}\right)^{\mathrm{2}} =\mathrm{1}−\left({Ax}+{B}\right)^{\mathrm{2}} \\ $$$$\Rightarrow\left({x}+\frac{{B}}{{A}}\right)^{\mathrm{2}} +\left({y}−{C}\right)^{\mathrm{2}} =\left(\frac{\mathrm{1}}{{A}}\right)^{\mathrm{2}} \\ $$$${let}\:\frac{{B}}{{A}}=−{x}_{\mathrm{0}} ,\:{C}={y}_{\mathrm{0}} ,\:{r}=\frac{\mathrm{1}}{{A}} \\ $$$$\Rightarrow\left({x}−{x}_{\mathrm{0}} \right)^{\mathrm{2}} +\left({y}−{y}_{\mathrm{0}} \right)^{\mathrm{2}} ={r}^{\mathrm{2}} \\ $$$$\Rightarrow{The}\:{solutions}\:{are}\:{all}\:{possible}\:{circles} \\ $$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com