
Question Number 104034 by mohammad17 last updated on 19/Jul/20

$${Solve}:\:{y}^{''} +\mathrm{2}{y}^{'} +\mathrm{2}{y}={secax} \\ $$
Answered by bramlex last updated on 19/Jul/20
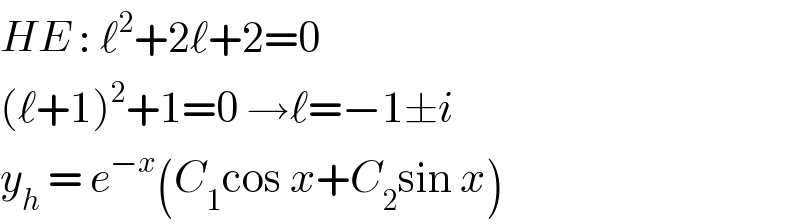
$${HE}\::\:\ell^{\mathrm{2}} +\mathrm{2}\ell+\mathrm{2}=\mathrm{0} \\ $$$$\left(\ell+\mathrm{1}\right)^{\mathrm{2}} +\mathrm{1}=\mathrm{0}\:\rightarrow\ell=−\mathrm{1}\pm{i}\: \\ $$$${y}_{{h}} \:=\:{e}^{−{x}} \left({C}_{\mathrm{1}} \mathrm{cos}\:{x}+{C}_{\mathrm{2}} \mathrm{sin}\:{x}\right) \\ $$
Commented by mohammad17 last updated on 19/Jul/20

$${sir}\:{i}\:{want}\:{yp}\:? \\ $$
Commented by bobhans last updated on 19/Jul/20
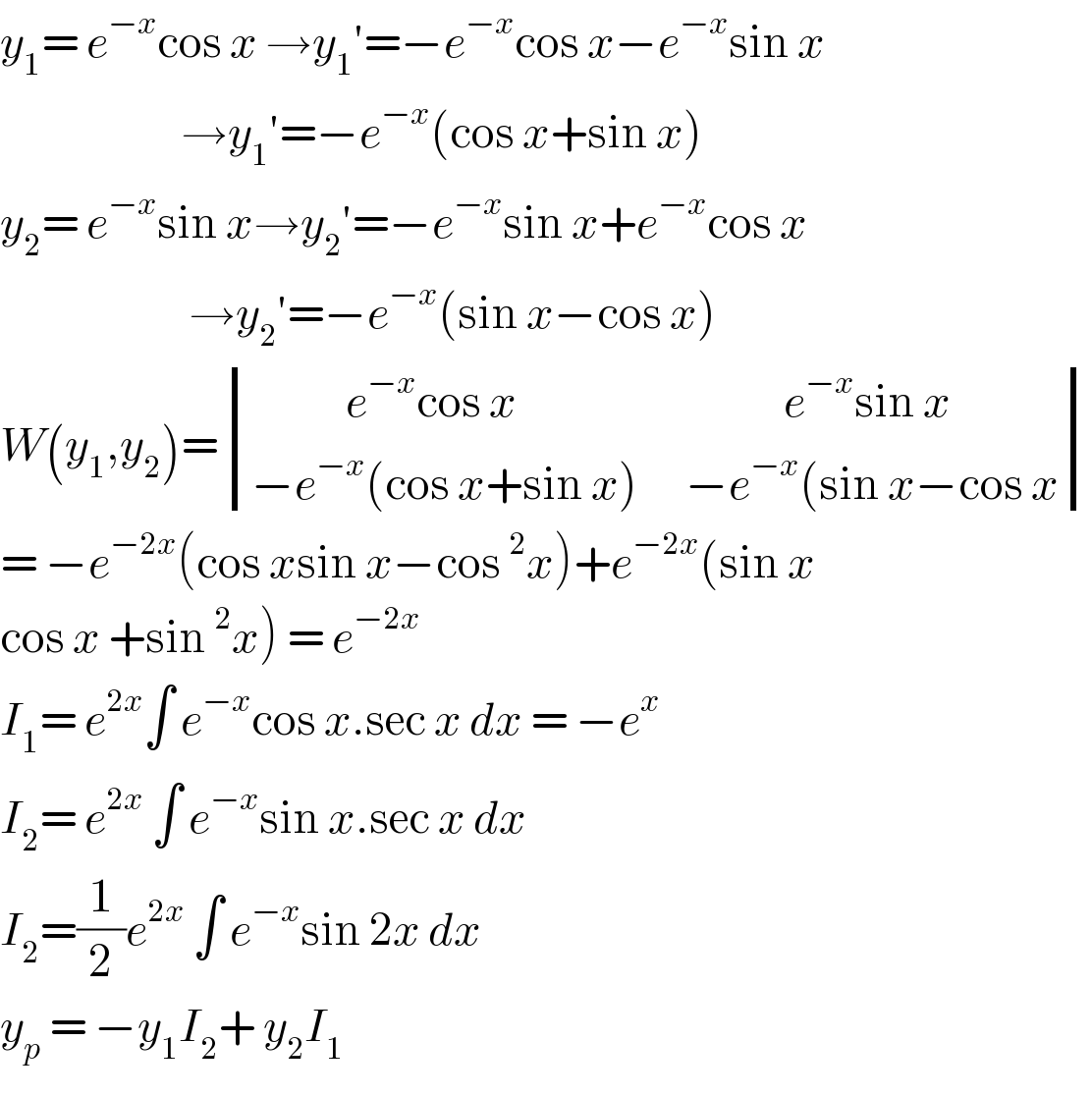
$${y}_{\mathrm{1}} =\:{e}^{−{x}} \mathrm{cos}\:{x}\:\rightarrow{y}_{\mathrm{1}} '=−{e}^{−{x}} \mathrm{cos}\:{x}−{e}^{−{x}} \mathrm{sin}\:{x} \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\rightarrow{y}_{\mathrm{1}} '=−{e}^{−{x}} \left(\mathrm{cos}\:{x}+\mathrm{sin}\:{x}\right) \\ $$$${y}_{\mathrm{2}} =\:{e}^{−{x}} \mathrm{sin}\:{x}\rightarrow{y}_{\mathrm{2}} '=−{e}^{−{x}} \mathrm{sin}\:{x}+{e}^{−{x}} \mathrm{cos}\:{x} \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\rightarrow{y}_{\mathrm{2}} '=−{e}^{−{x}} \left(\mathrm{sin}\:{x}−\mathrm{cos}\:{x}\right) \\ $$$${W}\left({y}_{\mathrm{1}} ,{y}_{\mathrm{2}} \right)=\begin{vmatrix}{\:\:\:\:\:\:\:\:\:\:\:\:{e}^{−{x}} \mathrm{cos}\:{x}\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:{e}^{−{x}} \mathrm{sin}\:{x}}\\{−{e}^{−{x}} \left(\mathrm{cos}\:{x}+\mathrm{sin}\:{x}\right)\:\:\:\:\:\:−{e}^{−{x}} \left(\mathrm{sin}\:{x}−\mathrm{cos}\:{x}\right.}\end{vmatrix} \\ $$$$=\:−{e}^{−\mathrm{2}{x}} \left(\mathrm{cos}\:{x}\mathrm{sin}\:{x}−\mathrm{cos}\:^{\mathrm{2}} {x}\right)+{e}^{−\mathrm{2}{x}} \left(\mathrm{sin}\:{x}\right. \\ $$$$\left.\mathrm{cos}\:{x}\:+\mathrm{sin}\:^{\mathrm{2}} {x}\right)\:=\:{e}^{−\mathrm{2}{x}} \\ $$$${I}_{\mathrm{1}} =\:{e}^{\mathrm{2}{x}} \int\:{e}^{−{x}} \mathrm{cos}\:{x}.\mathrm{sec}\:{x}\:{dx}\:=\:−{e}^{{x}} \\ $$$${I}_{\mathrm{2}} =\:{e}^{\mathrm{2}{x}} \:\int\:{e}^{−{x}} \mathrm{sin}\:{x}.\mathrm{sec}\:{x}\:{dx}\: \\ $$$${I}_{\mathrm{2}} =\frac{\mathrm{1}}{\mathrm{2}}{e}^{\mathrm{2}{x}} \:\int\:{e}^{−{x}} \mathrm{sin}\:\mathrm{2}{x}\:{dx}\: \\ $$$${y}_{{p}} \:=\:−{y}_{\mathrm{1}} {I}_{\mathrm{2}} +\:{y}_{\mathrm{2}} {I}_{\mathrm{1}} \\ $$
Answered by mathmax by abdo last updated on 19/Jul/20
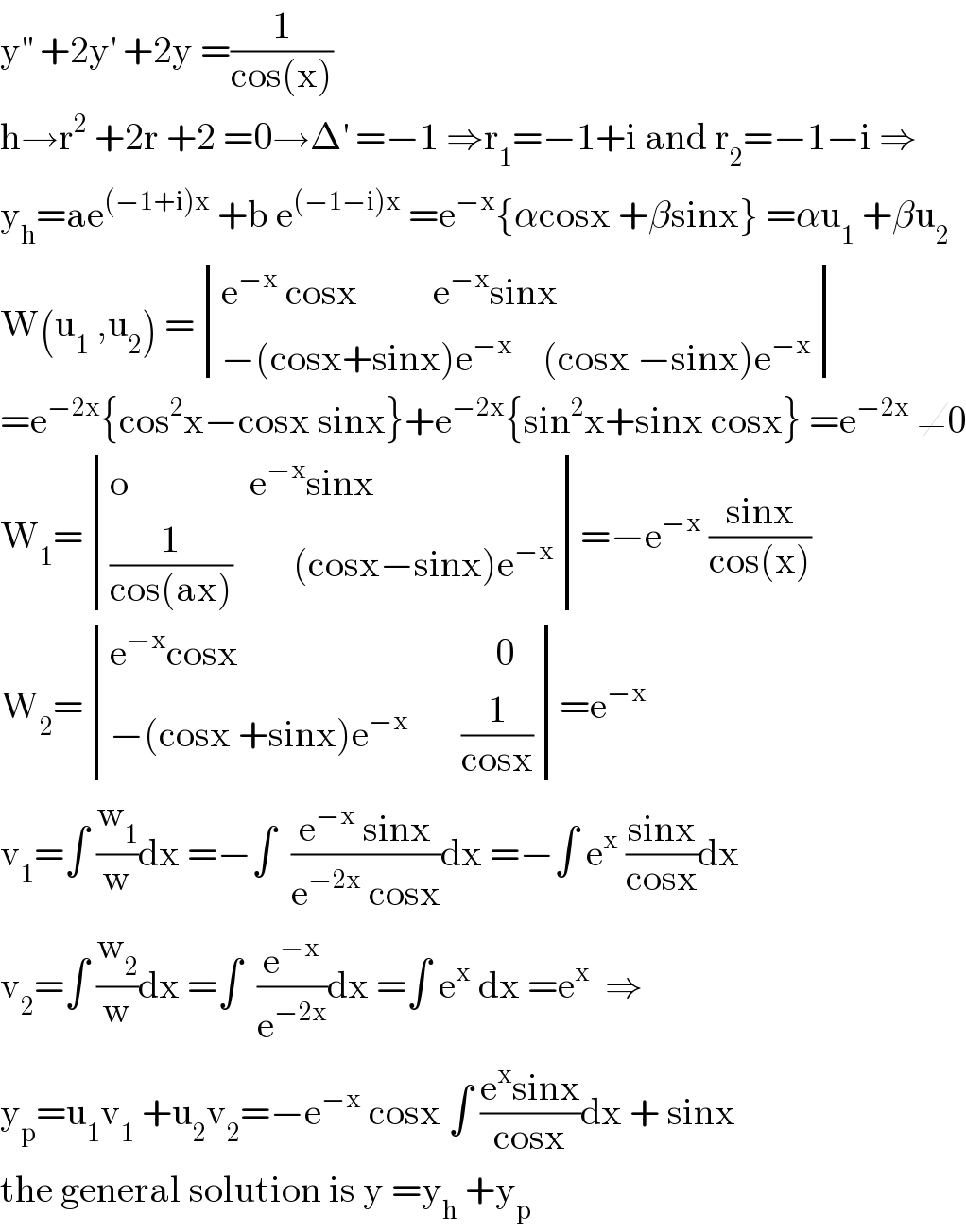
$$\mathrm{y}^{''} \:+\mathrm{2y}^{'} \:+\mathrm{2y}\:=\frac{\mathrm{1}}{\mathrm{cos}\left(\mathrm{x}\right)} \\ $$$$\mathrm{h}\rightarrow\mathrm{r}^{\mathrm{2}} \:+\mathrm{2r}\:+\mathrm{2}\:=\mathrm{0}\rightarrow\Delta^{'} \:=−\mathrm{1}\:\Rightarrow\mathrm{r}_{\mathrm{1}} =−\mathrm{1}+\mathrm{i}\:\mathrm{and}\:\mathrm{r}_{\mathrm{2}} =−\mathrm{1}−\mathrm{i}\:\Rightarrow \\ $$$$\mathrm{y}_{\mathrm{h}} =\mathrm{ae}^{\left(−\mathrm{1}+\mathrm{i}\right)\mathrm{x}} \:+\mathrm{b}\:\mathrm{e}^{\left(−\mathrm{1}−\mathrm{i}\right)\mathrm{x}} \:=\mathrm{e}^{−\mathrm{x}} \left\{\alpha\mathrm{cosx}\:+\beta\mathrm{sinx}\right\}\:=\alpha\mathrm{u}_{\mathrm{1}} \:+\beta\mathrm{u}_{\mathrm{2}} \\ $$$$\mathrm{W}\left(\mathrm{u}_{\mathrm{1}} \:,\mathrm{u}_{\mathrm{2}} \right)\:=\begin{vmatrix}{\mathrm{e}^{−\mathrm{x}} \:\mathrm{cosx}\:\:\:\:\:\:\:\:\:\:\mathrm{e}^{−\mathrm{x}} \mathrm{sinx}}\\{−\left(\mathrm{cosx}+\mathrm{sinx}\right)\mathrm{e}^{−\mathrm{x}} \:\:\:\:\left(\mathrm{cosx}\:−\mathrm{sinx}\right)\mathrm{e}^{−\mathrm{x}} }\end{vmatrix} \\ $$$$=\mathrm{e}^{−\mathrm{2x}} \left\{\mathrm{cos}^{\mathrm{2}} \mathrm{x}−\mathrm{cosx}\:\mathrm{sinx}\right\}+\mathrm{e}^{−\mathrm{2x}} \left\{\mathrm{sin}^{\mathrm{2}} \mathrm{x}+\mathrm{sinx}\:\mathrm{cosx}\right\}\:=\mathrm{e}^{−\mathrm{2x}} \:\neq\mathrm{0} \\ $$$$\mathrm{W}_{\mathrm{1}} =\begin{vmatrix}{\mathrm{o}\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\mathrm{e}^{−\mathrm{x}} \mathrm{sinx}}\\{\frac{\mathrm{1}}{\mathrm{cos}\left(\mathrm{ax}\right)}\:\:\:\:\:\:\:\:\left(\mathrm{cosx}−\mathrm{sinx}\right)\mathrm{e}^{−\mathrm{x}} }\end{vmatrix}=−\mathrm{e}^{−\mathrm{x}} \:\frac{\mathrm{sinx}}{\mathrm{cos}\left(\mathrm{x}\right)} \\ $$$$\mathrm{W}_{\mathrm{2}} =\begin{vmatrix}{\mathrm{e}^{−\mathrm{x}} \mathrm{cosx}\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\mathrm{0}}\\{−\left(\mathrm{cosx}\:+\mathrm{sinx}\right)\mathrm{e}^{−\mathrm{x}} \:\:\:\:\:\:\:\frac{\mathrm{1}}{\mathrm{cosx}}}\end{vmatrix}=\mathrm{e}^{−\mathrm{x}} \: \\ $$$$\mathrm{v}_{\mathrm{1}} =\int\:\frac{\mathrm{w}_{\mathrm{1}} }{\mathrm{w}}\mathrm{dx}\:=−\int\:\:\frac{\mathrm{e}^{−\mathrm{x}} \:\mathrm{sinx}}{\mathrm{e}^{−\mathrm{2x}} \:\mathrm{cosx}}\mathrm{dx}\:=−\int\:\mathrm{e}^{\mathrm{x}} \:\frac{\mathrm{sinx}}{\mathrm{cosx}}\mathrm{dx} \\ $$$$\mathrm{v}_{\mathrm{2}} =\int\:\frac{\mathrm{w}_{\mathrm{2}} }{\mathrm{w}}\mathrm{dx}\:=\int\:\:\frac{\mathrm{e}^{−\mathrm{x}} }{\mathrm{e}^{−\mathrm{2x}} }\mathrm{dx}\:=\int\:\mathrm{e}^{\mathrm{x}} \:\mathrm{dx}\:=\mathrm{e}^{\mathrm{x}} \:\:\Rightarrow \\ $$$$\mathrm{y}_{\mathrm{p}} =\mathrm{u}_{\mathrm{1}} \mathrm{v}_{\mathrm{1}} \:+\mathrm{u}_{\mathrm{2}} \mathrm{v}_{\mathrm{2}} =−\mathrm{e}^{−\mathrm{x}} \:\mathrm{cosx}\:\int\:\frac{\mathrm{e}^{\mathrm{x}} \mathrm{sinx}}{\mathrm{cosx}}\mathrm{dx}\:+\:\mathrm{sinx}\: \\ $$$$\mathrm{the}\:\mathrm{general}\:\mathrm{solution}\:\mathrm{is}\:\mathrm{y}\:=\mathrm{y}_{\mathrm{h}} \:+\mathrm{y}_{\mathrm{p}} \\ $$
