
Previous in Relation and Functions Next in Relation and Functions
Question Number 15262 by Tinkutara last updated on 08/Jun/17
![Solve : x^2 − 6x + [x] + 7 = 0.](Q15262.png)
$$\mathrm{Solve}\::\:{x}^{\mathrm{2}} \:−\:\mathrm{6}{x}\:+\:\left[{x}\right]\:+\:\mathrm{7}\:=\:\mathrm{0}. \\ $$
Commented by ajfour last updated on 08/Jun/17
![(x−3)^2 =2−[x] No solution .](Q15276.png)
$$\left({x}−\mathrm{3}\right)^{\mathrm{2}} =\mathrm{2}−\left[{x}\right] \\ $$$${No}\:{solution}\:. \\ $$
Commented by mrW1 last updated on 08/Jun/17
![what were if x^2 − 6x + [x] + 5 = 0?](Q15283.png)
$$\mathrm{what}\:\mathrm{were}\:\mathrm{if}\:{x}^{\mathrm{2}} \:−\:\mathrm{6}{x}\:+\:\left[{x}\right]\:+\:\mathrm{5}\:=\:\mathrm{0}? \\ $$
Commented by Tinkutara last updated on 09/Jun/17
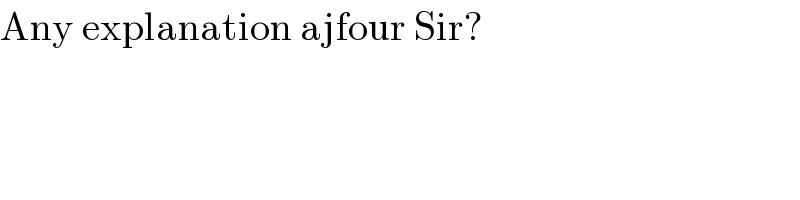
$$\mathrm{Any}\:\mathrm{explanation}\:\mathrm{ajfour}\:\mathrm{Sir}? \\ $$
Commented by ajfour last updated on 09/Jun/17

$${yes}\:{Q}.\mathrm{15288}\:\left({i}\:{tried}\:{to}\:{upload}\right. \\ $$$${image}\:{here},\:{it}\:{got}\:{uploaded}\:{as} \\ $$$$\left.{question}\right). \\ $$
Commented by Tinkutara last updated on 09/Jun/17

$$\mathrm{Thanks}\:\mathrm{Sir}! \\ $$
Commented by mrW1 last updated on 11/Jun/17
![How to solve such equations without using graph? For example x^2 −6x+[x]+5=0](Q15537.png)
$$\mathrm{How}\:\mathrm{to}\:\mathrm{solve}\:\mathrm{such}\:\mathrm{equations}\:\mathrm{without} \\ $$$$\mathrm{using}\:\mathrm{graph}?\:\mathrm{For}\:\mathrm{example} \\ $$$$\mathrm{x}^{\mathrm{2}} −\mathrm{6x}+\left[\mathrm{x}\right]+\mathrm{5}=\mathrm{0} \\ $$
Commented by Tinkutara last updated on 13/Jun/17
![Sir I found a good method. We will write [x] = x − {x} and because we know that 0 ≤ {x} < 1, we will use this range in the quadratic expression so formed. Thus we can solve such type of questions without graph.](Q15717.png)
$$\mathrm{Sir}\:\mathrm{I}\:\mathrm{found}\:\mathrm{a}\:\mathrm{good}\:\mathrm{method}.\:\mathrm{We}\:\mathrm{will} \\ $$$$\mathrm{write}\:\left[{x}\right]\:=\:{x}\:−\:\left\{{x}\right\}\:\mathrm{and}\:\mathrm{because}\:\mathrm{we}\:\mathrm{know} \\ $$$$\mathrm{that}\:\mathrm{0}\:\leqslant\:\left\{{x}\right\}\:<\:\mathrm{1},\:\mathrm{we}\:\mathrm{will}\:\mathrm{use}\:\mathrm{this}\:\mathrm{range} \\ $$$$\mathrm{in}\:\mathrm{the}\:\mathrm{quadratic}\:\mathrm{expression}\:\mathrm{so}\:\mathrm{formed}. \\ $$$$\mathrm{Thus}\:\mathrm{we}\:\mathrm{can}\:\mathrm{solve}\:\mathrm{such}\:\mathrm{type}\:\mathrm{of} \\ $$$$\mathrm{questions}\:\mathrm{without}\:\mathrm{graph}. \\ $$
