Question and Answers Forum

All Questions      Topic List

Algebra Questions

Previous in All Question      Next in All Question      

Previous in Algebra      Next in Algebra      

Question Number 182093 by Fridunatjan08 last updated on 04/Dec/22

Solve the equation: ((x−6)/(2020))+((x−5)/(2021))+((x−4)/(2022))=3

$${Solve}\:{the}\:{equation}: \\ $$$$\frac{{x}−\mathrm{6}}{\mathrm{2020}}+\frac{{x}−\mathrm{5}}{\mathrm{2021}}+\frac{{x}−\mathrm{4}}{\mathrm{2022}}=\mathrm{3} \\ $$

Commented by Fridunatjan08 last updated on 04/Dec/22

Please show me the easy way to solve this equation

$${Please}\:{show}\:{me}\:{the}\:{easy}\:{way}\:{to}\:{solve}\:{this}\:{equation} \\ $$

Commented by CElcedricjunior last updated on 04/Dec/22

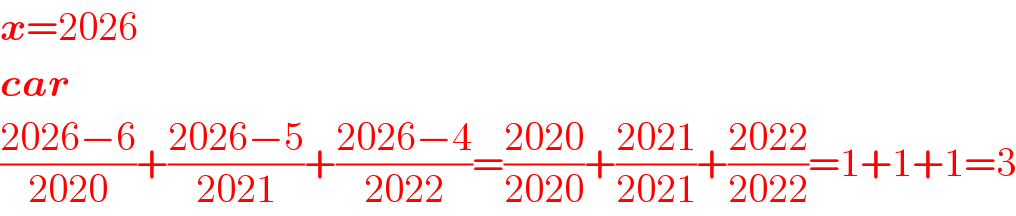
x=2026 car ((2026−6)/(2020))+((2026−5)/(2021))+((2026−4)/(2022))=((2020)/(2020))+((2021)/(2021))+((2022)/(2022))=1+1+1=3

$$\boldsymbol{{x}}=\mathrm{2026} \\ $$$$\boldsymbol{{car}} \\ $$$$\frac{\mathrm{2026}−\mathrm{6}}{\mathrm{2020}}+\frac{\mathrm{2026}−\mathrm{5}}{\mathrm{2021}}+\frac{\mathrm{2026}−\mathrm{4}}{\mathrm{2022}}=\frac{\mathrm{2020}}{\mathrm{2020}}+\frac{\mathrm{2021}}{\mathrm{2021}}+\frac{\mathrm{2022}}{\mathrm{2022}}=\mathrm{1}+\mathrm{1}+\mathrm{1}=\mathrm{3} \\ $$

Answered by Rasheed.Sindhi last updated on 04/Dec/22

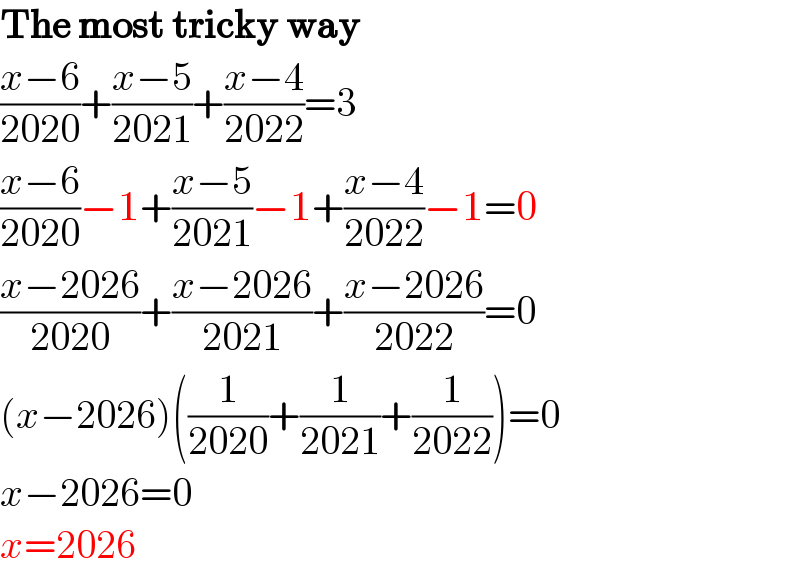
The most tricky way ((x−6)/(2020))+((x−5)/(2021))+((x−4)/(2022))=3 ((x−6)/(2020))−1+((x−5)/(2021))−1+((x−4)/(2022))−1=0 ((x−2026)/(2020))+((x−2026)/(2021))+((x−2026)/(2022))=0 (x−2026)((1/(2020))+(1/(2021))+(1/(2022)))=0 x−2026=0 x=2026

$$\boldsymbol{\mathrm{The}}\:\boldsymbol{\mathrm{most}}\:\boldsymbol{\mathrm{tricky}}\:\boldsymbol{\mathrm{way}} \\ $$$$\frac{{x}−\mathrm{6}}{\mathrm{2020}}+\frac{{x}−\mathrm{5}}{\mathrm{2021}}+\frac{{x}−\mathrm{4}}{\mathrm{2022}}=\mathrm{3} \\ $$$$\frac{{x}−\mathrm{6}}{\mathrm{2020}}−\mathrm{1}+\frac{{x}−\mathrm{5}}{\mathrm{2021}}−\mathrm{1}+\frac{{x}−\mathrm{4}}{\mathrm{2022}}−\mathrm{1}=\mathrm{0} \\ $$$$\frac{{x}−\mathrm{2026}}{\mathrm{2020}}+\frac{{x}−\mathrm{2026}}{\mathrm{2021}}+\frac{{x}−\mathrm{2026}}{\mathrm{2022}}=\mathrm{0} \\ $$$$\left({x}−\mathrm{2026}\right)\left(\frac{\mathrm{1}}{\mathrm{2020}}+\frac{\mathrm{1}}{\mathrm{2021}}+\frac{\mathrm{1}}{\mathrm{2022}}\right)=\mathrm{0} \\ $$$${x}−\mathrm{2026}=\mathrm{0} \\ $$$${x}=\mathrm{2026} \\ $$

Commented by Fridunatjan08 last updated on 04/Dec/22

thanks you!

$${thanks}\:{you}! \\ $$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com