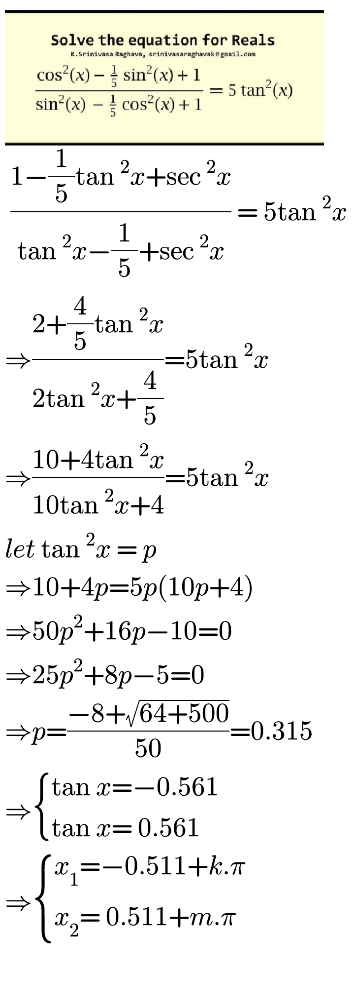Question and Answers Forum

All Questions      Topic List

Trigonometry Questions

Previous in All Question      Next in All Question      

Previous in Trigonometry      Next in Trigonometry      

Question Number 154216 by EDWIN88 last updated on 15/Sep/21

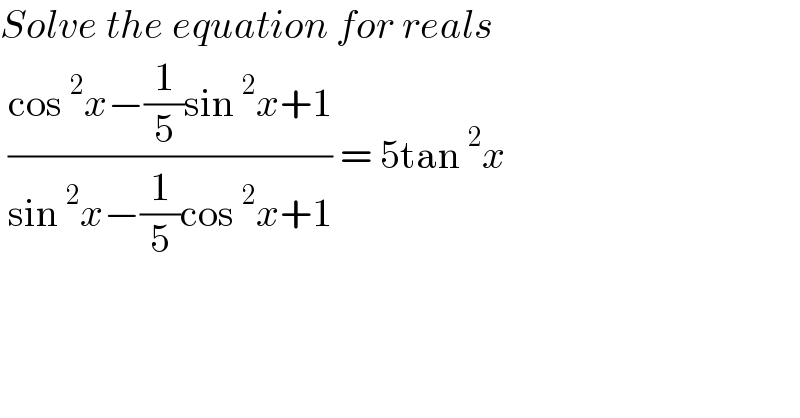
Solve the equation for reals ((cos^2 x−(1/5)sin^2 x+1)/(sin^2 x−(1/5)cos^2 x+1)) = 5tan^2 x

$${Solve}\:{the}\:{equation}\:{for}\:{reals}\: \\ $$$$\:\frac{\mathrm{cos}\:^{\mathrm{2}} {x}−\frac{\mathrm{1}}{\mathrm{5}}\mathrm{sin}\:^{\mathrm{2}} {x}+\mathrm{1}}{\mathrm{sin}\:^{\mathrm{2}} {x}−\frac{\mathrm{1}}{\mathrm{5}}\mathrm{cos}\:^{\mathrm{2}} {x}+\mathrm{1}}\:=\:\mathrm{5tan}\:^{\mathrm{2}} {x}\: \\ $$

Answered by MJS_new last updated on 15/Sep/21

y=cos^2 x ⇒ 0≤y≤1 y^2 −(7/2)y+((25)/(12))=0 ⇒ y=((21−(√(141)))/(12)) x=nπ±arccos (√((21−(√(141)))/(12)))

$${y}=\mathrm{cos}^{\mathrm{2}} \:{x} \\ $$$$\Rightarrow \\ $$$$\mathrm{0}\leqslant{y}\leqslant\mathrm{1} \\ $$$${y}^{\mathrm{2}} −\frac{\mathrm{7}}{\mathrm{2}}{y}+\frac{\mathrm{25}}{\mathrm{12}}=\mathrm{0} \\ $$$$\Rightarrow\:{y}=\frac{\mathrm{21}−\sqrt{\mathrm{141}}}{\mathrm{12}} \\ $$$${x}={n}\pi\pm\mathrm{arccos}\:\sqrt{\frac{\mathrm{21}−\sqrt{\mathrm{141}}}{\mathrm{12}}} \\ $$

Answered by benhamimed last updated on 15/Sep/21

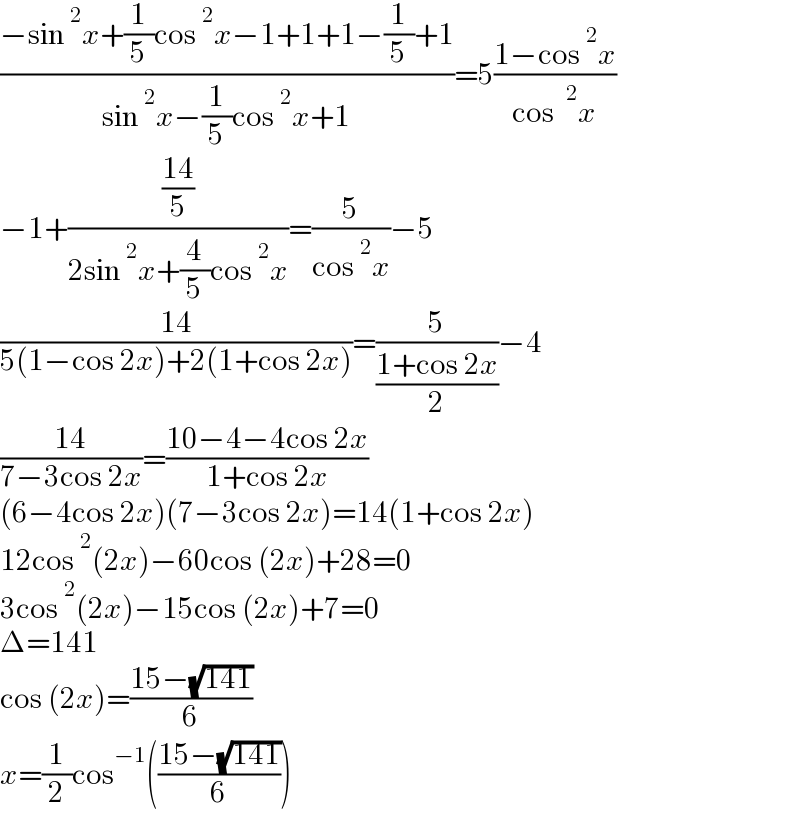
((−sin^2 x+(1/5)cos^2 x−1+1+1−(1/5)+1)/(sin^2 x−(1/5)cos^2 x+1))=5((1−cos^2 x)/(cos ^2 x)) −1+(((14)/5)/(2sin^2 x+(4/5)cos^2 x))=(5/(cos^2 x))−5 ((14)/(5(1−cos 2x)+2(1+cos 2x)))=(5/((1+cos 2x)/2))−4 ((14)/(7−3cos 2x))=((10−4−4cos 2x)/(1+cos 2x)) (6−4cos 2x)(7−3cos 2x)=14(1+cos 2x) 12cos^2 (2x)−60cos (2x)+28=0 3cos^2 (2x)−15cos (2x)+7=0 Δ=141 cos (2x)=((15−(√(141)))/6) x=(1/2)cos^(−1) (((15−(√(141)))/6))

$$\frac{−\mathrm{sin}\:^{\mathrm{2}} {x}+\frac{\mathrm{1}}{\mathrm{5}}\mathrm{cos}\:^{\mathrm{2}} {x}−\mathrm{1}+\mathrm{1}+\mathrm{1}−\frac{\mathrm{1}}{\mathrm{5}}+\mathrm{1}}{\mathrm{sin}\:^{\mathrm{2}} {x}−\frac{\mathrm{1}}{\mathrm{5}}\mathrm{cos}\:^{\mathrm{2}} {x}+\mathrm{1}}=\mathrm{5}\frac{\mathrm{1}−\mathrm{cos}\:^{\mathrm{2}} {x}}{\mathrm{cos}\:\:^{\mathrm{2}} {x}} \\ $$$$−\mathrm{1}+\frac{\frac{\mathrm{14}}{\mathrm{5}}}{\mathrm{2sin}\:^{\mathrm{2}} {x}+\frac{\mathrm{4}}{\mathrm{5}}\mathrm{cos}\:^{\mathrm{2}} {x}}=\frac{\mathrm{5}}{\mathrm{cos}\:^{\mathrm{2}} {x}}−\mathrm{5} \\ $$$$\frac{\mathrm{14}}{\mathrm{5}\left(\mathrm{1}−\mathrm{cos}\:\mathrm{2}{x}\right)+\mathrm{2}\left(\mathrm{1}+\mathrm{cos}\:\mathrm{2}{x}\right)}=\frac{\mathrm{5}}{\frac{\mathrm{1}+\mathrm{cos}\:\mathrm{2}{x}}{\mathrm{2}}}−\mathrm{4} \\ $$$$\frac{\mathrm{14}}{\mathrm{7}−\mathrm{3cos}\:\mathrm{2}{x}}=\frac{\mathrm{10}−\mathrm{4}−\mathrm{4cos}\:\mathrm{2}{x}}{\mathrm{1}+\mathrm{cos}\:\mathrm{2}{x}} \\ $$$$\left(\mathrm{6}−\mathrm{4cos}\:\mathrm{2}{x}\right)\left(\mathrm{7}−\mathrm{3cos}\:\mathrm{2}{x}\right)=\mathrm{14}\left(\mathrm{1}+\mathrm{cos}\:\mathrm{2}{x}\right) \\ $$$$\mathrm{12cos}\:^{\mathrm{2}} \left(\mathrm{2}{x}\right)−\mathrm{60cos}\:\left(\mathrm{2}{x}\right)+\mathrm{28}=\mathrm{0} \\ $$$$\mathrm{3cos}\:^{\mathrm{2}} \left(\mathrm{2}{x}\right)−\mathrm{15cos}\:\left(\mathrm{2}{x}\right)+\mathrm{7}=\mathrm{0} \\ $$$$\Delta=\mathrm{141} \\ $$$$\mathrm{cos}\:\left(\mathrm{2}{x}\right)=\frac{\mathrm{15}−\sqrt{\mathrm{141}}}{\mathrm{6}}\:\: \\ $$$${x}=\frac{\mathrm{1}}{\mathrm{2}}\mathrm{cos}^{−\mathrm{1}} \left(\frac{\mathrm{15}−\sqrt{\mathrm{141}}}{\mathrm{6}}\right) \\ $$

Answered by liberty last updated on 16/Sep/21

Terms of Service

Privacy Policy

Contact: info@tinkutara.com