
Previous in Differential Equation Next in Differential Equation
Question Number 175670 by Engr_Jidda last updated on 04/Sep/22
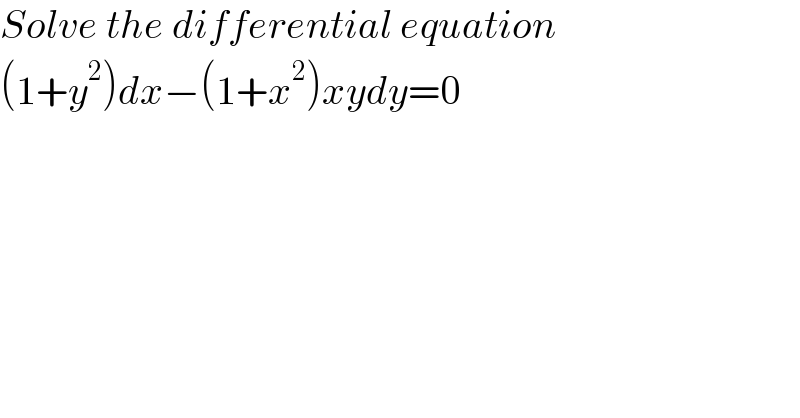
$${Solve}\:{the}\:{differential}\:{equation} \\ $$$$\left(\mathrm{1}+{y}^{\mathrm{2}} \right){dx}−\left(\mathrm{1}+{x}^{\mathrm{2}} \right){xydy}=\mathrm{0} \\ $$
Answered by mahdipoor last updated on 04/Sep/22
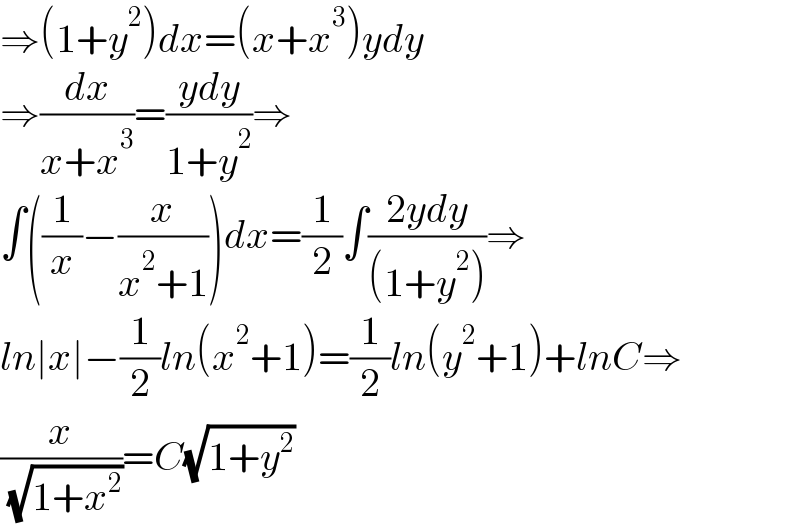
$$\Rightarrow\left(\mathrm{1}+{y}^{\mathrm{2}} \right){dx}=\left({x}+{x}^{\mathrm{3}} \right){ydy} \\ $$$$\Rightarrow\frac{{dx}}{{x}+{x}^{\mathrm{3}} }=\frac{{ydy}}{\mathrm{1}+{y}^{\mathrm{2}} }\Rightarrow \\ $$$$\int\left(\frac{\mathrm{1}}{{x}}−\frac{{x}}{{x}^{\mathrm{2}} +\mathrm{1}}\right){dx}=\frac{\mathrm{1}}{\mathrm{2}}\int\frac{\mathrm{2}{ydy}}{\left(\mathrm{1}+{y}^{\mathrm{2}} \right)}\Rightarrow \\ $$$${ln}\mid{x}\mid−\frac{\mathrm{1}}{\mathrm{2}}{ln}\left({x}^{\mathrm{2}} +\mathrm{1}\right)=\frac{\mathrm{1}}{\mathrm{2}}{ln}\left({y}^{\mathrm{2}} +\mathrm{1}\right)+{lnC}\Rightarrow \\ $$$$\frac{{x}}{\:\sqrt{\mathrm{1}+{x}^{\mathrm{2}} }}={C}\sqrt{\mathrm{1}+{y}^{\mathrm{2}} } \\ $$
Commented by Engr_Jidda last updated on 05/Sep/22

$${tnank}\:{you}\:{boss} \\ $$
Commented by peter frank last updated on 05/Sep/22

$$\mathrm{thanks} \\ $$
Commented by mahdipoor last updated on 05/Sep/22

$$\heartsuit \\ $$
