
Question Number 78168 by TawaTawa last updated on 14/Jan/20
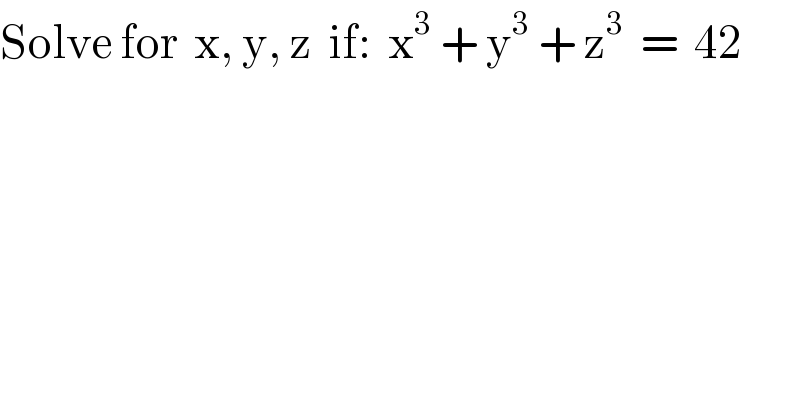
$$\mathrm{Solve}\:\mathrm{for}\:\:\mathrm{x},\:\mathrm{y},\:\mathrm{z}\:\:\mathrm{if}:\:\:\mathrm{x}^{\mathrm{3}} \:+\:\mathrm{y}^{\mathrm{3}} \:+\:\mathrm{z}^{\mathrm{3}} \:\:=\:\:\mathrm{42} \\ $$
Commented by MJS last updated on 15/Jan/20

$${x}\in\mathbb{N},\:\mathbb{Z},\:\mathbb{R}? \\ $$
Commented by TawaTawa last updated on 15/Jan/20

$$\mathbb{R}\:\mathrm{sir} \\ $$
Commented by mr W last updated on 15/Jan/20

$$\mathbb{R}\:? \\ $$$${how}\:{do}\:{you}\:{think}? \\ $$$${x}^{\mathrm{2}} +{y}^{\mathrm{2}} +{z}^{\mathrm{2}} =\mathrm{42} \\ $$$${is}\:{a}\:{sphere}.\:{it}\:{has}\:{infinite}\:{points}. \\ $$$$\Rightarrow{infinite}\:{solutions} \\ $$$$ \\ $$$${if}\:\mathbb{N},\:\Rightarrow{no}\:{solution} \\ $$
Commented by TawaTawa last updated on 15/Jan/20
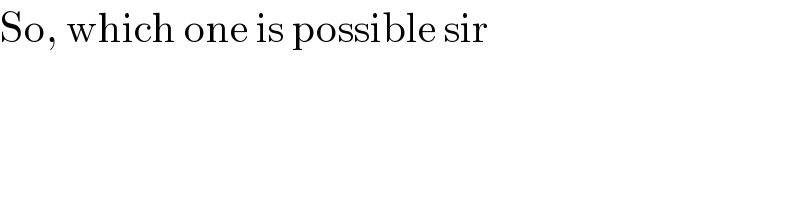
$$\mathrm{So},\:\mathrm{which}\:\mathrm{one}\:\mathrm{is}\:\mathrm{possible}\:\mathrm{sir} \\ $$
Commented by mr W last updated on 15/Jan/20

$${you}\:{can}\:{ask}\:{every}\:{question}.\:{but}\:{this} \\ $$$${question}\:{i}\:{think}\:{is}\:{not}\:{a}\:{good}\:{one}, \\ $$$${both}\:{as}\:\in\mathbb{N}\:{or}\:{as}\:\in\mathbb{R}. \\ $$
Commented by TawaTawa last updated on 15/Jan/20
$$\mathrm{Alright}\:\mathrm{sir}.\:\mathrm{i}\:\mathrm{apprecciate}. \\ $$
