Question and Answers Forum

All Questions      Topic List

Algebra Questions

Previous in All Question      Next in All Question      

Previous in Algebra      Next in Algebra      

Question Number 171549 by Shrinava last updated on 17/Jun/22

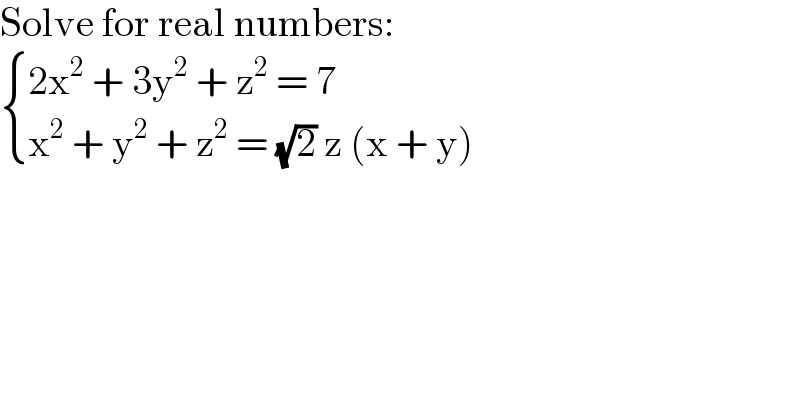
Solve for real numbers: { ((2x^2 + 3y^2 + z^2 = 7)),((x^2 + y^2 + z^2 = (√2) z (x + y))) :}

$$\mathrm{Solve}\:\mathrm{for}\:\mathrm{real}\:\mathrm{numbers}: \\ $$$$\begin{cases}{\mathrm{2x}^{\mathrm{2}} \:+\:\mathrm{3y}^{\mathrm{2}} \:+\:\mathrm{z}^{\mathrm{2}} \:=\:\mathrm{7}}\\{\mathrm{x}^{\mathrm{2}} \:+\:\mathrm{y}^{\mathrm{2}} \:+\:\mathrm{z}^{\mathrm{2}} \:=\:\sqrt{\mathrm{2}}\:\mathrm{z}\:\left(\mathrm{x}\:+\:\mathrm{y}\right)}\end{cases} \\ $$

Answered by MJS_new last updated on 21/Jun/22

let y=px∧z=qx { (((3p^2 +q^2 +2)x^2 −7=0)),(((p^2 −(√2)pq+q^2 −(√2)q+1)x^2 =0)) :} ⇒ x=0∨(p^2 −(√2)pq+q^2 −(√2)q+1)=0 if x=0 the first equation is wrong and p^2 −(√2)pq+q^2 −(√2)q+1=0 ⇒ q=((√2)/2)(p+1±(p−1)i) ⇒ only real if p=1 ⇒ q=(√2) ⇒ (3+2+2)x^2 −7=0 ⇒ x=±1 ⇒ x=±1∧y=x∧z=(√2)x

$$\mathrm{let}\:{y}={px}\wedge{z}={qx} \\ $$$$\begin{cases}{\left(\mathrm{3}{p}^{\mathrm{2}} +{q}^{\mathrm{2}} +\mathrm{2}\right){x}^{\mathrm{2}} −\mathrm{7}=\mathrm{0}}\\{\left({p}^{\mathrm{2}} −\sqrt{\mathrm{2}}{pq}+{q}^{\mathrm{2}} −\sqrt{\mathrm{2}}{q}+\mathrm{1}\right){x}^{\mathrm{2}} =\mathrm{0}}\end{cases} \\ $$$$\Rightarrow\:{x}=\mathrm{0}\vee\left({p}^{\mathrm{2}} −\sqrt{\mathrm{2}}{pq}+{q}^{\mathrm{2}} −\sqrt{\mathrm{2}}{q}+\mathrm{1}\right)=\mathrm{0} \\ $$$$\mathrm{if}\:{x}=\mathrm{0}\:\mathrm{the}\:\mathrm{first}\:\mathrm{equation}\:\mathrm{is}\:\mathrm{wrong}\:\mathrm{and} \\ $$$${p}^{\mathrm{2}} −\sqrt{\mathrm{2}}{pq}+{q}^{\mathrm{2}} −\sqrt{\mathrm{2}}{q}+\mathrm{1}=\mathrm{0}\:\Rightarrow\:{q}=\frac{\sqrt{\mathrm{2}}}{\mathrm{2}}\left({p}+\mathrm{1}\pm\left({p}−\mathrm{1}\right)\mathrm{i}\right) \\ $$$$\Rightarrow\:\mathrm{only}\:\mathrm{real}\:\mathrm{if}\:{p}=\mathrm{1}\:\Rightarrow\:{q}=\sqrt{\mathrm{2}} \\ $$$$\Rightarrow\:\left(\mathrm{3}+\mathrm{2}+\mathrm{2}\right){x}^{\mathrm{2}} −\mathrm{7}=\mathrm{0}\:\Rightarrow\:{x}=\pm\mathrm{1} \\ $$$$\Rightarrow\:{x}=\pm\mathrm{1}\wedge{y}={x}\wedge{z}=\sqrt{\mathrm{2}}{x} \\ $$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com