
Question Number 155204 by mathdanisur last updated on 26/Sep/21
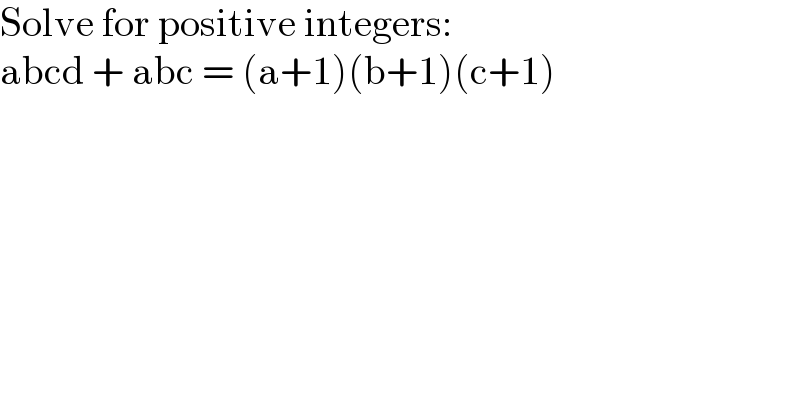
$$\mathrm{Solve}\:\mathrm{for}\:\mathrm{positive}\:\mathrm{integers}: \\ $$$$\mathrm{abcd}\:+\:\mathrm{abc}\:=\:\left(\mathrm{a}+\mathrm{1}\right)\left(\mathrm{b}+\mathrm{1}\right)\left(\mathrm{c}+\mathrm{1}\right) \\ $$
Answered by MJS_new last updated on 27/Sep/21

$${a}\leqslant{b}\leqslant{c} \\ $$$$\mathrm{all}\:\mathrm{possible}\:\mathrm{solutions}\:\mathrm{for}\:{a}\:{b}\:{c}\:{d}\:\mathrm{are} \\ $$$$\mathrm{1}\:\mathrm{1}\:\mathrm{1}\:\mathrm{7} \\ $$$$\mathrm{1}\:\mathrm{1}\:\mathrm{2}\:\mathrm{5} \\ $$$$\mathrm{1}\:\mathrm{1}\:\mathrm{4}\:\mathrm{4} \\ $$$$\mathrm{1}\:\mathrm{2}\:\mathrm{3}\:\mathrm{3} \\ $$$$\mathrm{1}\:\mathrm{3}\:\mathrm{8}\:\mathrm{2} \\ $$$$\mathrm{1}\:\mathrm{4}\:\mathrm{5}\:\mathrm{2} \\ $$$$\mathrm{2}\:\mathrm{2}\:\mathrm{3}\:\mathrm{2} \\ $$$$\mathrm{2}\:\mathrm{4}\:\mathrm{15}\:\mathrm{1} \\ $$$$\mathrm{2}\:\mathrm{5}\:\mathrm{9}\:\mathrm{1} \\ $$$$\mathrm{2}\:\mathrm{6}\:\mathrm{7}\:\mathrm{1} \\ $$$$\mathrm{3}\:\mathrm{3}\:\mathrm{8}\:\mathrm{1} \\ $$$$\mathrm{3}\:\mathrm{4}\:\mathrm{5}\:\mathrm{1} \\ $$
Commented by ajfour last updated on 27/Sep/21
$${sir},\:{i}\:{had}\:{emailed}\:{u}\:{couple}\:{of} \\ $$$${weeks}\:{back};\:{u}\:{dint}\:{reply}! \\ $$
Commented by MJS_new last updated on 27/Sep/21
Sorry I received no email from you, but this happened before, might be an issue of my provider.
Commented by ajfour last updated on 30/Sep/21

$${dint}\:{u}\:{chase}\:{our}\:{prize}\:{further} \\ $$$${sir}?\:\left({more}\:{or}\:{less}\:{i}\:{was}\:{asking}\right. \\ $$$$\left.{this}\right)\:\:\:{at}\:{least}\:{u}\:{can}\:{reply}\:{here} \\ $$$${Sir}\:{MjS}. \\ $$
Commented by mathdanisur last updated on 27/Sep/21

$$\mathrm{Yes}\:\boldsymbol{\mathrm{S}}\mathrm{er},\:\mathrm{thank}\:\mathrm{you},\:\mathrm{solution}\:\mathrm{if}\:\mathrm{possible} \\ $$
Answered by Rasheed.Sindhi last updated on 28/Sep/21

$$\mathrm{Solve}\:\mathrm{for}\:\mathrm{positive}\:\mathrm{integers}: \\ $$$$\underset{−} {\mathrm{abcd}\:+\:\mathrm{abc}\:=\:\left(\mathrm{a}+\mathrm{1}\right)\left(\mathrm{b}+\mathrm{1}\right)\left(\mathrm{c}+\mathrm{1}\right)\:\:\:\:\:\:\:\:\left[}\right. \\ $$$$\mathrm{abc}\left(\mathrm{d}+\mathrm{1}\right)=\left(\mathrm{a}+\mathrm{1}\right)\left(\mathrm{b}+\mathrm{1}\right)\left(\mathrm{c}+\mathrm{1}\right) \\ $$$$\Rightarrow\mathrm{abc}\:\mid\:\left(\mathrm{a}+\mathrm{1}\right)\left(\mathrm{b}+\mathrm{1}\right)\left(\mathrm{c}+\mathrm{1}\right)..........\mathrm{A} \\ $$$$\Rightarrow\begin{cases}{\mathrm{a}\mid\left(\mathrm{a}+\mathrm{1}\right)\Rightarrow\mathrm{a}=\mathrm{1}.........\left(\mathrm{i}\right)}\\{\mathrm{a}\mid\left(\mathrm{b}+\mathrm{1}\right)}\\{\mathrm{a}\mid\left(\mathrm{c}+\mathrm{1}\right)}\\{\mathrm{a}\mid\left(\mathrm{a}+\mathrm{1}\right)\left(\mathrm{b}+\mathrm{1}\right)}\\{\mathrm{a}\mid\left(\mathrm{b}+\mathrm{1}\right)\left(\mathrm{c}+\mathrm{1}\right)}\\{\mathrm{a}\mid\left(\mathrm{c}+\mathrm{1}\right)\left(\mathrm{a}+\mathrm{1}\right)}\\{\mathrm{a}\mid\left(\mathrm{a}+\mathrm{1}\right)\left(\mathrm{b}+\mathrm{1}\right)\left(\mathrm{c}+\mathrm{1}\right)}\end{cases} \\ $$$$\mathrm{a}=\mathrm{1}:\Rightarrow\mathrm{bc}\:\mid\:\mathrm{2}\left(\mathrm{b}+\mathrm{1}\right)\left(\mathrm{c}+\mathrm{1}\right)..........\mathrm{B} \\ $$$$\Rightarrow\begin{cases}{\mathrm{b}\mid\mathrm{2}\Rightarrow\mathrm{b}=\mathrm{1},\mathrm{2}}\\{\mathrm{b}\mid\left(\mathrm{b}+\mathrm{1}\right)\Rightarrow\mathrm{b}=\mathrm{1}}\\{\mathrm{b}\mid\left(\mathrm{c}+\mathrm{1}\right)}\\{\mathrm{b}\mid\mathrm{2}\left(\mathrm{b}+\mathrm{1}\right)}\\{\mathrm{b}\mid\mathrm{2}\left(\mathrm{c}+\mathrm{1}\right)}\\{\mathrm{b}\mid\left(\mathrm{b}+\mathrm{1}\right)\left(\mathrm{c}+\mathrm{1}\right)}\\{\mathrm{b}\mid\mathrm{2}\left(\mathrm{b}+\mathrm{1}\right)\left(\mathrm{c}+\mathrm{1}\right)}\end{cases} \\ $$$$\mathrm{a}=\mathrm{1},\mathrm{b}=\mathrm{1}:\:\mathrm{B}\Rightarrow\mathrm{c}\:\mid\:\mathrm{4}\left(\mathrm{c}+\mathrm{1}\right) \\ $$$$\Rightarrow\begin{cases}{\mathrm{c}\mid\mathrm{4}\Rightarrow\mathrm{c}=\mathrm{1},\mathrm{2},\mathrm{4}}\\{\mathrm{c}\mid\left(\mathrm{c}+\mathrm{1}\right)\Rightarrow\mathrm{c}=\mathrm{1}}\\{\mathrm{c}\mid\mathrm{4}\left(\mathrm{c}+\mathrm{1}\right)}\end{cases} \\ $$$$\mathrm{a}=\mathrm{1},\mathrm{b}=\mathrm{1},\mathrm{c}=\mathrm{1}: \\ $$$$\mathrm{d}+\mathrm{1}=\frac{\left(\mathrm{a}+\mathrm{1}\right)\left(\mathrm{b}+\mathrm{1}\right)\left(\mathrm{c}+\mathrm{1}\right)}{\mathrm{abc}} \\ $$$$\:\:\:\:\Rightarrow\mathrm{d}+\mathrm{1}=\frac{\mathrm{2}.\mathrm{2}.\mathrm{2}}{\mathrm{1}.\mathrm{1}.\mathrm{1}}\Rightarrow\mathrm{d}=\mathrm{7} \\ $$$$\mathrm{a}=\mathrm{1},\mathrm{b}=\mathrm{2},\mathrm{c}=\mathrm{1}: \\ $$$$\:\:\:\mathrm{d}+\mathrm{1}=\frac{\mathrm{2}.\mathrm{3}.\mathrm{2}}{\mathrm{1}.\mathrm{2}.\mathrm{1}}=\mathrm{6}\Rightarrow\mathrm{d}=\mathrm{5} \\ $$$$\mathrm{a}=\mathrm{1},\mathrm{b}=\mathrm{1},\mathrm{c}=\mathrm{2}: \\ $$$$\:\:\:\:\:\:\Rightarrow\mathrm{d}+\mathrm{1}=\frac{\mathrm{2}.\mathrm{2}.\mathrm{3}}{\mathrm{1}.\mathrm{1}.\mathrm{2}}=\mathrm{6}\Rightarrow\mathrm{d}=\mathrm{5} \\ $$$$ \\ $$$$\mathrm{Continue}... \\ $$
Answered by Rasheed.Sindhi last updated on 29/Sep/21

$$\mathrm{Solve}\:\mathrm{for}\:\mathrm{positive}\:\mathrm{integers}: \\ $$$$\mathrm{abcd}\:+\:\mathrm{abc}\:=\:\left(\mathrm{a}+\mathrm{1}\right)\left(\mathrm{b}+\mathrm{1}\right)\left(\mathrm{c}+\mathrm{1}\right) \\ $$$$\mathrm{d}+\mathrm{1}=\frac{\left(\mathrm{a}+\mathrm{1}\right)\left(\mathrm{b}+\mathrm{1}\right)\left(\mathrm{c}+\mathrm{1}\right)}{\mathrm{abc}}...........\mathrm{A} \\ $$$$\mathrm{since}\:\mathrm{d}\in\mathbb{Z}^{+} \\ $$$$\therefore\:\:\frac{\left(\mathrm{a}+\mathrm{1}\right)\left(\mathrm{b}+\mathrm{1}\right)\left(\mathrm{c}+\mathrm{1}\right)}{\mathrm{abc}}\in\mathbb{Z}^{+} \\ $$$$\mathrm{One}\:\mathrm{of}\:\mathrm{many}\:\mathrm{possibilities}\:\mathrm{is}\:\mathrm{a}\mid\left(\mathrm{a}+\mathrm{1}\right) \\ $$$$\mathrm{and}\:\mathrm{we}\:\mathrm{start}\:\mathrm{from}\:\mathrm{it} \\ $$$$\mathrm{a}\mid\left(\mathrm{a}+\mathrm{1}\right)\Rightarrow\mathrm{a}=\mathrm{1} \\ $$$$\mathrm{Let}\:\:\mathrm{a}\leqslant\mathrm{b}\leqslant\mathrm{c} \\ $$$$\mathrm{a}=\mathrm{1}:\mathrm{A}\Rightarrow\mathrm{d}+\mathrm{1}=\frac{\left(\mathrm{1}+\mathrm{1}\right)\left(\mathrm{b}+\mathrm{1}\right)\left(\mathrm{c}+\mathrm{1}\right)}{\mathrm{1}.\mathrm{bc}} \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:=\frac{\mathrm{2}\left(\mathrm{b}+\mathrm{1}\right)\left(\mathrm{c}+\mathrm{1}\right)}{\mathrm{bc}}\Rightarrow\mathrm{b}=\mathrm{1},\mathrm{2},... \\ $$$$\:\:\:\:\:\:\:\:\:\:\mathrm{b}=\mathrm{1}:\:\mathrm{d}+\mathrm{1}=\frac{\mathrm{2}\left(\mathrm{1}+\mathrm{1}\right)\left(\mathrm{c}+\mathrm{1}\right)}{\mathrm{1}.\mathrm{c}} \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:=\frac{\mathrm{4}\left(\mathrm{c}+\mathrm{1}\right)}{\mathrm{c}}\Rightarrow\mathrm{c}=\mathrm{1},\mathrm{2},\mathrm{4} \\ $$$$\mathrm{d}\left(\mathrm{a},\mathrm{b},\mathrm{c}\right)=\frac{\left(\mathrm{a}+\mathrm{1}\right)\left(\mathrm{b}+\mathrm{1}\right)\left(\mathrm{c}+\mathrm{1}\right)}{\mathrm{abc}}−\mathrm{1} \\ $$$$\mathrm{d}\left(\mathrm{1},\mathrm{1},\mathrm{1}\right)=\frac{\left(\mathrm{1}+\mathrm{1}\right)\left(\mathrm{1}+\mathrm{1}\right)\left(\mathrm{1}+\mathrm{1}\right)}{\mathrm{1}.\mathrm{1}.\mathrm{1}}−\mathrm{1}=\mathrm{7} \\ $$$$\mathrm{d}\left(\mathrm{1},\mathrm{1},\mathrm{2}\right)=\frac{\left(\mathrm{1}+\mathrm{1}\right)\left(\mathrm{1}+\mathrm{1}\right)\left(\mathrm{2}+\mathrm{1}\right)}{\mathrm{1}.\mathrm{1}.\mathrm{2}}−\mathrm{1}=\mathrm{5} \\ $$$$\mathrm{d}\left(\mathrm{1},\mathrm{1},\mathrm{4}\right)=\frac{\left(\mathrm{1}+\mathrm{1}\right)\left(\mathrm{1}+\mathrm{1}\right)\left(\mathrm{4}+\mathrm{1}\right)}{\mathrm{1}.\mathrm{1}.\mathrm{4}}−\mathrm{1}=\mathrm{4} \\ $$$$\mathrm{a}=\mathrm{1}:\:\:\mathrm{d}+\mathrm{1}=\frac{\left(\mathrm{1}+\mathrm{1}\right)\left(\mathrm{b}+\mathrm{1}\right)\left(\mathrm{c}+\mathrm{1}\right)}{\mathrm{1}.\mathrm{b}.\mathrm{c}} \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:=\frac{\mathrm{2}\left(\mathrm{b}+\mathrm{1}\right)\left(\mathrm{c}+\mathrm{1}\right)}{\mathrm{b}.\mathrm{c}}\Rightarrow\mathrm{b}=\mathrm{1},\mathrm{2} \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\mathrm{b}=\mathrm{2}: \\ $$$$\:\:\:\:\:\:\:\:\:\mathrm{d}+\mathrm{1}=\frac{\mathrm{2}\left(\mathrm{2}+\mathrm{1}\right)\left(\mathrm{c}+\mathrm{1}\right)}{\mathrm{1}.\mathrm{2}.\mathrm{c}}=\frac{\mathrm{3}\left(\mathrm{c}+\mathrm{1}\right)}{\mathrm{c}}\Rightarrow\mathrm{c}=\mathrm{1},\mathrm{3} \\ $$$$\mathrm{d}\left(\mathrm{1},\mathrm{2},\mathrm{3}\right)=\frac{\left(\mathrm{1}+\mathrm{1}\right)\left(\mathrm{2}+\mathrm{1}\right)\left(\mathrm{3}+\mathrm{1}\right)}{\mathrm{1}.\mathrm{2}.\mathrm{3}}−\mathrm{1}=\mathrm{3} \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\: \\ $$$$\mathrm{Continue}... \\ $$
