
Question Number 181331 by Mastermind last updated on 24/Nov/22
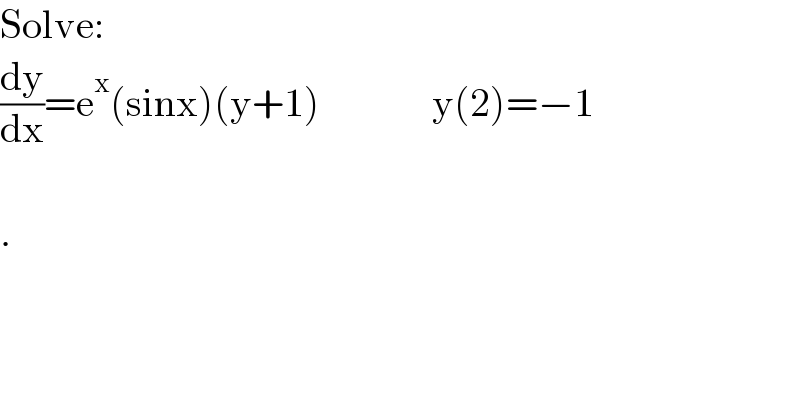
$$\mathrm{Solve}: \\ $$$$\frac{\mathrm{dy}}{\mathrm{dx}}=\mathrm{e}^{\mathrm{x}} \left(\mathrm{sinx}\right)\left(\mathrm{y}+\mathrm{1}\right)\:\:\:\:\:\:\:\:\:\:\:\:\:\:\mathrm{y}\left(\mathrm{2}\right)=−\mathrm{1} \\ $$$$ \\ $$$$. \\ $$
Answered by FelipeLz last updated on 24/Nov/22
![(dy/dx) = e^x sin(x)(y+1) (1/(y+1))dy = e^x sin(x)dx ∫(1/(y+1))dy = ∫e^x sin(x)dx ln∣y+1∣+c_1 = (1/2)e^x [sin(x)−cos(x)]+c_2 ln∣y+1∣ = (1/2)e^x [sin(x)−cos(x)]+c_2 −c_1 c_2 −c_1 = c_3 → ln∣y+1∣ = (1/2)e^x [sin(x)−cos(x)]+c_3 y+1 = e^((1/2)e^x [sin(x)−cos(x)]+c_3 ) e^c_3 = C → y+1 = Ce^((1/2)e^x [sin(x)−cos(x)]) y(x) = Ce^((1/2)e^x [sin(x)−cos(x)]) −1 y(2) = −1 → Ce^((1/2)e^2 [sin(2)−cos(2)]) −1 = −1 Ce^((1/2)e^2 [sin(2)−cos(2)]) −1 = −1 Ce^((1/2)e^2 [sin(2)−cos(2)]) = 0 e^t ≠ 0 ∀t → C = 0 y(x) = −1](Q181354.png)
$$\frac{{dy}}{{dx}}\:=\:{e}^{{x}} \mathrm{sin}\left({x}\right)\left({y}+\mathrm{1}\right) \\ $$$$\frac{\mathrm{1}}{{y}+\mathrm{1}}{dy}\:=\:{e}^{{x}} \mathrm{sin}\left({x}\right){dx} \\ $$$$\int\frac{\mathrm{1}}{{y}+\mathrm{1}}{dy}\:=\:\int{e}^{{x}} \mathrm{sin}\left({x}\right){dx} \\ $$$$\mathrm{ln}\mid{y}+\mathrm{1}\mid+{c}_{\mathrm{1}} \:=\:\frac{\mathrm{1}}{\mathrm{2}}{e}^{{x}} \left[\mathrm{sin}\left({x}\right)−\mathrm{cos}\left({x}\right)\right]+{c}_{\mathrm{2}} \\ $$$$\mathrm{ln}\mid{y}+\mathrm{1}\mid\:=\:\frac{\mathrm{1}}{\mathrm{2}}{e}^{{x}} \left[\mathrm{sin}\left({x}\right)−\mathrm{cos}\left({x}\right)\right]+{c}_{\mathrm{2}} −{c}_{\mathrm{1}} \\ $$$${c}_{\mathrm{2}} −{c}_{\mathrm{1}} \:=\:{c}_{\mathrm{3}} \:\rightarrow\:\mathrm{ln}\mid{y}+\mathrm{1}\mid\:=\:\frac{\mathrm{1}}{\mathrm{2}}{e}^{{x}} \left[\mathrm{sin}\left({x}\right)−\mathrm{cos}\left({x}\right)\right]+{c}_{\mathrm{3}} \\ $$$${y}+\mathrm{1}\:=\:{e}^{\frac{\mathrm{1}}{\mathrm{2}}{e}^{{x}} \left[\mathrm{sin}\left({x}\right)−\mathrm{cos}\left({x}\right)\right]+{c}_{\mathrm{3}} } \\ $$$${e}^{{c}_{\mathrm{3}} } \:=\:{C}\:\rightarrow\:{y}+\mathrm{1}\:=\:{Ce}^{\frac{\mathrm{1}}{\mathrm{2}}{e}^{{x}} \left[\mathrm{sin}\left({x}\right)−\mathrm{cos}\left({x}\right)\right]} \\ $$$${y}\left({x}\right)\:=\:{Ce}^{\frac{\mathrm{1}}{\mathrm{2}}{e}^{{x}} \left[\mathrm{sin}\left({x}\right)−\mathrm{cos}\left({x}\right)\right]} −\mathrm{1} \\ $$$${y}\left(\mathrm{2}\right)\:=\:−\mathrm{1}\:\rightarrow\:{Ce}^{\frac{\mathrm{1}}{\mathrm{2}}{e}^{\mathrm{2}} \left[\mathrm{sin}\left(\mathrm{2}\right)−\mathrm{cos}\left(\mathrm{2}\right)\right]} −\mathrm{1}\:=\:−\mathrm{1}\: \\ $$$${Ce}^{\frac{\mathrm{1}}{\mathrm{2}}{e}^{\mathrm{2}} \left[\mathrm{sin}\left(\mathrm{2}\right)−\mathrm{cos}\left(\mathrm{2}\right)\right]} −\mathrm{1}\:=\:−\mathrm{1} \\ $$$${Ce}^{\frac{\mathrm{1}}{\mathrm{2}}{e}^{\mathrm{2}} \left[\mathrm{sin}\left(\mathrm{2}\right)−\mathrm{cos}\left(\mathrm{2}\right)\right]} \:=\:\mathrm{0} \\ $$$${e}^{{t}} \:\neq\:\mathrm{0}\:\forall{t}\:\rightarrow\:{C}\:=\:\mathrm{0} \\ $$$${y}\left({x}\right)\:=\:−\mathrm{1} \\ $$
