
Previous in Probability and Statistics Next in Probability and Statistics
Question Number 131631 by liberty last updated on 07/Feb/21

$$\mathrm{Selecting}\:\mathrm{randomly}\:\mathrm{an}\:\mathrm{integer} \\ $$$$\mathrm{from}\:\mathrm{1}\:\mathrm{to}\:\mathrm{2000}\:,\:\mathrm{find}\:\mathrm{the}\:\mathrm{probability} \\ $$$$\mathrm{that}\:\mathrm{neither}\:\mathrm{6}\:\mathrm{nor}\:\mathrm{8}\:\mathrm{divides}\:\mathrm{the} \\ $$$$\mathrm{integer}. \\ $$
Answered by som(math1967) last updated on 07/Feb/21
![probability divisible by 6 ((333)/(2000)) [In the sereies 6,12....1998 1998=6+(n−1)×6 ∴n=333] probability divisible by 8 =((250)/(2000)) [8+(n−1)×8=2000 ∴n=250] probability divisible by both 6,8=((83)/(2000)) [1992=24+(n−1)×24 n=83] ∴probability divisible by 6 or 8=((333)/(2000)) +((250)/(2000))−((83)/(2000)) =((500)/(2000)) ∴neither 6 nor 8 1−((500)/(2000))=((1500)/(2000))=(3/4)](Q131633.png)
$${probability}\:{divisible}\:{by}\:\mathrm{6} \\ $$$$\frac{\mathrm{333}}{\mathrm{2000}} \\ $$$$\left[{In}\:{the}\:{sereies}\:\mathrm{6},\mathrm{12}....\mathrm{1998}\right. \\ $$$$\left.\mathrm{1998}=\mathrm{6}+\left({n}−\mathrm{1}\right)×\mathrm{6}\:\therefore{n}=\mathrm{333}\right] \\ $$$${probability}\:{divisible}\:{by}\:\mathrm{8} \\ $$$$=\frac{\mathrm{250}}{\mathrm{2000}}\:\:\left[\mathrm{8}+\left({n}−\mathrm{1}\right)×\mathrm{8}=\mathrm{2000}\right. \\ $$$$\left.\therefore{n}=\mathrm{250}\right] \\ $$$${probability}\:{divisible}\:{by}\:{both} \\ $$$$\mathrm{6},\mathrm{8}=\frac{\mathrm{83}}{\mathrm{2000}} \\ $$$$\left[\mathrm{1992}=\mathrm{24}+\left({n}−\mathrm{1}\right)×\mathrm{24}\right. \\ $$$$\left.{n}=\mathrm{83}\right] \\ $$$$\therefore{probability}\:{divisible}\:{by} \\ $$$$\mathrm{6}\:{or}\:\mathrm{8}=\frac{\mathrm{333}}{\mathrm{2000}}\:+\frac{\mathrm{250}}{\mathrm{2000}}−\frac{\mathrm{83}}{\mathrm{2000}} \\ $$$$=\frac{\mathrm{500}}{\mathrm{2000}} \\ $$$$\therefore{neither}\:\mathrm{6}\:{nor}\:\mathrm{8} \\ $$$$\:\mathrm{1}−\frac{\mathrm{500}}{\mathrm{2000}}=\frac{\mathrm{1500}}{\mathrm{2000}}=\frac{\mathrm{3}}{\mathrm{4}} \\ $$
Commented by liberty last updated on 07/Feb/21
![typo sir it should be ((250)/(2000))[8n] =2000 n=250](Q131634.png)
$$\mathrm{typo}\:\mathrm{sir}\:\mathrm{it}\:\mathrm{should}\:\mathrm{be}\:\frac{\mathrm{250}}{\mathrm{2000}}\left[\mathrm{8n}\right]\:=\mathrm{2000} \\ $$$$\mathrm{n}=\mathrm{250} \\ $$
Commented by som(math1967) last updated on 07/Feb/21
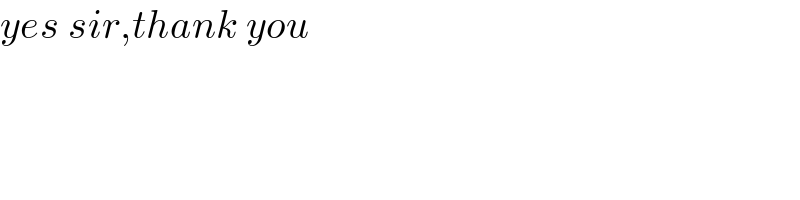
$${yes}\:{sir},{thank}\:{you} \\ $$
