
Question Number 13097 by FilupS last updated on 14/May/17

$${S}=\underset{{x}_{\mathrm{2}} =\mathrm{1}} {\overset{{x}_{\mathrm{1}} } {\sum}}\underset{{x}_{\mathrm{3}} =\mathrm{1}} {\overset{{x}_{\mathrm{2}} } {\sum}}\centerdot\centerdot\centerdot\underset{{x}_{{n}} =\mathrm{1}} {\overset{{x}_{{n}−\mathrm{1}} } {\sum}}\underset{{t}=\mathrm{1}} {\overset{{x}_{{n}} } {\sum}}{t} \\ $$$$\mathrm{Can}\:\mathrm{you}\:\mathrm{evaluate}\:{S}? \\ $$
Answered by nume1114 last updated on 15/May/17
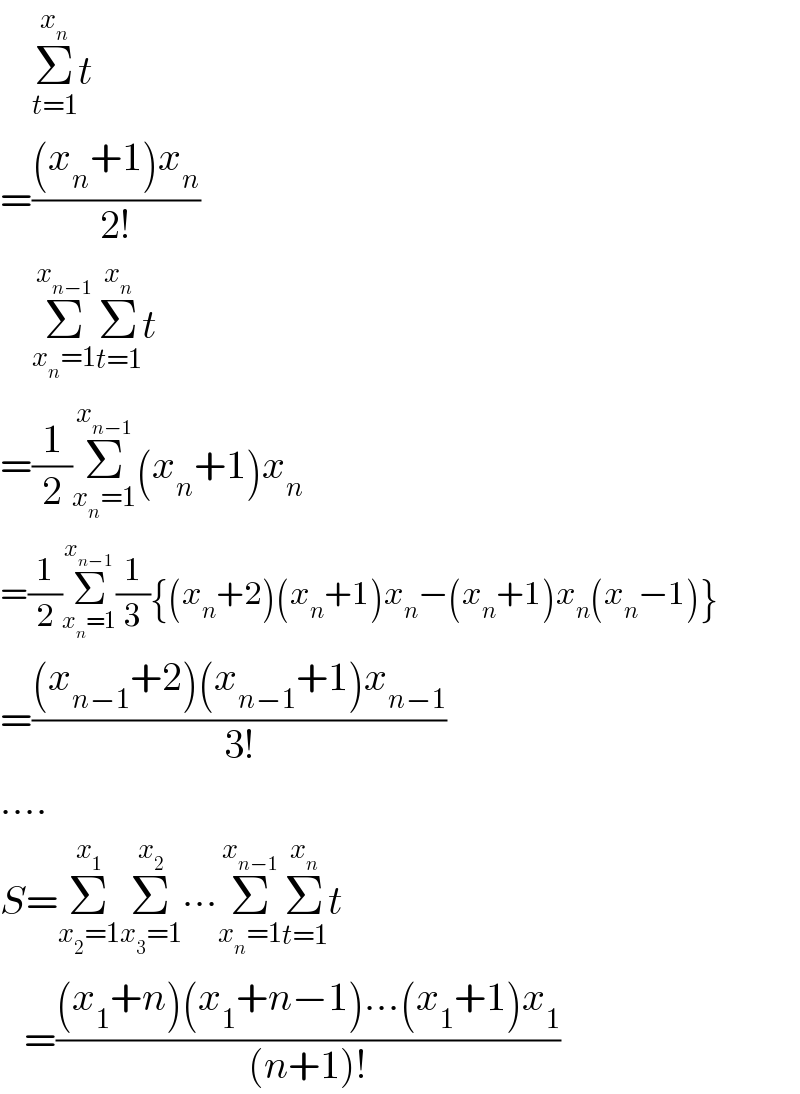
$$\:\:\:\:\underset{{t}=\mathrm{1}} {\overset{{x}_{{n}} } {\sum}}{t} \\ $$$$=\frac{\left({x}_{{n}} +\mathrm{1}\right){x}_{{n}} }{\mathrm{2}!} \\ $$$$\:\:\:\:\underset{{x}_{{n}} =\mathrm{1}} {\overset{{x}_{{n}−\mathrm{1}} } {\sum}}\underset{{t}=\mathrm{1}} {\overset{{x}_{{n}} } {\sum}}{t} \\ $$$$=\frac{\mathrm{1}}{\mathrm{2}}\underset{{x}_{{n}} =\mathrm{1}} {\overset{{x}_{{n}−\mathrm{1}} } {\sum}}\left({x}_{{n}} +\mathrm{1}\right){x}_{{n}} \\ $$$$=\frac{\mathrm{1}}{\mathrm{2}}\underset{{x}_{{n}} =\mathrm{1}} {\overset{{x}_{{n}−\mathrm{1}} } {\sum}}\frac{\mathrm{1}}{\mathrm{3}}\left\{\left({x}_{{n}} +\mathrm{2}\right)\left({x}_{{n}} +\mathrm{1}\right){x}_{{n}} −\left({x}_{{n}} +\mathrm{1}\right){x}_{{n}} \left({x}_{{n}} −\mathrm{1}\right)\right\} \\ $$$$=\frac{\left({x}_{{n}−\mathrm{1}} +\mathrm{2}\right)\left({x}_{{n}−\mathrm{1}} +\mathrm{1}\right){x}_{{n}−\mathrm{1}} }{\mathrm{3}!} \\ $$$$.... \\ $$$${S}=\underset{{x}_{\mathrm{2}} =\mathrm{1}} {\overset{{x}_{\mathrm{1}} } {\sum}}\underset{{x}_{\mathrm{3}} =\mathrm{1}} {\overset{{x}_{\mathrm{2}} } {\sum}}\centerdot\centerdot\centerdot\underset{{x}_{{n}} =\mathrm{1}} {\overset{{x}_{{n}−\mathrm{1}} } {\sum}}\underset{{t}=\mathrm{1}} {\overset{{x}_{{n}} } {\sum}}{t} \\ $$$$\:\:\:=\frac{\left({x}_{\mathrm{1}} +{n}\right)\left({x}_{\mathrm{1}} +{n}−\mathrm{1}\right)...\left({x}_{\mathrm{1}} +\mathrm{1}\right){x}_{\mathrm{1}} }{\left({n}+\mathrm{1}\right)!} \\ $$
Commented by mrW1 last updated on 16/May/17

$$\mathscr{N}{ice}! \\ $$
