
Question Number 95485 by O Predador last updated on 25/May/20
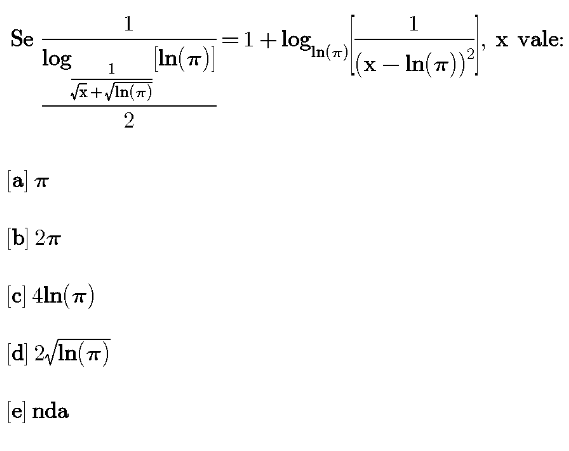
Commented by PRITHWISH SEN 2 last updated on 25/May/20

$$\mathrm{2llog}_{\mathrm{ln}\left(\pi\right)} \frac{\mathrm{1}}{\sqrt{\mathrm{x}}+\sqrt{\mathrm{ln}\left(\pi\right)}}\:−\mathrm{2log}_{\mathrm{ln}\left(\pi\right)} \frac{\mathrm{1}}{\mathrm{x}−\mathrm{lnx}}\:=\:\mathrm{1} \\ $$$$\mathrm{2log}_{\mathrm{ln}\left(\pi\right)} \frac{\mathrm{x}−\mathrm{ln}\pi}{\sqrt{\mathrm{x}}+\sqrt{\mathrm{ln}\left(\pi\right)}}\:=\:\mathrm{1} \\ $$$$\mathrm{log}_{\mathrm{ln}\pi} \left(\sqrt{\mathrm{x}}+\sqrt{\mathrm{ln}\pi}\right)^{\mathrm{2}} =\mathrm{1} \\ $$$$\mathrm{x}+\mathrm{2}\sqrt{\mathrm{x}\left(\mathrm{ln}\pi\right)}\:+\mathrm{ln}\pi=\mathrm{ln}\pi \\ $$$$\sqrt{\mathrm{x}}=−\mathrm{2}\sqrt{\mathrm{ln}\pi}\:\:\:\:\left\{\because\:\mathrm{x}\neq\mathrm{0}\:\right\} \\ $$$$\boldsymbol{\mathrm{x}}=\mathrm{4}\boldsymbol{\mathrm{ln}\pi} \\ $$$$ \\ $$
Commented by O Predador last updated on 25/May/20

$$\:\mathrm{Mito}! \\ $$
