Question and Answers Forum

All Questions      Topic List

Arithmetic Questions

Previous in All Question      Next in All Question      

Previous in Arithmetic      Next in Arithmetic      

Question Number 9460 by tawakalitu last updated on 09/Dec/16

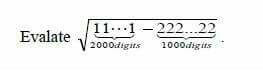
Answered by sou1618 last updated on 09/Dec/16

(√(11−2))=(√(10+1−2))=(√9)=3 (√(1111−22))=(√(1000+100+10+1−20−2))=(√(1089))=33 ...... X=(√(1111......11_(2000digits) −22...2_(1000digits) )) ∗1000_(4digits) =10^(4−1) X^2 =(10^(1999) +10^(1998) +...+10^1 +10^0 )−2(10^(999) +10^(998) +...+10^1 +10^0 ) =Σ_(k=1) ^(2000) 10^(k−1) −2Σ_(k=1) ^(1000) 10^(k−1) =((10^(2000) −1)/(10−1))−2((10^(1000) −1)/(10−1)) =(1/9)(10^(2000) −2×10^(1000) +1) =(1/3^2 ){(10^(1000) )^2 −2(10^(1000) )+1} =(1/3^2 )(10^(1000) −1)^2 ={(1/3)(1000...000_(1001digits) −1)}^2 X=(1/3)×999...999_(1000digits) =333...333_(1000digits) (√(11...1_(2n(digits)) −22...2_(n(digits)) ))=33...3_(n(digits))

$$\sqrt{\mathrm{11}−\mathrm{2}}=\sqrt{\mathrm{10}+\mathrm{1}−\mathrm{2}}=\sqrt{\mathrm{9}}=\mathrm{3} \\ $$$$\sqrt{\mathrm{1111}−\mathrm{22}}=\sqrt{\mathrm{1000}+\mathrm{100}+\mathrm{10}+\mathrm{1}−\mathrm{20}−\mathrm{2}}=\sqrt{\mathrm{1089}}=\mathrm{33} \\ $$$$...... \\ $$$${X}=\sqrt{\mathrm{1111}......\mathrm{11}_{\mathrm{2000}{digits}} −\mathrm{22}...\mathrm{2}_{\mathrm{1000}{digits}} } \\ $$$$\ast\mathrm{1000}_{\mathrm{4}{digits}} =\mathrm{10}^{\mathrm{4}−\mathrm{1}} \\ $$$${X}^{\mathrm{2}} =\left(\mathrm{10}^{\mathrm{1999}} +\mathrm{10}^{\mathrm{1998}} +...+\mathrm{10}^{\mathrm{1}} +\mathrm{10}^{\mathrm{0}} \right)−\mathrm{2}\left(\mathrm{10}^{\mathrm{999}} +\mathrm{10}^{\mathrm{998}} +...+\mathrm{10}^{\mathrm{1}} +\mathrm{10}^{\mathrm{0}} \right) \\ $$$$\:\:\:\:=\underset{{k}=\mathrm{1}} {\overset{\mathrm{2000}} {\sum}}\mathrm{10}^{{k}−\mathrm{1}} −\mathrm{2}\underset{{k}=\mathrm{1}} {\overset{\mathrm{1000}} {\sum}}\mathrm{10}^{{k}−\mathrm{1}} \\ $$$$\:\:\:\:=\frac{\mathrm{10}^{\mathrm{2000}} −\mathrm{1}}{\mathrm{10}−\mathrm{1}}−\mathrm{2}\frac{\mathrm{10}^{\mathrm{1000}} −\mathrm{1}}{\mathrm{10}−\mathrm{1}} \\ $$$$\:\:\:\:=\frac{\mathrm{1}}{\mathrm{9}}\left(\mathrm{10}^{\mathrm{2000}} −\mathrm{2}×\mathrm{10}^{\mathrm{1000}} +\mathrm{1}\right) \\ $$$$\:\:\:\:=\frac{\mathrm{1}}{\mathrm{3}^{\mathrm{2}} }\left\{\left(\mathrm{10}^{\mathrm{1000}} \right)^{\mathrm{2}} −\mathrm{2}\left(\mathrm{10}^{\mathrm{1000}} \right)+\mathrm{1}\right\} \\ $$$$\:\:\:\:=\frac{\mathrm{1}}{\mathrm{3}^{\mathrm{2}} }\left(\mathrm{10}^{\mathrm{1000}} −\mathrm{1}\right)^{\mathrm{2}} \\ $$$$\:\:\:\:=\left\{\frac{\mathrm{1}}{\mathrm{3}}\left(\mathrm{1000}...\mathrm{000}_{\mathrm{1001}{digits}} −\mathrm{1}\right)\right\}^{\mathrm{2}} \\ $$$${X}=\frac{\mathrm{1}}{\mathrm{3}}×\mathrm{999}...\mathrm{999}_{\mathrm{1000}{digits}} \\ $$$$\:\:\:\:=\mathrm{333}...\mathrm{333}_{\mathrm{1000}{digits}} \\ $$$$ \\ $$$$ \\ $$$$\sqrt{\mathrm{11}...\mathrm{1}_{\mathrm{2}{n}\left({digits}\right)} −\mathrm{22}...\mathrm{2}_{{n}\left({digits}\right)} }=\mathrm{33}...\mathrm{3}_{{n}\left({digits}\right)} \\ $$

Commented by tawakalitu last updated on 09/Dec/16

Thank you sir. God bless you.

$$\mathrm{Thank}\:\mathrm{you}\:\mathrm{sir}.\:\mathrm{God}\:\mathrm{bless}\:\mathrm{you}. \\ $$

Commented by tawakalitu last updated on 10/Dec/16

i really appreciate.

$$\mathrm{i}\:\mathrm{really}\:\mathrm{appreciate}. \\ $$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com