
Question Number 81421 by ajfour last updated on 12/Feb/20
Commented by ajfour last updated on 12/Feb/20
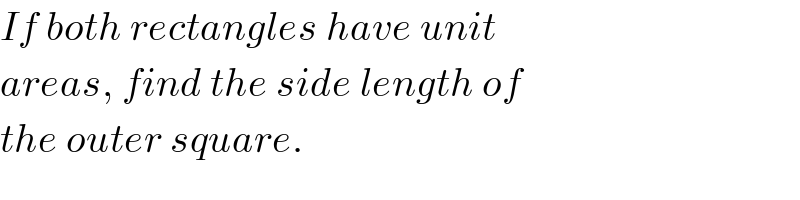
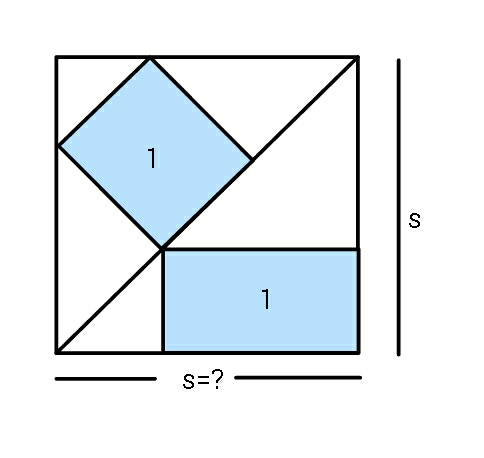
$${If}\:{both}\:{rectangles}\:{have}\:{unit} \\ $$$${areas},\:{find}\:{the}\:{side}\:{length}\:{of} \\ $$$${the}\:{outer}\:{square}. \\ $$
Answered by ajfour last updated on 12/Feb/20
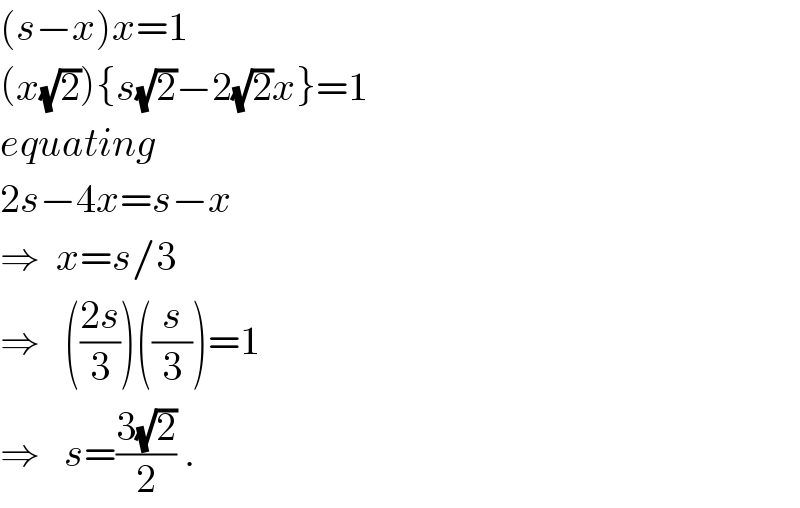
$$\left({s}−{x}\right){x}=\mathrm{1} \\ $$$$\left({x}\sqrt{\mathrm{2}}\right)\left\{{s}\sqrt{\mathrm{2}}−\mathrm{2}\sqrt{\mathrm{2}}{x}\right\}=\mathrm{1} \\ $$$${equating} \\ $$$$\mathrm{2}{s}−\mathrm{4}{x}={s}−{x} \\ $$$$\Rightarrow\:\:{x}={s}/\mathrm{3} \\ $$$$\Rightarrow\:\:\:\left(\frac{\mathrm{2}{s}}{\mathrm{3}}\right)\left(\frac{{s}}{\mathrm{3}}\right)=\mathrm{1}\:\:\: \\ $$$$\Rightarrow\:\:\:{s}=\frac{\mathrm{3}\sqrt{\mathrm{2}}}{\mathrm{2}}\:. \\ $$
