
Question Number 80230 by M±th+et£s last updated on 01/Feb/20
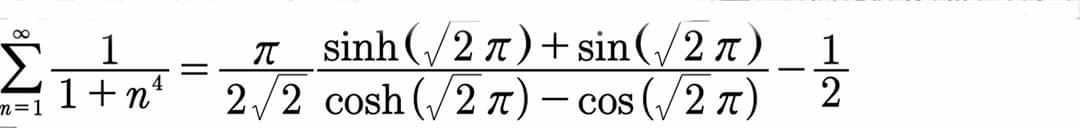
Answered by mind is power last updated on 03/Feb/20
![let f(z)=((πcot(πz))/(1+z^4 )),Main idee use Residus Th and evaluate Integrales in Two ways pols of f are n∈Z∪{e^(i(((1+2k)/4)}π) ,k∈{0,1,2,3}} Res(f,n)=(1/(1+n^4 )) Res(f,e^(i(((1+2k)/4))π) )=(π/(4e^(i3(((1+2k)/4))π) ))cot(πe^(i(((1+2k)/4))π) )=g(k) ∫_C_R f(z)dz=0 C_R =Re^(iθ) ,θ∈[0,2π] cause∣∫_C_R f(z)∣dz≤2πR.Max∣f(z)∣ since ∣cot(z)∣≤M ⇒Max(∣f(z)∣)≤((πM)/(R^4 −1)) ⇒∣∫_C_R f(z)dz∣≤((2πMR)/(R^4 −1))→0 as R→∞ Res th⇒∫_C_R f(z)dz=2iπΣ_(z∈C_R ) Res(f,z),R→∞ ⇒ ∫_C_R f(z)dz=2iπΣ_(n∈Z) (1/(1+n^4 ))+2iπΣ_(k=0) ^3 ((πcot(πe^(i(((1+2k)/4))π) ))/4)e^(−i3(((1+2k)/4))π) =0..1 ⇒Σ_(n∈Z) (1/(n^4 +1))=−Σ_(k=0) ^3 g(k) g(3)=g(1)^− g(2)=g(0)^� ⇒Σ_(k=0) ^3 g(k)=2Re{g(0)+g(1)} g(1)=g(0)^− ⇒Σ_(k=0) ^3 g(k)=4Re{g(0)} ⇒1⇔ Σ_(n∈Z) (1/(1+n^4 ))=−4Re{(π/4)cot(πe^(i(π/4)) )}e^((−3iπ)/4) =−πRe{cot((π/(√2))+i(π/(√2)))e^(−((3iπ)/4)) } =((π(√2))/2)Re{((cos((π/(√2)))ch((π/(√2)))−isin((π/(√2)))sh((π/(√2))))/(sin((π/(√2)))ch((π/(√2)))+icos((π/(√2)))sh((π/(√2)))))(1+i)} ⇔ multiply by (sin((π/(√2)))ch((π/(√2)))−icos((π/(√2)))sh((π/(√2)))) =((π(√2))/2){((((sin(π(√2)))/2)−i((sh(π(√2)))/2))/(sin^2 ((π/(√2)))+sh^2 ((π/(√2)))))(1+i)} =((π(√2))/2){((sin(π(√2))−ish(π(√2)))/(ch(π(√2))−cos(π(√2))))(1+i)} =((π(√2))/2) ((sin(π(√2))+sh(π(√2)))/(ch(π(√2))−cos(π(√2))))=Σ_(n∈Z) (1/(1+n^4 ))=2Σ_(n≥1) (1/(n^4 +1))+1 ⇒Σ_(n≥1) (1/(1+n^4 ))=(π/(2(√2))) ((sin(π(√2))+sh(π(√2)))/(ch(π(√2))−cos(π(√2))))−(1/2)](Q80478.png)
$${let}\:{f}\left({z}\right)=\frac{\pi{cot}\left(\pi{z}\right)}{\mathrm{1}+{z}^{\mathrm{4}} },{Main}\:{idee}\:{use}\:{Residus}\:{Th} \\ $$$${and}\:{evaluate}\:{Integrales}\:{in}\:{Two}\:{ways} \\ $$$${pols}\:{of}\:{f}\:{are}\:\:\:{n}\in\mathbb{Z}\cup\left\{{e}^{{i}\left(\frac{\mathrm{1}+\mathrm{2}{k}}{\mathrm{4}}\right\}\pi} ,{k}\in\left\{\mathrm{0},\mathrm{1},\mathrm{2},\mathrm{3}\right\}\right\} \\ $$$${Res}\left({f},{n}\right)=\frac{\mathrm{1}}{\mathrm{1}+{n}^{\mathrm{4}} } \\ $$$${Res}\left({f},{e}^{{i}\left(\frac{\mathrm{1}+\mathrm{2}{k}}{\mathrm{4}}\right)\pi} \right)=\frac{\pi}{\mathrm{4}{e}^{{i}\mathrm{3}\left(\frac{\mathrm{1}+\mathrm{2}{k}}{\mathrm{4}}\right)\pi} }{cot}\left(\pi{e}^{{i}\left(\frac{\mathrm{1}+\mathrm{2}{k}}{\mathrm{4}}\right)\pi} \right)={g}\left({k}\right) \\ $$$$\int_{{C}_{{R}} } {f}\left({z}\right){dz}=\mathrm{0} \\ $$$${C}_{{R}} ={Re}^{{i}\theta} ,\theta\in\left[\mathrm{0},\mathrm{2}\pi\right] \\ $$$${cause}\mid\int_{{C}_{{R}} } {f}\left({z}\right)\mid{dz}\leqslant\mathrm{2}\pi{R}.{Max}\mid{f}\left({z}\right)\mid \\ $$$${since}\:\mid{cot}\left({z}\right)\mid\leqslant{M} \\ $$$$\Rightarrow{Max}\left(\mid{f}\left({z}\right)\mid\right)\leqslant\frac{\pi{M}}{{R}^{\mathrm{4}} −\mathrm{1}} \\ $$$$\Rightarrow\mid\int_{{C}_{{R}} } {f}\left({z}\right){dz}\mid\leqslant\frac{\mathrm{2}\pi{MR}}{{R}^{\mathrm{4}} −\mathrm{1}}\rightarrow\mathrm{0}\:\:{as}\:{R}\rightarrow\infty \\ $$$${Res}\:{th}\Rightarrow\int_{{C}_{{R}} } {f}\left({z}\right){dz}=\mathrm{2}{i}\pi\underset{{z}\in{C}_{{R}} } {\sum}{Res}\left({f},{z}\right),{R}\rightarrow\infty\:\Rightarrow \\ $$$$\int_{{C}_{{R}} } {f}\left({z}\right){dz}=\mathrm{2}{i}\pi\underset{{n}\in\mathbb{Z}} {\sum}\frac{\mathrm{1}}{\mathrm{1}+{n}^{\mathrm{4}} }+\mathrm{2}{i}\pi\underset{{k}=\mathrm{0}} {\overset{\mathrm{3}} {\sum}}\frac{\pi{cot}\left(\pi{e}^{{i}\left(\frac{\mathrm{1}+\mathrm{2}{k}}{\mathrm{4}}\right)\pi} \right)}{\mathrm{4}}{e}^{−{i}\mathrm{3}\left(\frac{\mathrm{1}+\mathrm{2}{k}}{\mathrm{4}}\right)\pi} =\mathrm{0}..\mathrm{1} \\ $$$$\Rightarrow\underset{{n}\in\mathbb{Z}} {\sum}\frac{\mathrm{1}}{{n}^{\mathrm{4}} +\mathrm{1}}=−\underset{{k}=\mathrm{0}} {\overset{\mathrm{3}} {\sum}}{g}\left({k}\right) \\ $$$${g}\left(\mathrm{3}\right)={g}\left(\mathrm{1}\overset{−} {\right)} \\ $$$${g}\left(\mathrm{2}\right)={g}\left(\mathrm{0}\bar {\right)} \\ $$$$\Rightarrow\underset{{k}=\mathrm{0}} {\overset{\mathrm{3}} {\sum}}{g}\left({k}\right)=\mathrm{2}{Re}\left\{{g}\left(\mathrm{0}\right)+{g}\left(\mathrm{1}\right)\right\} \\ $$$${g}\left(\mathrm{1}\right)={g}\left(\mathrm{0}\overset{−} {\right)}\Rightarrow\underset{{k}=\mathrm{0}} {\overset{\mathrm{3}} {\sum}}{g}\left({k}\right)=\mathrm{4}{Re}\left\{{g}\left(\mathrm{0}\right)\right\} \\ $$$$\Rightarrow\mathrm{1}\Leftrightarrow \\ $$$$\underset{{n}\in\mathbb{Z}} {\sum}\frac{\mathrm{1}}{\mathrm{1}+{n}^{\mathrm{4}} }=−\mathrm{4}{Re}\left\{\frac{\pi}{\mathrm{4}}{cot}\left(\pi{e}^{{i}\frac{\pi}{\mathrm{4}}} \right)\right\}{e}^{\frac{−\mathrm{3}{i}\pi}{\mathrm{4}}} \\ $$$$=−\pi{Re}\left\{{cot}\left(\frac{\pi}{\sqrt{\mathrm{2}}}+{i}\frac{\pi}{\sqrt{\mathrm{2}}}\right){e}^{−\frac{\mathrm{3}{i}\pi}{\mathrm{4}}} \right\} \\ $$$$=\frac{\pi\sqrt{\mathrm{2}}}{\mathrm{2}}{Re}\left\{\frac{{cos}\left(\frac{\pi}{\sqrt{\mathrm{2}}}\right){ch}\left(\frac{\pi}{\sqrt{\mathrm{2}}}\right)−{isin}\left(\frac{\pi}{\sqrt{\mathrm{2}}}\right){sh}\left(\frac{\pi}{\sqrt{\mathrm{2}}}\right)}{{sin}\left(\frac{\pi}{\sqrt{\mathrm{2}}}\right){ch}\left(\frac{\pi}{\sqrt{\mathrm{2}}}\right)+{icos}\left(\frac{\pi}{\sqrt{\mathrm{2}}}\right){sh}\left(\frac{\pi}{\sqrt{\mathrm{2}}}\right)}\left(\mathrm{1}+{i}\right)\right\} \\ $$$$\Leftrightarrow\:\:{multiply}\:{by}\:\:\:\left({sin}\left(\frac{\pi}{\sqrt{\mathrm{2}}}\right){ch}\left(\frac{\pi}{\sqrt{\mathrm{2}}}\right)−{icos}\left(\frac{\pi}{\sqrt{\mathrm{2}}}\right){sh}\left(\frac{\pi}{\sqrt{\mathrm{2}}}\right)\right) \\ $$$$=\frac{\pi\sqrt{\mathrm{2}}}{\mathrm{2}}\left\{\frac{\frac{{sin}\left(\pi\sqrt{\mathrm{2}}\right)}{\mathrm{2}}−{i}\frac{{sh}\left(\pi\sqrt{\mathrm{2}}\right)}{\mathrm{2}}}{{sin}^{\mathrm{2}} \left(\frac{\pi}{\sqrt{\mathrm{2}}}\right)+{sh}^{\mathrm{2}} \left(\frac{\pi}{\sqrt{\mathrm{2}}}\right)}\left(\mathrm{1}+{i}\right)\right\} \\ $$$$=\frac{\pi\sqrt{\mathrm{2}}}{\mathrm{2}}\left\{\frac{{sin}\left(\pi\sqrt{\mathrm{2}}\right)−{ish}\left(\pi\sqrt{\mathrm{2}}\right)}{{ch}\left(\pi\sqrt{\mathrm{2}}\right)−{cos}\left(\pi\sqrt{\mathrm{2}}\right)}\left(\mathrm{1}+{i}\right)\right\} \\ $$$$=\frac{\pi\sqrt{\mathrm{2}}}{\mathrm{2}}\:\:\frac{{sin}\left(\pi\sqrt{\mathrm{2}}\right)+{sh}\left(\pi\sqrt{\mathrm{2}}\right)}{{ch}\left(\pi\sqrt{\mathrm{2}}\right)−{cos}\left(\pi\sqrt{\mathrm{2}}\right)}=\underset{{n}\in\mathbb{Z}} {\sum}\frac{\mathrm{1}}{\mathrm{1}+{n}^{\mathrm{4}} }=\mathrm{2}\underset{{n}\geqslant\mathrm{1}} {\sum}\frac{\mathrm{1}}{{n}^{\mathrm{4}} +\mathrm{1}}+\mathrm{1} \\ $$$$\Rightarrow\underset{{n}\geqslant\mathrm{1}} {\sum}\frac{\mathrm{1}}{\mathrm{1}+{n}^{\mathrm{4}} }=\frac{\pi}{\mathrm{2}\sqrt{\mathrm{2}}}\:\:\frac{{sin}\left(\pi\sqrt{\mathrm{2}}\right)+{sh}\left(\pi\sqrt{\mathrm{2}}\right)}{{ch}\left(\pi\sqrt{\mathrm{2}}\right)−{cos}\left(\pi\sqrt{\mathrm{2}}\right)}−\frac{\mathrm{1}}{\mathrm{2}} \\ $$$$ \\ $$$$ \\ $$$$ \\ $$
Commented by M±th+et£s last updated on 03/Feb/20
$${god}\:{bless}\:{you}\:{sir}\:.\:{thank}\:{you} \\ $$
Commented by mind is power last updated on 03/Feb/20
$${Withe}\:{pleasur}\:{Sir}\:\: \\ $$
