
Question Number 7026 by Tawakalitu. last updated on 07/Aug/16
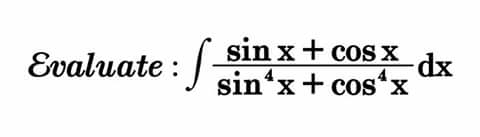
Commented by Yozzii last updated on 07/Aug/16

$${Write}\:\int\frac{{sinx}+{cosx}}{{sin}^{\mathrm{4}} {x}+{cos}^{\mathrm{4}} {x}}{dx}=\int\frac{{sinx}}{{sin}^{\mathrm{4}} {x}+{cos}^{\mathrm{4}} {x}}{dx}+\int\frac{{cosx}}{{sin}^{\mathrm{4}} {x}+{cos}^{\mathrm{4}} {x}}{dx}. \\ $$$${Evaluate}\:{each}\:{integral}\:{by}\:{the}\:{following}\:{steps} \\ $$$$\left(\mathrm{1}\right)\:{Substitute}\:{u}={cosx}\:{in}\:\int\frac{{sinx}}{{sin}^{\mathrm{4}} {x}+{cos}^{\mathrm{4}} {x}}{dx} \\ $$$${where}\:{sin}^{\mathrm{4}} {x}+{cos}^{\mathrm{4}} {x}=\mathrm{2}{cos}^{\mathrm{4}} {x}−\mathrm{2}{cos}^{\mathrm{2}} {x}+\mathrm{1},\: \\ $$$$\left\{{and}\:{substitute}\:{k}={sinx}\:{in}\:\int\frac{{cosx}}{{sin}^{\mathrm{4}} {x}+{cos}^{\mathrm{4}} {x}}{dx}\right. \\ $$$$\left.{where}\:{sin}^{\mathrm{4}} {x}+{cos}^{\mathrm{4}} {x}=\mathrm{2}{sin}^{\mathrm{4}} {x}−\mathrm{2}{sin}^{\mathrm{2}} {x}+\mathrm{1}.\right\} \\ $$$$\left(\mathrm{2}\right)\:{Complete}\:{the}\:{square}\:{in}\:{the}\:{denominators}\:{of} \\ $$$${the}\:{resulting}\:{integrand}. \\ $$$${e}.{g}\:\mathrm{2}{u}^{\mathrm{4}} −\mathrm{2}{u}^{\mathrm{2}} +\mathrm{1}=\mathrm{0}.\mathrm{5}\left(\left(\mathrm{2}{u}^{\mathrm{2}} −\mathrm{1}\right)^{\mathrm{2}} +\mathrm{1}\right). \\ $$$$\left(\mathrm{3}\right){Apply}\:{the}\:{identity}\:{z}^{\mathrm{2}} +\mathrm{1}=\left({z}−{i}\right)\left({z}+{i}\right) \\ $$$${with}\:{z}=\mathrm{2}{u}^{\mathrm{2}} −\mathrm{1}\:{for}\:{the}\:{first}\:{integrand}\: \\ $$$${for}\:{example}. \\ $$$$\Rightarrow\left(\mathrm{2}{u}^{\mathrm{2}} −\mathrm{1}\right)^{\mathrm{2}} +\mathrm{1}=\left(\mathrm{2}{u}^{\mathrm{2}} −\mathrm{1}−{i}\right)\left(\mathrm{2}{u}^{\mathrm{2}} −\mathrm{1}+{i}\right) \\ $$$${or}\:\left(\mathrm{2}{u}^{\mathrm{2}} −\mathrm{1}\right)^{\mathrm{2}} +\mathrm{1}=\left(\sqrt{\mathrm{2}}{u}−\sqrt{\mathrm{1}+{i}}\right)\left(\sqrt{\mathrm{2}}{u}+\sqrt{\mathrm{1}+{i}}\right)\left(\mathrm{2}{u}^{\mathrm{2}} −\mathrm{1}+{i}\right) \\ $$$$\left(\mathrm{4}\right){Split}\:\frac{\mathrm{2}}{\left(\mathrm{2}{u}^{\mathrm{2}} −\mathrm{1}\right)^{\mathrm{2}} +\mathrm{1}}\:{into}\:{partial}\:{fractions}. \\ $$$${Solve}\:{for}\:{a},{b},{c},{d}\in\mathbb{C}\:{in} \\ $$$$\frac{\mathrm{2}}{\left(\mathrm{2}{u}^{\mathrm{2}} −\mathrm{1}\right)^{\mathrm{2}} +\mathrm{1}}\equiv\frac{{a}}{\sqrt{\mathrm{2}}{u}−\sqrt{\mathrm{1}+{i}}}+\frac{{b}}{\sqrt{\mathrm{2}}{u}+\sqrt{\mathrm{1}+{i}}}+\frac{{cu}+{d}}{\mathrm{2}{u}^{\mathrm{2}} +{i}−\mathrm{1}} \\ $$$$\left(\mathrm{5}\right){Then}\:{integrate}\:{and}\:{simplify}\:{as}\:{usual} \\ $$$${e}.{g}:\:\int\frac{{sinx}}{{sin}^{\mathrm{4}} {x}+{cos}^{\mathrm{4}} {x}}{dx}=−\int\left\{\frac{{a}}{\sqrt{\mathrm{2}}{u}−\sqrt{\mathrm{1}+{i}}}+\frac{{b}}{\sqrt{\mathrm{2}}{u}+\sqrt{\mathrm{1}+{i}}}+\frac{{cu}+{d}}{\mathrm{2}{u}^{\mathrm{2}} +{i}−\mathrm{1}}\right\}{du}=−{F}\left({u}\right)+{C}=−{F}\left({cosx}\right)+{C} \\ $$$${where}\:\frac{{d}}{{du}}{F}\left({u}\right)=\frac{{a}}{\sqrt{\mathrm{2}}{u}−\sqrt{\mathrm{1}+{i}}}+\frac{{b}}{\sqrt{\mathrm{2}}{u}+\sqrt{\mathrm{1}+{i}}}+\frac{{cu}+{d}}{\mathrm{2}{u}^{\mathrm{2}} +{i}−\mathrm{1}}. \\ $$$$−−−−−−−−−−−−−−−−−−−−−−−−−− \\ $$$${For}\:\int\frac{{cosx}}{{sin}^{\mathrm{4}} {x}+{cos}^{\mathrm{4}} {x}}{dx},\:{this}\:{turns}\:{out}\:{to}\:{be} \\ $$$$\int\frac{{cosx}}{{sin}^{\mathrm{4}} {x}+{cos}^{\mathrm{4}} {x}}{dx}={F}\left({k}\right)+{T}={F}\left({sinx}\right)+{T} \\ $$$$\therefore\:\int\frac{{sinx}+{cosx}}{{sin}^{\mathrm{4}} {x}+{cos}^{\mathrm{4}} {x}}{dx}={F}\left({sinx}\right)−{F}\left({cosx}\right)+{constant}. \\ $$
Commented by Tawakalitu. last updated on 07/Aug/16
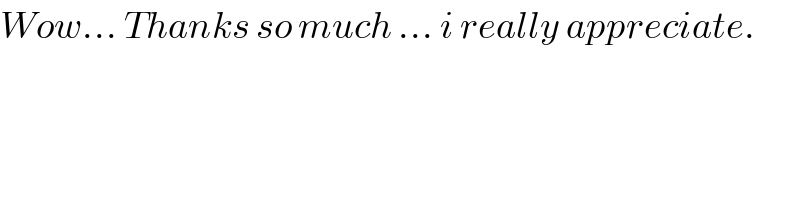
$${Wow}...\:{Thanks}\:{so}\:{much}\:...\:{i}\:{really}\:{appreciate}. \\ $$
Commented by Tawakalitu. last updated on 07/Aug/16
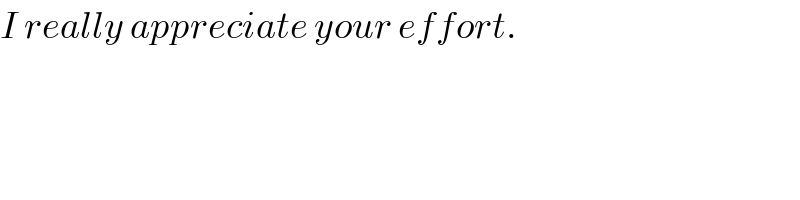
$${I}\:{really}\:{appreciate}\:{your}\:{effort}. \\ $$
Commented by Tawakalitu. last updated on 07/Aug/16

Commented by Tawakalitu. last updated on 07/Aug/16
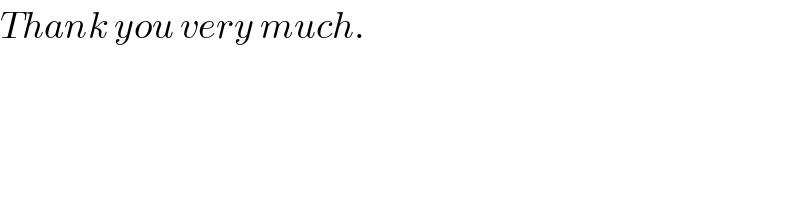
$${Thank}\:{you}\:{very}\:{much}. \\ $$
