Question and Answers Forum

All Questions      Topic List

Algebra Questions

Previous in All Question      Next in All Question      

Previous in Algebra      Next in Algebra      

Question Number 69741 by ajfour last updated on 27/Sep/19

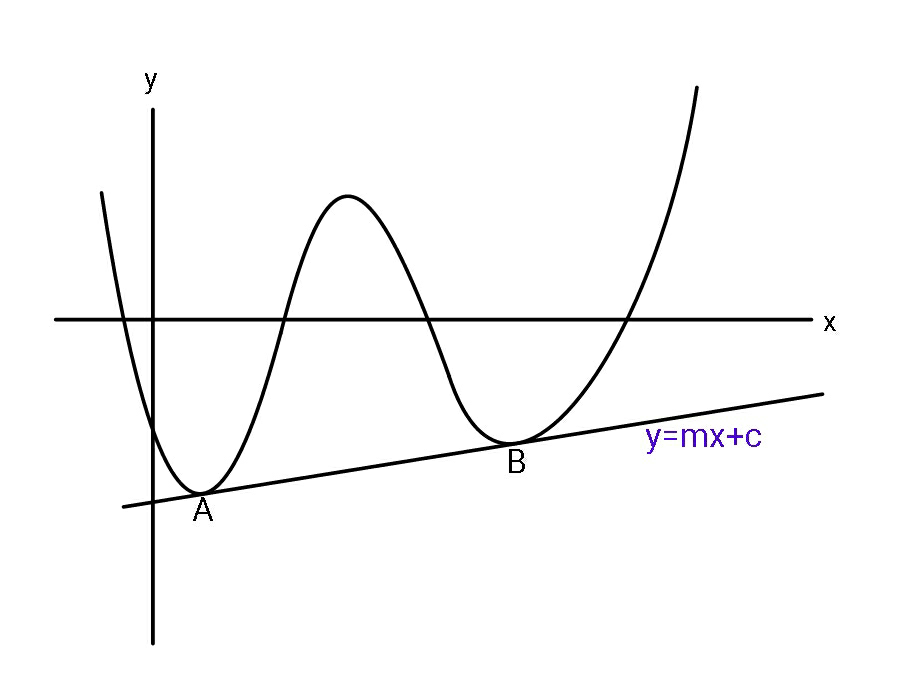
Commented by mr W last updated on 27/Sep/19

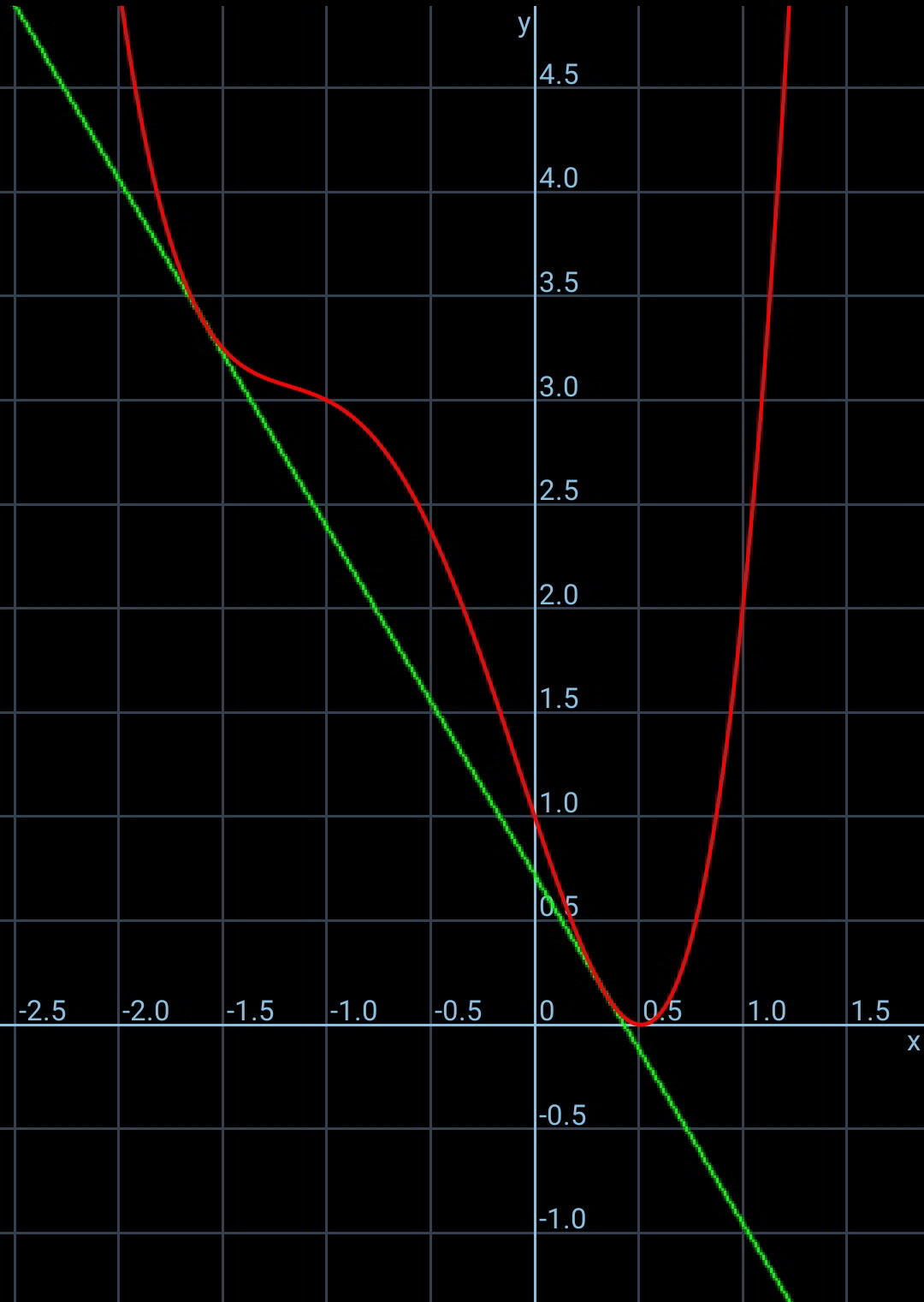
Commented by mr W last updated on 27/Sep/19

Commented by mr W last updated on 27/Sep/19

Commented by ajfour last updated on 27/Sep/19

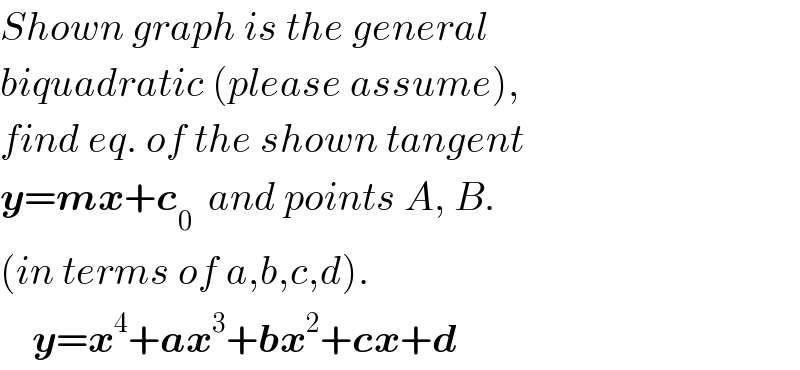
Shown graph is the general biquadratic (please assume), find eq. of the shown tangent y=mx+c_0 and points A, B. (in terms of a,b,c,d). y=x^4 +ax^3 +bx^2 +cx+d

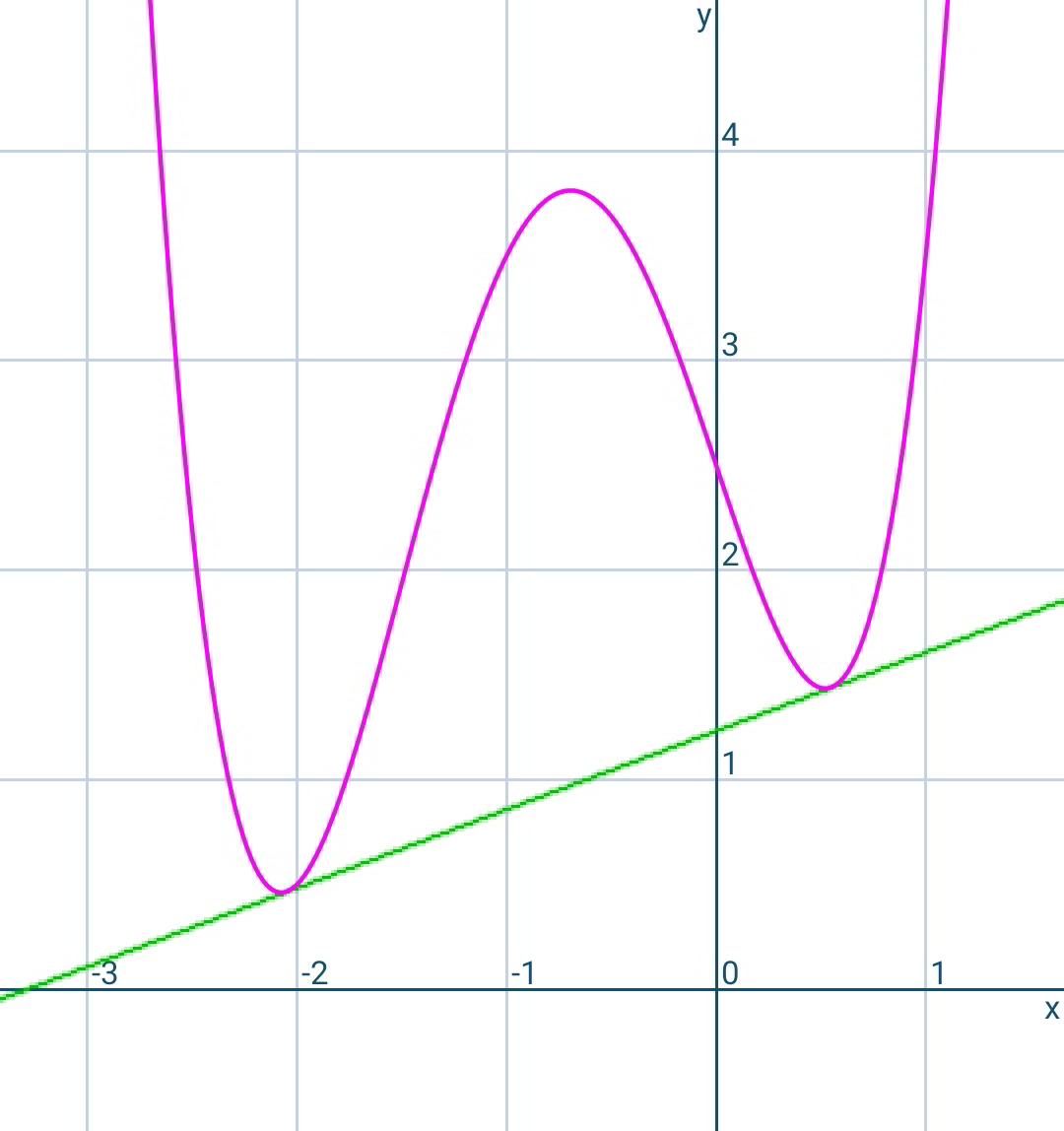
$${Shown}\:{graph}\:{is}\:{the}\:{general} \\ $$$${biquadratic}\:\left({please}\:{assume}\right), \\ $$$${find}\:{eq}.\:{of}\:{the}\:{shown}\:{tangent} \\ $$$$\boldsymbol{{y}}=\boldsymbol{{mx}}+\boldsymbol{{c}}_{\mathrm{0}} \:\:{and}\:{points}\:{A},\:{B}. \\ $$$$\left({in}\:{terms}\:{of}\:{a},{b},{c},{d}\right). \\ $$$$\:\:\:\:\boldsymbol{{y}}=\boldsymbol{{x}}^{\mathrm{4}} +\boldsymbol{{ax}}^{\mathrm{3}} +\boldsymbol{{bx}}^{\mathrm{2}} +\boldsymbol{{cx}}+\boldsymbol{{d}} \\ $$

Answered by mr W last updated on 28/Sep/19

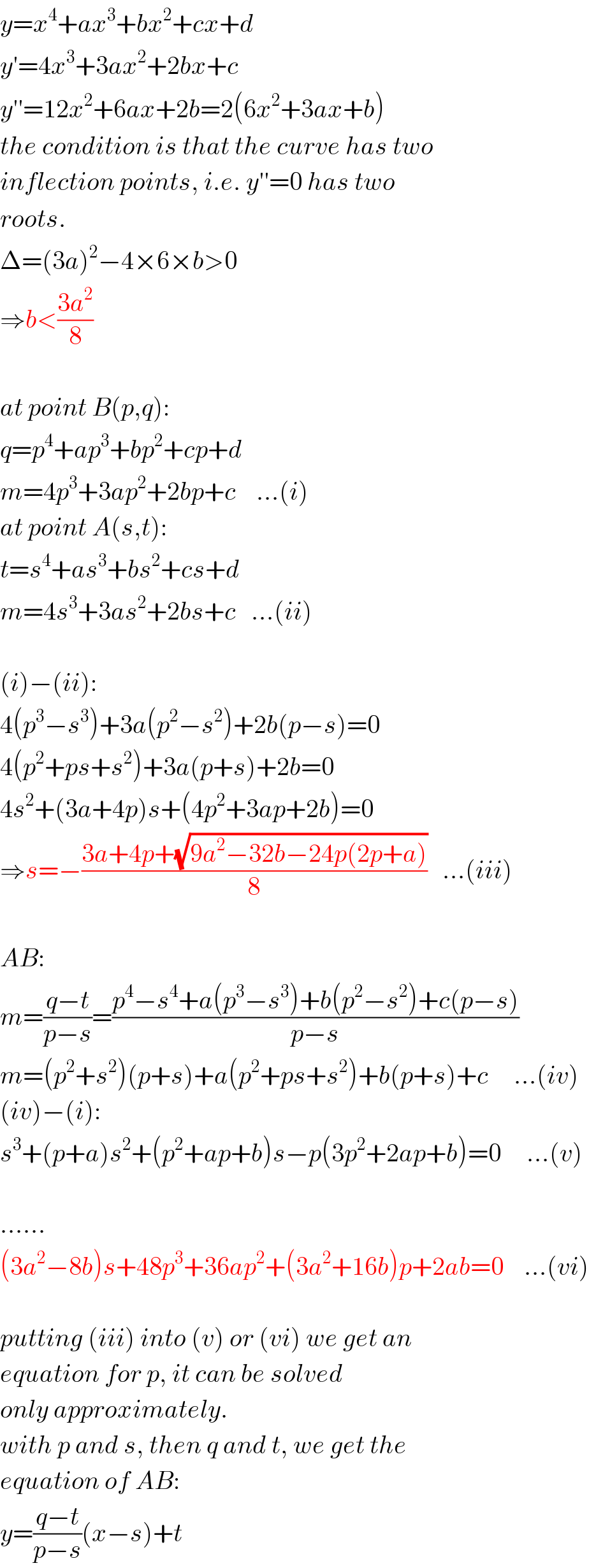
y=x^4 +ax^3 +bx^2 +cx+d y′=4x^3 +3ax^2 +2bx+c y′′=12x^2 +6ax+2b=2(6x^2 +3ax+b) the condition is that the curve has two inflection points, i.e. y′′=0 has two roots. Δ=(3a)^2 −4×6×b>0 ⇒b<((3a^2 )/8) at point B(p,q): q=p^4 +ap^3 +bp^2 +cp+d m=4p^3 +3ap^2 +2bp+c ...(i) at point A(s,t): t=s^4 +as^3 +bs^2 +cs+d m=4s^3 +3as^2 +2bs+c ...(ii) (i)−(ii): 4(p^3 −s^3 )+3a(p^2 −s^2 )+2b(p−s)=0 4(p^2 +ps+s^2 )+3a(p+s)+2b=0 4s^2 +(3a+4p)s+(4p^2 +3ap+2b)=0 ⇒s=−((3a+4p+(√(9a^2 −32b−24p(2p+a))))/8) ...(iii) AB: m=((q−t)/(p−s))=((p^4 −s^4 +a(p^3 −s^3 )+b(p^2 −s^2 )+c(p−s))/(p−s)) m=(p^2 +s^2 )(p+s)+a(p^2 +ps+s^2 )+b(p+s)+c ...(iv) (iv)−(i): s^3 +(p+a)s^2 +(p^2 +ap+b)s−p(3p^2 +2ap+b)=0 ...(v) ...... (3a^2 −8b)s+48p^3 +36ap^2 +(3a^2 +16b)p+2ab=0 ...(vi) putting (iii) into (v) or (vi) we get an equation for p, it can be solved only approximately. with p and s, then q and t, we get the equation of AB: y=((q−t)/(p−s))(x−s)+t

$${y}={x}^{\mathrm{4}} +{ax}^{\mathrm{3}} +{bx}^{\mathrm{2}} +{cx}+{d} \\ $$$${y}'=\mathrm{4}{x}^{\mathrm{3}} +\mathrm{3}{ax}^{\mathrm{2}} +\mathrm{2}{bx}+{c} \\ $$$${y}''=\mathrm{12}{x}^{\mathrm{2}} +\mathrm{6}{ax}+\mathrm{2}{b}=\mathrm{2}\left(\mathrm{6}{x}^{\mathrm{2}} +\mathrm{3}{ax}+{b}\right) \\ $$$${the}\:{condition}\:{is}\:{that}\:{the}\:{curve}\:{has}\:{two} \\ $$$${inflection}\:{points},\:{i}.{e}.\:{y}''=\mathrm{0}\:{has}\:{two} \\ $$$${roots}. \\ $$$$\Delta=\left(\mathrm{3}{a}\right)^{\mathrm{2}} −\mathrm{4}×\mathrm{6}×{b}>\mathrm{0} \\ $$$$\Rightarrow{b}<\frac{\mathrm{3}{a}^{\mathrm{2}} }{\mathrm{8}} \\ $$$$ \\ $$$${at}\:{point}\:{B}\left({p},{q}\right): \\ $$$${q}={p}^{\mathrm{4}} +{ap}^{\mathrm{3}} +{bp}^{\mathrm{2}} +{cp}+{d} \\ $$$${m}=\mathrm{4}{p}^{\mathrm{3}} +\mathrm{3}{ap}^{\mathrm{2}} +\mathrm{2}{bp}+{c}\:\:\:\:...\left({i}\right) \\ $$$${at}\:{point}\:{A}\left({s},{t}\right): \\ $$$${t}={s}^{\mathrm{4}} +{as}^{\mathrm{3}} +{bs}^{\mathrm{2}} +{cs}+{d} \\ $$$${m}=\mathrm{4}{s}^{\mathrm{3}} +\mathrm{3}{as}^{\mathrm{2}} +\mathrm{2}{bs}+{c}\:\:\:...\left({ii}\right) \\ $$$$ \\ $$$$\left({i}\right)−\left({ii}\right): \\ $$$$\mathrm{4}\left({p}^{\mathrm{3}} −{s}^{\mathrm{3}} \right)+\mathrm{3}{a}\left({p}^{\mathrm{2}} −{s}^{\mathrm{2}} \right)+\mathrm{2}{b}\left({p}−{s}\right)=\mathrm{0} \\ $$$$\mathrm{4}\left({p}^{\mathrm{2}} +{ps}+{s}^{\mathrm{2}} \right)+\mathrm{3}{a}\left({p}+{s}\right)+\mathrm{2}{b}=\mathrm{0} \\ $$$$\mathrm{4}{s}^{\mathrm{2}} +\left(\mathrm{3}{a}+\mathrm{4}{p}\right){s}+\left(\mathrm{4}{p}^{\mathrm{2}} +\mathrm{3}{ap}+\mathrm{2}{b}\right)=\mathrm{0} \\ $$$$\Rightarrow{s}=−\frac{\mathrm{3}{a}+\mathrm{4}{p}+\sqrt{\mathrm{9}{a}^{\mathrm{2}} −\mathrm{32}{b}−\mathrm{24}{p}\left(\mathrm{2}{p}+{a}\right)}}{\mathrm{8}}\:\:\:...\left({iii}\right) \\ $$$$ \\ $$$${AB}: \\ $$$${m}=\frac{{q}−{t}}{{p}−{s}}=\frac{{p}^{\mathrm{4}} −{s}^{\mathrm{4}} +{a}\left({p}^{\mathrm{3}} −{s}^{\mathrm{3}} \right)+{b}\left({p}^{\mathrm{2}} −{s}^{\mathrm{2}} \right)+{c}\left({p}−{s}\right)}{{p}−{s}} \\ $$$${m}=\left({p}^{\mathrm{2}} +{s}^{\mathrm{2}} \right)\left({p}+{s}\right)+{a}\left({p}^{\mathrm{2}} +{ps}+{s}^{\mathrm{2}} \right)+{b}\left({p}+{s}\right)+{c}\:\:\:\:\:...\left({iv}\right) \\ $$$$\left({iv}\right)−\left({i}\right): \\ $$$${s}^{\mathrm{3}} +\left({p}+{a}\right){s}^{\mathrm{2}} +\left({p}^{\mathrm{2}} +{ap}+{b}\right){s}−{p}\left(\mathrm{3}{p}^{\mathrm{2}} +\mathrm{2}{ap}+{b}\right)=\mathrm{0}\:\:\:\:\:...\left({v}\right) \\ $$$$ \\ $$$$...... \\ $$$$\left(\mathrm{3}{a}^{\mathrm{2}} −\mathrm{8}{b}\right){s}+\mathrm{48}{p}^{\mathrm{3}} +\mathrm{36}{ap}^{\mathrm{2}} +\left(\mathrm{3}{a}^{\mathrm{2}} +\mathrm{16}{b}\right){p}+\mathrm{2}{ab}=\mathrm{0}\:\:\:\:...\left({vi}\right) \\ $$$$ \\ $$$${putting}\:\left({iii}\right)\:{into}\:\left({v}\right)\:{or}\:\left({vi}\right)\:{we}\:{get}\:{an} \\ $$$${equation}\:{for}\:{p},\:{it}\:{can}\:{be}\:{solved} \\ $$$${only}\:{approximately}. \\ $$$${with}\:{p}\:{and}\:{s},\:{then}\:{q}\:{and}\:{t},\:{we}\:{get}\:{the} \\ $$$${equation}\:{of}\:{AB}: \\ $$$${y}=\frac{{q}−{t}}{{p}−{s}}\left({x}−{s}\right)+{t} \\ $$

Commented by mr W last updated on 28/Sep/19

Commented by ajfour last updated on 28/Sep/19

Thanks Sir, please view my attempt..

$${Thanks}\:{Sir},\:{please}\:{view}\:{my}\: \\ $$$${attempt}.. \\ $$

Answered by ajfour last updated on 28/Sep/19

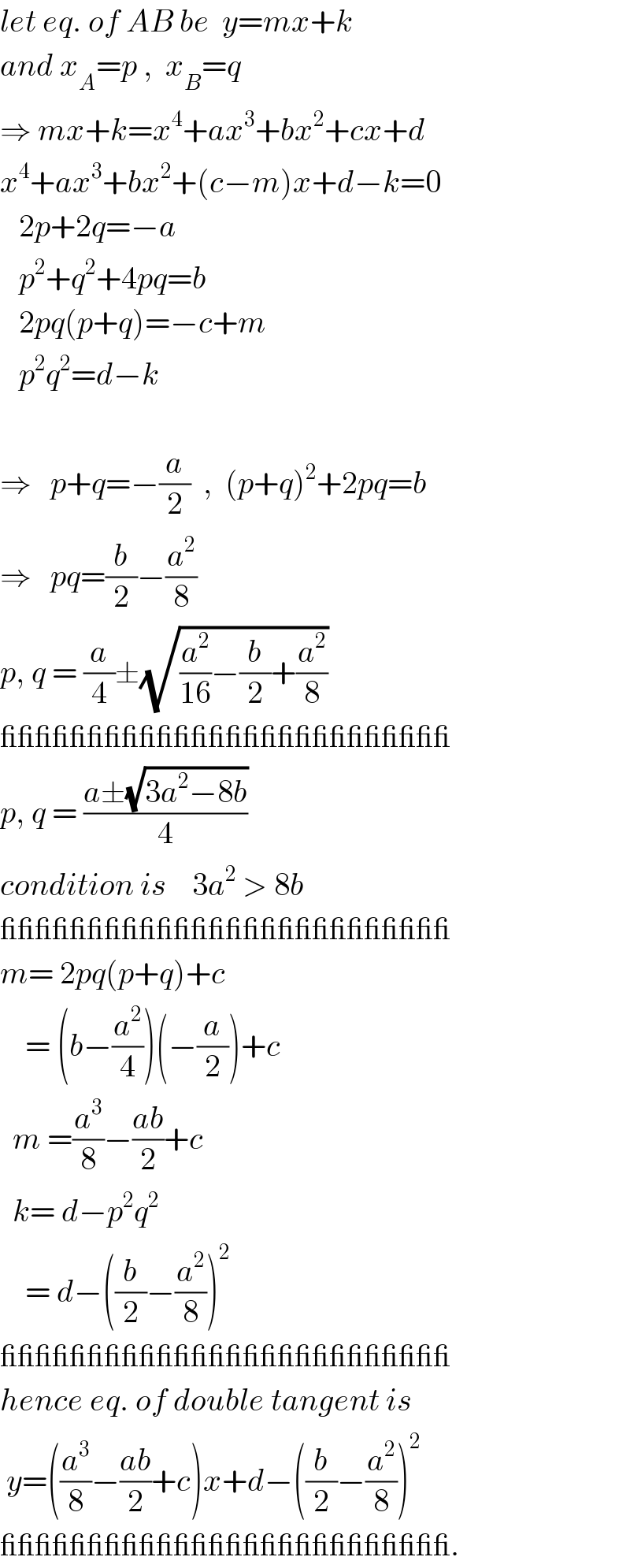
let eq. of AB be y=mx+k and x_A =p , x_B =q ⇒ mx+k=x^4 +ax^3 +bx^2 +cx+d x^4 +ax^3 +bx^2 +(c−m)x+d−k=0 2p+2q=−a p^2 +q^2 +4pq=b 2pq(p+q)=−c+m p^2 q^2 =d−k ⇒ p+q=−(a/2) , (p+q)^2 +2pq=b ⇒ pq=(b/2)−(a^2 /8) p, q = (a/4)±(√((a^2 /(16))−(b/2)+(a^2 /8))) __________________________ p, q = ((a±(√(3a^2 −8b)))/4) condition is 3a^2 > 8b __________________________ m= 2pq(p+q)+c = (b−(a^2 /4))(−(a/2))+c m =(a^3 /8)−((ab)/2)+c k= d−p^2 q^2 = d−((b/2)−(a^2 /8))^2 __________________________ hence eq. of double tangent is y=((a^3 /8)−((ab)/2)+c)x+d−((b/2)−(a^2 /8))^2 __________________________.

$${let}\:{eq}.\:{of}\:{AB}\:{be}\:\:{y}={mx}+{k} \\ $$$${and}\:{x}_{{A}} ={p}\:,\:\:{x}_{{B}} ={q} \\ $$$$\Rightarrow\:{mx}+{k}={x}^{\mathrm{4}} +{ax}^{\mathrm{3}} +{bx}^{\mathrm{2}} +{cx}+{d} \\ $$$${x}^{\mathrm{4}} +{ax}^{\mathrm{3}} +{bx}^{\mathrm{2}} +\left({c}−{m}\right){x}+{d}−{k}=\mathrm{0} \\ $$$$\:\:\:\mathrm{2}{p}+\mathrm{2}{q}=−{a} \\ $$$$\:\:\:{p}^{\mathrm{2}} +{q}^{\mathrm{2}} +\mathrm{4}{pq}={b} \\ $$$$\:\:\:\mathrm{2}{pq}\left({p}+{q}\right)=−{c}+{m} \\ $$$$\:\:\:{p}^{\mathrm{2}} {q}^{\mathrm{2}} ={d}−{k} \\ $$$$ \\ $$$$\Rightarrow\:\:\:{p}+{q}=−\frac{{a}}{\mathrm{2}}\:\:,\:\:\left({p}+{q}\right)^{\mathrm{2}} +\mathrm{2}{pq}={b} \\ $$$$\Rightarrow\:\:\:{pq}=\frac{{b}}{\mathrm{2}}−\frac{{a}^{\mathrm{2}} }{\mathrm{8}} \\ $$$${p},\:{q}\:=\:\frac{{a}}{\mathrm{4}}\pm\sqrt{\frac{{a}^{\mathrm{2}} }{\mathrm{16}}−\frac{{b}}{\mathrm{2}}+\frac{{a}^{\mathrm{2}} }{\mathrm{8}}} \\ $$$$\_\_\_\_\_\_\_\_\_\_\_\_\_\_\_\_\_\_\_\_\_\_\_\_\_\_ \\ $$$${p},\:{q}\:=\:\frac{{a}\pm\sqrt{\mathrm{3}{a}^{\mathrm{2}} −\mathrm{8}{b}}}{\mathrm{4}} \\ $$$${condition}\:{is}\:\:\:\:\mathrm{3}{a}^{\mathrm{2}} \:>\:\mathrm{8}{b} \\ $$$$\_\_\_\_\_\_\_\_\_\_\_\_\_\_\_\_\_\_\_\_\_\_\_\_\_\_ \\ $$$${m}=\:\mathrm{2}{pq}\left({p}+{q}\right)+{c} \\ $$$$\:\:\:\:=\:\left({b}−\frac{{a}^{\mathrm{2}} }{\mathrm{4}}\right)\left(−\frac{{a}}{\mathrm{2}}\right)+{c} \\ $$$$\:\:{m}\:=\frac{{a}^{\mathrm{3}} }{\mathrm{8}}−\frac{{ab}}{\mathrm{2}}+{c} \\ $$$$\:\:{k}=\:{d}−{p}^{\mathrm{2}} {q}^{\mathrm{2}} \\ $$$$\:\:\:\:=\:{d}−\left(\frac{{b}}{\mathrm{2}}−\frac{{a}^{\mathrm{2}} }{\mathrm{8}}\right)^{\mathrm{2}} \\ $$$$\_\_\_\_\_\_\_\_\_\_\_\_\_\_\_\_\_\_\_\_\_\_\_\_\_\_ \\ $$$${hence}\:{eq}.\:{of}\:{double}\:{tangent}\:{is} \\ $$$$\:{y}=\left(\frac{{a}^{\mathrm{3}} }{\mathrm{8}}−\frac{{ab}}{\mathrm{2}}+{c}\right){x}+{d}−\left(\frac{{b}}{\mathrm{2}}−\frac{{a}^{\mathrm{2}} }{\mathrm{8}}\right)^{\mathrm{2}} \: \\ $$$$\_\_\_\_\_\_\_\_\_\_\_\_\_\_\_\_\_\_\_\_\_\_\_\_\_\_. \\ $$

Commented by mr W last updated on 28/Sep/19

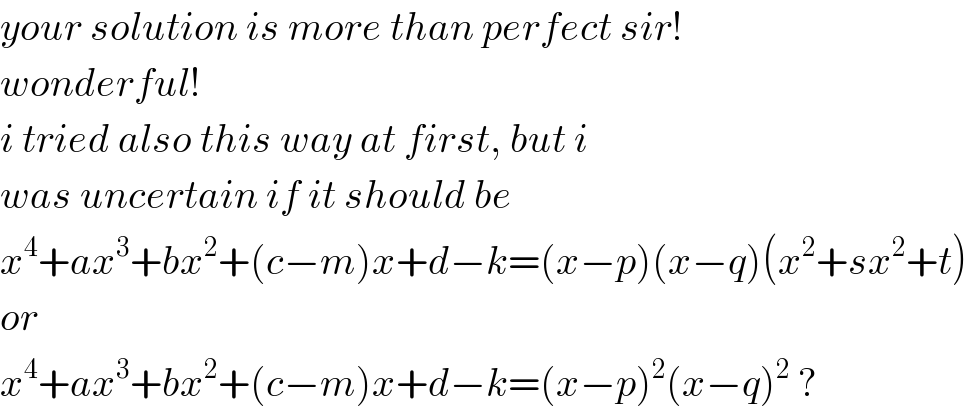
your solution is more than perfect sir! wonderful! i tried also this way at first, but i was uncertain if it should be x^4 +ax^3 +bx^2 +(c−m)x+d−k=(x−p)(x−q)(x^2 +sx^2 +t) or x^4 +ax^3 +bx^2 +(c−m)x+d−k=(x−p)^2 (x−q)^2 ?

$${your}\:{solution}\:{is}\:{more}\:{than}\:{perfect}\:{sir}! \\ $$$${wonderful}! \\ $$$${i}\:{tried}\:{also}\:{this}\:{way}\:{at}\:{first},\:{but}\:{i} \\ $$$${was}\:{uncertain}\:{if}\:{it}\:{should}\:{be} \\ $$$${x}^{\mathrm{4}} +{ax}^{\mathrm{3}} +{bx}^{\mathrm{2}} +\left({c}−{m}\right){x}+{d}−{k}=\left({x}−{p}\right)\left({x}−{q}\right)\left({x}^{\mathrm{2}} +{sx}^{\mathrm{2}} +{t}\right) \\ $$$${or} \\ $$$${x}^{\mathrm{4}} +{ax}^{\mathrm{3}} +{bx}^{\mathrm{2}} +\left({c}−{m}\right){x}+{d}−{k}=\left({x}−{p}\right)^{\mathrm{2}} \left({x}−{q}\right)^{\mathrm{2}} \:? \\ $$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com