Question and Answers Forum

All Questions      Topic List

Mechanics Questions

Previous in All Question      Next in All Question      

Previous in Mechanics      Next in Mechanics      

Question Number 68342 by Tony Lin last updated on 09/Sep/19

Commented by Tony Lin last updated on 09/Sep/19

when m slide to the bottom if there is no fraction find v_m &v_M find a_m &a_M

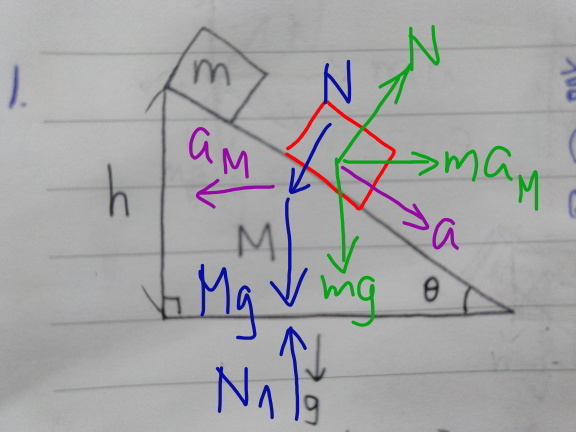
$${when}\:{m}\:{slide}\:{to}\:{the}\:{bottom} \\ $$$${if}\:{there}\:{is}\:{no}\:{fraction} \\ $$$${find}\:{v}_{{m}} \&{v}_{{M}} \\ $$$${find}\:{a}_{{m}} \&{a}_{{M}} \\ $$

Answered by mr W last updated on 09/Sep/19

Commented by mr W last updated on 10/Sep/19

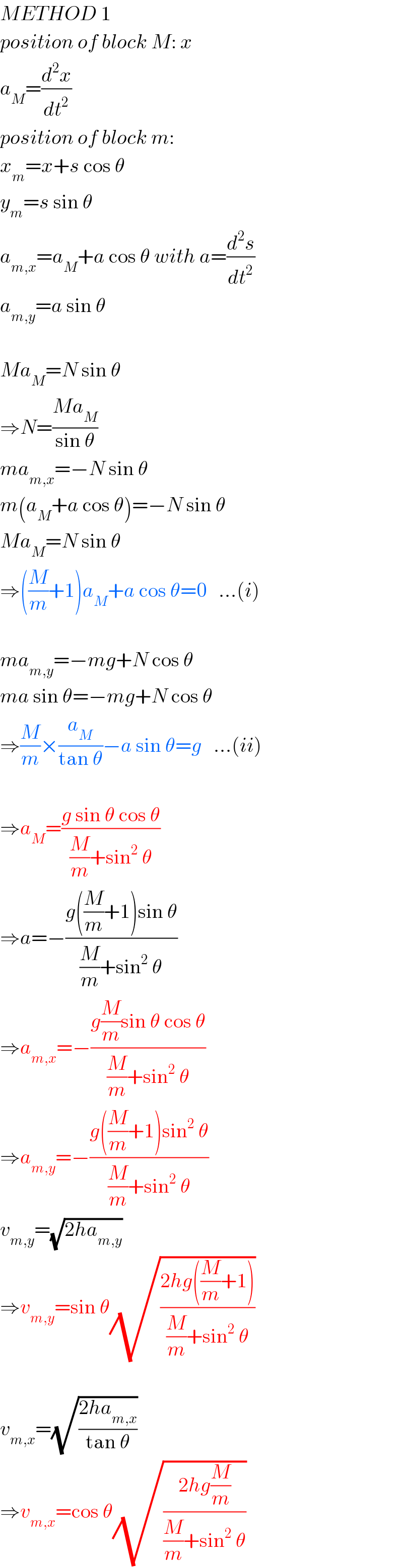
METHOD 1 position of block M: x a_M =(d^2 x/dt^2 ) position of block m: x_m =x+s cos θ y_m =s sin θ a_(m,x) =a_M +a cos θ with a=(d^2 s/dt^2 ) a_(m,y) =a sin θ Ma_M =N sin θ ⇒N=((Ma_M )/(sin θ)) ma_(m,x) =−N sin θ m(a_M +a cos θ)=−N sin θ Ma_M =N sin θ ⇒((M/m)+1)a_M +a cos θ=0 ...(i) ma_(m,y) =−mg+N cos θ ma sin θ=−mg+N cos θ ⇒(M/m)×(a_M /(tan θ))−a sin θ=g ...(ii) ⇒a_M =((g sin θ cos θ)/((M/m)+sin^2 θ)) ⇒a=−((g((M/m)+1)sin θ)/((M/m)+sin^2 θ)) ⇒a_(m,x) =−((g(M/m)sin θ cos θ)/((M/m)+sin^2 θ)) ⇒a_(m,y) =−((g((M/m)+1)sin^2 θ)/((M/m)+sin^2 θ)) v_(m,y) =(√(2ha_(m,y) )) ⇒v_(m,y) =sin θ(√((2hg((M/m)+1))/((M/m)+sin^2 θ))) v_(m,x) =(√((2ha_(m,x) )/(tan θ))) ⇒v_(m,x) =cos θ(√((2hg(M/m))/((M/m)+sin^2 θ)))

$${METHOD}\:\mathrm{1} \\ $$$${position}\:{of}\:{block}\:{M}:\:{x} \\ $$$${a}_{{M}} =\frac{{d}^{\mathrm{2}} {x}}{{dt}^{\mathrm{2}} } \\ $$$${position}\:{of}\:{block}\:{m}: \\ $$$${x}_{{m}} ={x}+{s}\:\mathrm{cos}\:\theta \\ $$$${y}_{{m}} ={s}\:\mathrm{sin}\:\theta \\ $$$${a}_{{m},{x}} ={a}_{{M}} +{a}\:\mathrm{cos}\:\theta\:{with}\:{a}=\frac{{d}^{\mathrm{2}} {s}}{{dt}^{\mathrm{2}} } \\ $$$${a}_{{m},{y}} ={a}\:\mathrm{sin}\:\theta \\ $$$$ \\ $$$${Ma}_{{M}} ={N}\:\mathrm{sin}\:\theta \\ $$$$\Rightarrow{N}=\frac{{Ma}_{{M}} }{\mathrm{sin}\:\theta} \\ $$$${ma}_{{m},{x}} =−{N}\:\mathrm{sin}\:\theta \\ $$$${m}\left({a}_{{M}} +{a}\:\mathrm{cos}\:\theta\right)=−{N}\:\mathrm{sin}\:\theta \\ $$$${Ma}_{{M}} ={N}\:\mathrm{sin}\:\theta \\ $$$$\Rightarrow\left(\frac{{M}}{{m}}+\mathrm{1}\right){a}_{{M}} +{a}\:\mathrm{cos}\:\theta=\mathrm{0}\:\:\:...\left({i}\right) \\ $$$$ \\ $$$${ma}_{{m},{y}} =−{mg}+{N}\:\mathrm{cos}\:\theta \\ $$$${ma}\:\mathrm{sin}\:\theta=−{mg}+{N}\:\mathrm{cos}\:\theta \\ $$$$\Rightarrow\frac{{M}}{{m}}×\frac{{a}_{{M}} }{\mathrm{tan}\:\theta}−{a}\:\mathrm{sin}\:\theta={g}\:\:\:...\left({ii}\right) \\ $$$$ \\ $$$$\Rightarrow{a}_{{M}} =\frac{{g}\:\mathrm{sin}\:\theta\:\mathrm{cos}\:\theta}{\frac{{M}}{{m}}+\mathrm{sin}^{\mathrm{2}} \:\theta} \\ $$$$\Rightarrow{a}=−\frac{{g}\left(\frac{{M}}{{m}}+\mathrm{1}\right)\mathrm{sin}\:\theta}{\frac{{M}}{{m}}+\mathrm{sin}^{\mathrm{2}} \:\theta} \\ $$$$\Rightarrow{a}_{{m},{x}} =−\frac{{g}\frac{{M}}{{m}}\mathrm{sin}\:\theta\:\mathrm{cos}\:\theta}{\frac{{M}}{{m}}+\mathrm{sin}^{\mathrm{2}} \:\theta} \\ $$$$\Rightarrow{a}_{{m},{y}} =−\frac{{g}\left(\frac{{M}}{{m}}+\mathrm{1}\right)\mathrm{sin}^{\mathrm{2}} \:\theta}{\frac{{M}}{{m}}+\mathrm{sin}^{\mathrm{2}} \:\theta} \\ $$$${v}_{{m},{y}} =\sqrt{\mathrm{2}{ha}_{{m},{y}} } \\ $$$$\Rightarrow{v}_{{m},{y}} =\mathrm{sin}\:\theta\sqrt{\frac{\mathrm{2}{hg}\left(\frac{{M}}{{m}}+\mathrm{1}\right)}{\frac{{M}}{{m}}+\mathrm{sin}^{\mathrm{2}} \:\theta}} \\ $$$$ \\ $$$${v}_{{m},{x}} =\sqrt{\frac{\mathrm{2}{ha}_{{m},{x}} }{\mathrm{tan}\:\theta}} \\ $$$$\Rightarrow{v}_{{m},{x}} =\mathrm{cos}\:\theta\sqrt{\frac{\mathrm{2}{hg}\frac{{M}}{{m}}}{\frac{{M}}{{m}}+\mathrm{sin}^{\mathrm{2}} \:\theta}} \\ $$

Commented by Tony Lin last updated on 10/Sep/19

thanks sir

$${thanks}\:{sir} \\ $$

Answered by mr W last updated on 09/Sep/19

Commented by mr W last updated on 09/Sep/19

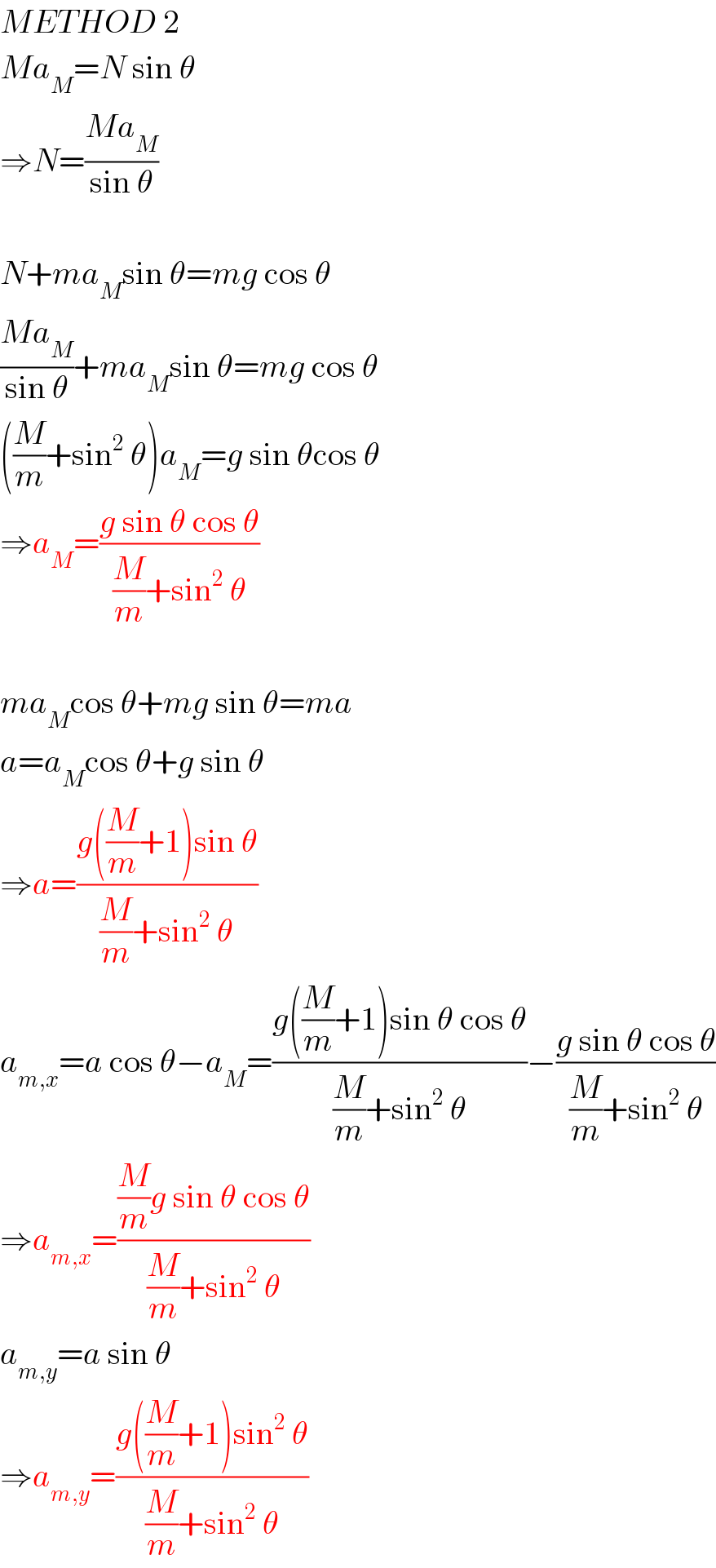
METHOD 2 Ma_M =N sin θ ⇒N=((Ma_M )/(sin θ)) N+ma_M sin θ=mg cos θ ((Ma_M )/(sin θ))+ma_M sin θ=mg cos θ ((M/m)+sin^2 θ)a_M =g sin θcos θ ⇒a_M =((g sin θ cos θ)/((M/m)+sin^2 θ)) ma_M cos θ+mg sin θ=ma a=a_M cos θ+g sin θ ⇒a=((g((M/m)+1)sin θ)/((M/m)+sin^2 θ)) a_(m,x) =a cos θ−a_M =((g((M/m)+1)sin θ cos θ)/((M/m)+sin^2 θ))−((g sin θ cos θ)/((M/m)+sin^2 θ)) ⇒a_(m,x) =(((M/m)g sin θ cos θ)/((M/m)+sin^2 θ)) a_(m,y) =a sin θ ⇒a_(m,y) =((g((M/m)+1)sin^2 θ)/((M/m)+sin^2 θ))

$${METHOD}\:\mathrm{2} \\ $$$${Ma}_{{M}} ={N}\:\mathrm{sin}\:\theta \\ $$$$\Rightarrow{N}=\frac{{Ma}_{{M}} }{\mathrm{sin}\:\theta} \\ $$$$ \\ $$$${N}+{ma}_{{M}} \mathrm{sin}\:\theta={mg}\:\mathrm{cos}\:\theta \\ $$$$\frac{{Ma}_{{M}} }{\mathrm{sin}\:\theta}+{ma}_{{M}} \mathrm{sin}\:\theta={mg}\:\mathrm{cos}\:\theta \\ $$$$\left(\frac{{M}}{{m}}+\mathrm{sin}^{\mathrm{2}} \:\theta\right){a}_{{M}} ={g}\:\mathrm{sin}\:\theta\mathrm{cos}\:\theta \\ $$$$\Rightarrow{a}_{{M}} =\frac{{g}\:\mathrm{sin}\:\theta\:\mathrm{cos}\:\theta}{\frac{{M}}{{m}}+\mathrm{sin}^{\mathrm{2}} \:\theta} \\ $$$$ \\ $$$${ma}_{{M}} \mathrm{cos}\:\theta+{mg}\:\mathrm{sin}\:\theta={ma} \\ $$$${a}={a}_{{M}} \mathrm{cos}\:\theta+{g}\:\mathrm{sin}\:\theta \\ $$$$\Rightarrow{a}=\frac{{g}\left(\frac{{M}}{{m}}+\mathrm{1}\right)\mathrm{sin}\:\theta}{\frac{{M}}{{m}}+\mathrm{sin}^{\mathrm{2}} \:\theta} \\ $$$${a}_{{m},{x}} ={a}\:\mathrm{cos}\:\theta−{a}_{{M}} =\frac{{g}\left(\frac{{M}}{{m}}+\mathrm{1}\right)\mathrm{sin}\:\theta\:\mathrm{cos}\:\theta}{\frac{{M}}{{m}}+\mathrm{sin}^{\mathrm{2}} \:\theta}−\frac{{g}\:\mathrm{sin}\:\theta\:\mathrm{cos}\:\theta}{\frac{{M}}{{m}}+\mathrm{sin}^{\mathrm{2}} \:\theta} \\ $$$$\Rightarrow{a}_{{m},{x}} =\frac{\frac{{M}}{{m}}{g}\:\mathrm{sin}\:\theta\:\mathrm{cos}\:\theta}{\frac{{M}}{{m}}+\mathrm{sin}^{\mathrm{2}} \:\theta} \\ $$$${a}_{{m},{y}} ={a}\:\mathrm{sin}\:\theta \\ $$$$\Rightarrow{a}_{{m},{y}} =\frac{{g}\left(\frac{{M}}{{m}}+\mathrm{1}\right)\mathrm{sin}^{\mathrm{2}} \:\theta}{\frac{{M}}{{m}}+\mathrm{sin}^{\mathrm{2}} \:\theta} \\ $$

Commented by Tony Lin last updated on 10/Sep/19

thanks sir

$${thanks}\:{sir} \\ $$

Answered by Tanmay chaudhury last updated on 09/Sep/19

Commented by Tanmay chaudhury last updated on 09/Sep/19

Mg+Ncosθ=N_G MA=Nsinθ for m mgsinθ+mAcosθ=ma mgcosθ=N+mAsinθ MA=sinθ(mgcosθ−mAsinθ) A(M+msin^2 θ)=mgsinθcosθ A=((mgsinθcosθ)/(M+msin^2 θ)) A=acc of wedge a=gsinθ+(((mgsinθcosθ)/(M+msin^2 θ)))cosθ a=((Mgsinθ+mgsin^3 θ+mgsinθcos^2 θ)/(M+msin^2 θ)) a=((Mgsinθ+mgsinθ(sin^2 θ+cos^2 θ))/(M+msin^2 θ)) a=(((M+m)gsinθ)/(M+msin^2 θ))

$${Mg}+{Ncos}\theta={N}_{{G}} \\ $$$${MA}={Nsin}\theta \\ $$$${for}\:{m} \\ $$$${mgsin}\theta+{mAcos}\theta={ma} \\ $$$${mgcos}\theta={N}+{mAsin}\theta \\ $$$${MA}={sin}\theta\left({mgcos}\theta−{mAsin}\theta\right) \\ $$$${A}\left({M}+{msin}^{\mathrm{2}} \theta\right)={mgsin}\theta{cos}\theta \\ $$$${A}=\frac{{mgsin}\theta{cos}\theta}{{M}+{msin}^{\mathrm{2}} \theta} \\ $$$${A}={acc}\:{of}\:{wedge} \\ $$$${a}={gsin}\theta+\left(\frac{{mgsin}\theta{cos}\theta}{{M}+{msin}^{\mathrm{2}} \theta}\right){cos}\theta \\ $$$${a}=\frac{{Mgsin}\theta+{mgsin}^{\mathrm{3}} \theta+{mgsin}\theta{cos}^{\mathrm{2}} \theta}{{M}+{msin}^{\mathrm{2}} \theta} \\ $$$${a}=\frac{{Mgsin}\theta+{mgsin}\theta\left({sin}^{\mathrm{2}} \theta+{cos}^{\mathrm{2}} \theta\right)}{{M}+{msin}^{\mathrm{2}} \theta} \\ $$$${a}=\frac{\left({M}+{m}\right){gsin}\theta}{{M}+{msin}^{\mathrm{2}} \theta} \\ $$$$ \\ $$$$ \\ $$

Commented by Tony Lin last updated on 10/Sep/19

thanks sir

$${thanks}\:{sir} \\ $$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com