
Question Number 61318 by Tawa1 last updated on 31/May/19

Answered by tanmay last updated on 01/Jun/19
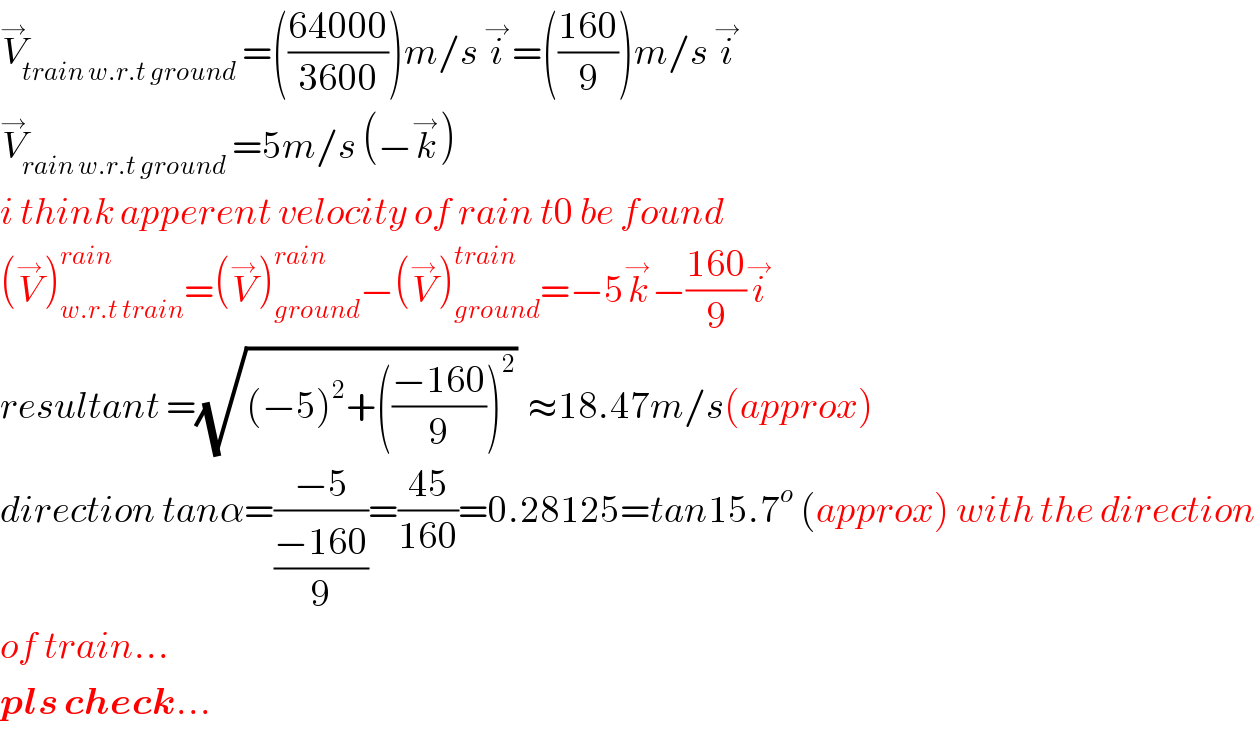
$$\overset{\rightarrow} {{V}}_{{train}\:{w}.{r}.{t}\:{ground}} =\left(\frac{\mathrm{64000}}{\mathrm{3600}}\right){m}/{s}\:\overset{\rightarrow} {{i}}=\left(\frac{\mathrm{160}}{\mathrm{9}}\right){m}/{s}\:\overset{\rightarrow} {{i}} \\ $$$$\overset{\rightarrow} {{V}}_{{rain}\:{w}.{r}.{t}\:{ground}} =\mathrm{5}{m}/{s}\:\left(−\overset{\rightarrow} {{k}}\right) \\ $$$${i}\:{think}\:{apperent}\:{velocity}\:{of}\:{rain}\:{t}\mathrm{0}\:{be}\:{found} \\ $$$$\left(\overset{\rightarrow} {{V}}\right)_{{w}.{r}.{t}\:{train}} ^{{rain}} =\left(\overset{\rightarrow} {{V}}\right)_{{ground}} ^{{rain}} −\left(\overset{\rightarrow} {{V}}\right)_{{ground}} ^{{train}} =−\mathrm{5}\overset{\rightarrow} {{k}}−\frac{\mathrm{160}}{\mathrm{9}}\overset{\rightarrow} {{i}} \\ $$$${resultant}\:=\sqrt{\left(−\mathrm{5}\right)^{\mathrm{2}} +\left(\frac{−\mathrm{160}}{\mathrm{9}}\right)^{\mathrm{2}} }\:\:\approx\mathrm{18}.\mathrm{47}{m}/{s}\left({approx}\right) \\ $$$${direction}\:{tan}\alpha=\frac{−\mathrm{5}}{\frac{−\mathrm{160}}{\mathrm{9}}}=\frac{\mathrm{45}}{\mathrm{160}}=\mathrm{0}.\mathrm{28125}={tan}\mathrm{15}.\mathrm{7}^{{o}} \:\left({approx}\right)\:{with}\:{the}\:{direction} \\ $$$${of}\:{train}... \\ $$$$\boldsymbol{{pls}}\:\boldsymbol{{check}}... \\ $$
Commented by Tawa1 last updated on 01/Jun/19
$$\mathrm{God}\:\mathrm{bless}\:\mathrm{you}\:\mathrm{sir} \\ $$
