
Question Number 59509 by aliesam last updated on 11/May/19

Answered by MJS last updated on 11/May/19
![∫csc πx dx=∫(dx/(sin πx))= [t=πx → dx=(dt/π)] =(1/π)∫(dt/(sin t))= [u=tan (t/2) → dt=2(du/(u^2 +1))] =(1/π)∫(du/u)=(1/π)ln u =(1/π)ln tan (t/2) = =(1/π)ln tan (π/2)x +C ∫_(1/6) ^(1/3) csc πx dx=(1/(2π))ln ((7+4(√3))/3) ≈.244351](Q59522.png)
$$\int\mathrm{csc}\:\pi{x}\:{dx}=\int\frac{{dx}}{\mathrm{sin}\:\pi{x}}= \\ $$$$\:\:\:\:\:\left[{t}=\pi{x}\:\rightarrow\:{dx}=\frac{{dt}}{\pi}\right] \\ $$$$=\frac{\mathrm{1}}{\pi}\int\frac{{dt}}{\mathrm{sin}\:{t}}= \\ $$$$\:\:\:\:\:\left[{u}=\mathrm{tan}\:\frac{{t}}{\mathrm{2}}\:\rightarrow\:{dt}=\mathrm{2}\frac{{du}}{{u}^{\mathrm{2}} +\mathrm{1}}\right] \\ $$$$=\frac{\mathrm{1}}{\pi}\int\frac{{du}}{{u}}=\frac{\mathrm{1}}{\pi}\mathrm{ln}\:{u}\:=\frac{\mathrm{1}}{\pi}\mathrm{ln}\:\mathrm{tan}\:\frac{{t}}{\mathrm{2}}\:= \\ $$$$=\frac{\mathrm{1}}{\pi}\mathrm{ln}\:\mathrm{tan}\:\frac{\pi}{\mathrm{2}}{x}\:+{C} \\ $$$$\underset{\mathrm{1}/\mathrm{6}} {\overset{\mathrm{1}/\mathrm{3}} {\int}}\mathrm{csc}\:\pi{x}\:{dx}=\frac{\mathrm{1}}{\mathrm{2}\pi}\mathrm{ln}\:\frac{\mathrm{7}+\mathrm{4}\sqrt{\mathrm{3}}}{\mathrm{3}}\:\approx.\mathrm{244351} \\ $$
Answered by aliesam last updated on 11/May/19
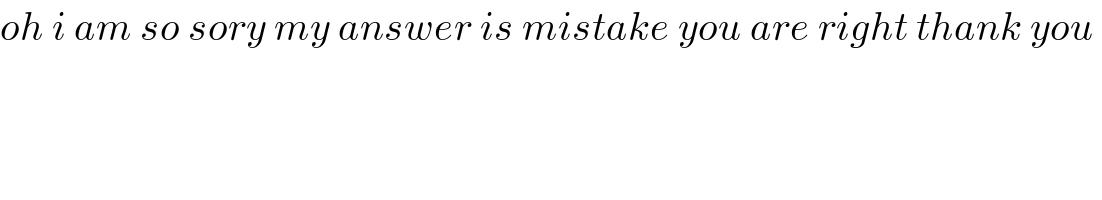
$${oh}\:{i}\:{am}\:{so}\:{sory}\:{my}\:{answer}\:{is}\:{mistake}\:{you}\:{are}\:{right}\:{thank}\:{you}\: \\ $$
Commented by MJS last updated on 11/May/19
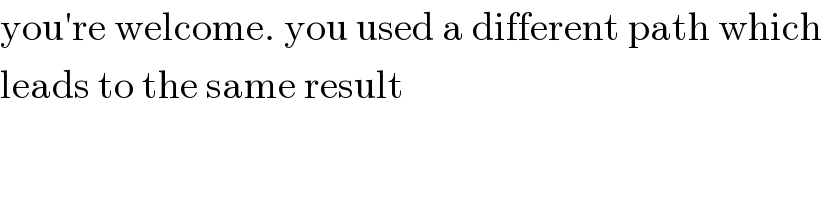
$$\mathrm{you}'\mathrm{re}\:\mathrm{welcome}.\:\mathrm{you}\:\mathrm{used}\:\mathrm{a}\:\mathrm{different}\:\mathrm{path}\:\mathrm{which} \\ $$$$\mathrm{leads}\:\mathrm{to}\:\mathrm{the}\:\mathrm{same}\:\mathrm{result} \\ $$
