
Question Number 56818 by Gulay last updated on 24/Mar/19
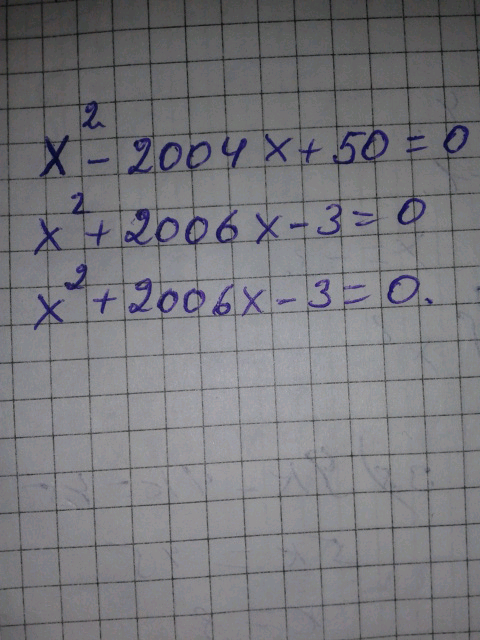
Commented by Gulay last updated on 24/Mar/19

$$\mathrm{sir}\:\mathrm{plz}\:\mathrm{help}\:\mathrm{me} \\ $$
Commented by tanmay.chaudhury50@gmail.com last updated on 24/Mar/19
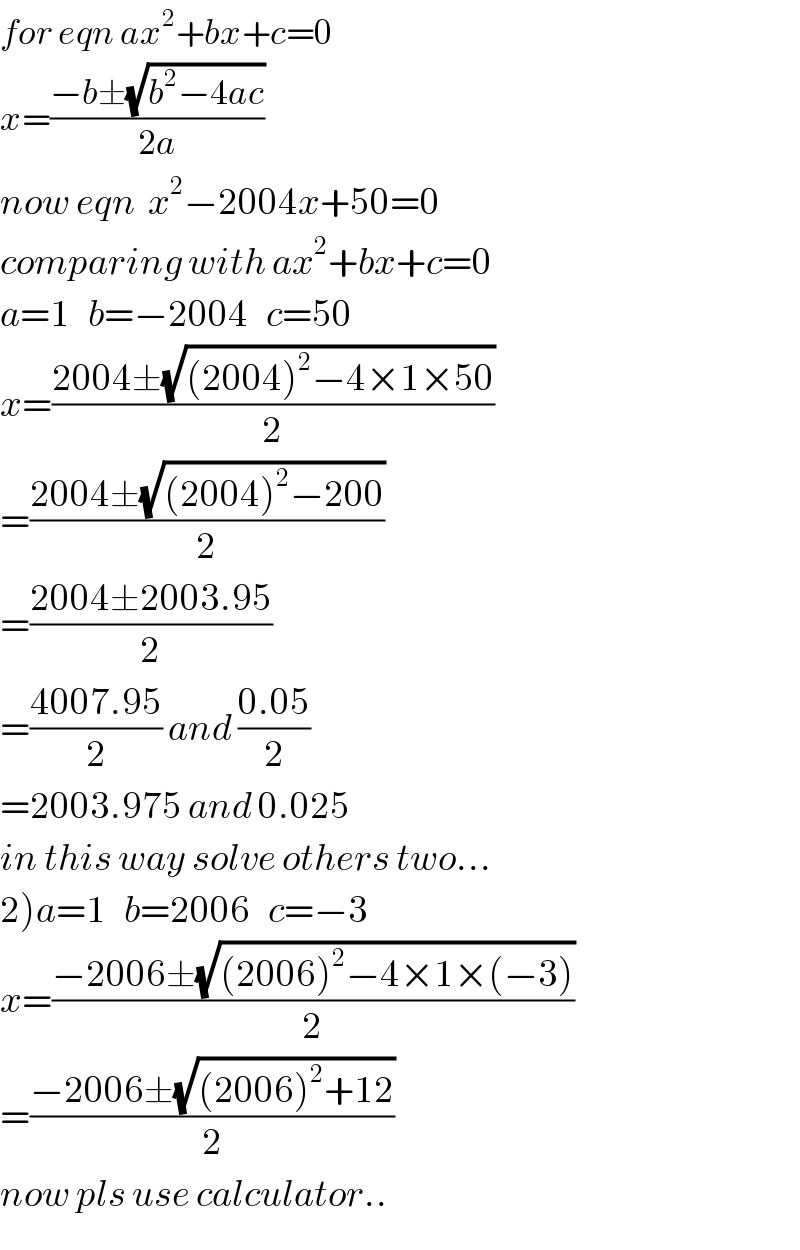
$${for}\:{eqn}\:{ax}^{\mathrm{2}} +{bx}+{c}=\mathrm{0} \\ $$$${x}=\frac{−{b}\pm\sqrt{{b}^{\mathrm{2}} −\mathrm{4}{ac}}}{\mathrm{2}{a}} \\ $$$${now}\:{eqn}\:\:{x}^{\mathrm{2}} −\mathrm{2004}{x}+\mathrm{50}=\mathrm{0}\:\: \\ $$$${comparing}\:{with}\:{ax}^{\mathrm{2}} +{bx}+{c}=\mathrm{0} \\ $$$${a}=\mathrm{1}\:\:\:{b}=−\mathrm{2004}\:\:\:{c}=\mathrm{50} \\ $$$${x}=\frac{\mathrm{2004}\pm\sqrt{\left(\mathrm{2004}\right)^{\mathrm{2}} −\mathrm{4}×\mathrm{1}×\mathrm{50}}}{\mathrm{2}} \\ $$$$=\frac{\mathrm{2004}\pm\sqrt{\left(\mathrm{2004}\right)^{\mathrm{2}} −\mathrm{200}}}{\mathrm{2}} \\ $$$$=\frac{\mathrm{2004}\pm\mathrm{2003}.\mathrm{95}}{\mathrm{2}} \\ $$$$=\frac{\mathrm{4007}.\mathrm{95}}{\mathrm{2}}\:{and}\:\frac{\mathrm{0}.\mathrm{05}}{\mathrm{2}} \\ $$$$=\mathrm{2003}.\mathrm{975}\:{and}\:\mathrm{0}.\mathrm{025} \\ $$$${in}\:{this}\:{way}\:{solve}\:{others}\:{two}... \\ $$$$\left.\mathrm{2}\right){a}=\mathrm{1}\:\:\:{b}=\mathrm{2006}\:\:\:{c}=−\mathrm{3} \\ $$$${x}=\frac{−\mathrm{2006}\pm\sqrt{\left(\mathrm{2006}\right)^{\mathrm{2}} −\mathrm{4}×\mathrm{1}×\left(−\mathrm{3}\right)}}{\mathrm{2}} \\ $$$$=\frac{−\mathrm{2006}\pm\sqrt{\left(\mathrm{2006}\right)^{\mathrm{2}} +\mathrm{12}}}{\mathrm{2}} \\ $$$${now}\:{pls}\:{use}\:{calculator}.. \\ $$
